Advertisements
Advertisements
प्रश्न
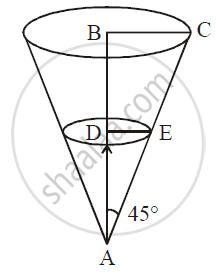
Water is dripping out from a conical funnel of semi-verticle angle `pi/4` at the uniform rate of `2 cm^2/sec`in the surface, through a tiny hole at the vertex of the bottom. When the slant height of the water level is 4 cm, find the rate of decrease of the slant height of the water.
उत्तर
Let r be the radius, h be the height and V be the volume of the funnel at any time t
`V = 1/3 pir^2h` ... (i)
Let I be the slant height of the funnel
Given: Semi-vertical angle = 45° in the triangle ADE:
`sin 45^@ = (DE)/(AE) => 1/sqrt2 = r/l`
`cos 45^@ = (AD)/(AE) => 1/sqrt2 = h/l`
`r = 1/sqrt2` and h = `1/sqrt2` ...(ii)
therefore the equation (i) can be rewritten as :
`V = 1/3 pi xx (I/sqrt2)^2 xx I/sqrt2 = pi/(3xx2xxsqrt2) xx I^3``
`V = pi/(6sqrt2) I^3` ...(iii)
Differentiate w.r.t. t :
`(dV)/(dt) = pi/(6sqrt2) xx 3l^2 xx (dl)/(dt)`
`(dv)/(dt) = pi/(2sqrt2) xx l^2 xx (dl)/(dt)`
`(dl)/(dt) = (2sqrt2)/(pil^2) xx (dV)/(dt)` ...(iv)
Since it is given that rate of change (decrease) of volume of water w.r.t. t is
`(dV)/(dt) = -2cm^3"/"sec`
therefore
`(dl)/(dt) = (2sqrt2)/(lambdal^2) xx (-2) = -(4sqrt2)/(lambdal^2)`
`(dl)/(dt)|_"at l = 4" = - (4sqrt2)/(pixx(4)^2) = - (sqrt2)/(4pi) "cm/sec"`
APPEARS IN
संबंधित प्रश्न
The amount of pollution content added in air in a city due to x-diesel vehicles is given by P(x) = 0.005x3 + 0.02x2 + 30x. Find the marginal increase in pollution content when 3 diesel vehicles are added and write which value is indicated in the above question.
Prove that the function f given by f(x) = log sin x is strictly increasing on `(0, pi/2)` and strictly decreasing on `(pi/2, pi)`
Prove that the function f(x) = loge x is increasing on (0, ∞) ?
Prove that the function f(x) = loga x is increasing on (0, ∞) if a > 1 and decreasing on (0, ∞), if 0 < a < 1 ?
Prove that f(x) = ax + b, where a, b are constants and a < 0 is a decreasing function on R ?
Find the interval in which the following function are increasing or decreasing f(x) = x2 + 2x − 5 ?
Find the interval in which the following function are increasing or decreasing \[f\left( x \right) = 3 x^4 - 4 x^3 - 12 x^2 + 5\] ?
Determine whether f(x) = −x/2 + sin x is increasing or decreasing on (−π/3, π/3) ?
Show that f(x) = x2 − x sin x is an increasing function on (0, π/2) ?
Write the set of values of 'a' for which f(x) = loga x is increasing in its domain ?
Let f(x) = x3 + ax2 + bx + 5 sin2x be an increasing function on the set R. Then, a and b satisfy.
The function \[f\left( x \right) = \log_e \left( x^3 + \sqrt{x^6 + 1} \right)\] is of the following types:
Function f(x) = cos x − 2 λ x is monotonic decreasing when
f(x) = 2x − tan−1 x − log \[\left\{ x + \sqrt{x^2 + 1} \right\}\] is monotonically increasing when
Find the intervals in which the function `f("x") = (4sin"x")/(2+cos"x") -"x";0≤"x"≤2pi` is strictly increasing or strictly decreasing.
Find the values of x for which the function f(x) = x3 – 12x2 – 144x + 13 (a) increasing (b) decreasing
Find the values of x for which f(x) = `x/(x^2 + 1)` is (a) strictly increasing (b) decreasing.
Show that y = `log (1 + x) – (2x)/(2 + x), x > - 1` is an increasing function on its domain.
Show that function f(x) =`("x - 2")/("x + 1")`, x ≠ -1 is increasing.
Find the values of x, for which the function f(x) = x3 + 12x2 + 36𝑥 + 6 is monotonically decreasing
The price P for the demand D is given as P = 183 + 120D − 3D2, then the value of D for which price is increasing, is ______.
A man of height 1.9 m walks directly away from a lamp of height 4.75m on a level road at 6m/s. The rate at which the length of his shadow is increasing is
Let the f : R → R be defined by f (x) = 2x + cosx, then f : ______.
The values of a for which the function f(x) = sinx – ax + b increases on R are ______.
The function f(x) = x2 – 2x is increasing in the interval ____________.
The function f(x) = `(4x^3 - 3x^2)/6 - 2sinx + (2x - 1)cosx` ______.
Function f(x) = `log(1 + x) - (2x)/(2 + x)` is monotonically increasing when ______.
A function f is said to be increasing at a point c if ______.
Read the following passage:
|
The use of electric vehicles will curb air pollution in the long run. V(t) = `1/5 t^3 - 5/2 t^2 + 25t - 2` where t represents the time and t = 1, 2, 3, ...... corresponds to years 2001, 2002, 2003, ...... respectively. |
Based on the above information, answer the following questions:
- Can the above function be used to estimate number of vehicles in the year 2000? Justify. (2)
- Prove that the function V(t) is an increasing function. (2)

