Advertisements
Advertisements
प्रश्न
Answer the following:
If `log ((x - y)/5) = 1/2 logx + 1/2 log y`, show that x2 + y2 = 27xy
उत्तर
`log ((x - y)/5) = 1/2 logx + 1/2 log y`
Multiplying throughout by 2, we get
`2log ((x - y)/5)` = log x + log y
∴ `log((x - y)/5)^2` = log xy
∴ `(x - y)^2/25` = xy
∴ x2 – 2xy + y2 = 25xy
∴ x2 + y2 = 27xy
APPEARS IN
संबंधित प्रश्न
What is the fundamental difference between a relation and a function? Is every relation a function?
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(b) f2 = {(1, 1), (2, 7), (3, 5)}
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(v) \[\frac{g}{f}\]
Let f(x) = x2 and g(x) = 2x+ 1 be two real functions. Find (f + g) (x), (f − g) (x), (fg) (x) and \[\left( \frac{f}{g} \right) \left( x \right)\] .
Write the range of the function f(x) = ex−[x], x ∈ R.
Let A = {1, 2, 3} and B = {2, 3, 4}. Then which of the following is a function from A to B?
If \[e^{f\left( x \right)} = \frac{10 + x}{10 - x}\] , x ∈ (−10, 10) and \[f\left( x \right) = kf\left( \frac{200 x}{100 + x^2} \right)\] , then k =
If \[3f\left( x \right) + 5f\left( \frac{1}{x} \right) = \frac{1}{x} - 3\] for all non-zero x, then f(x) =
If f(m) = m2 − 3m + 1, find f(− x)
Check if the following relation is a function.
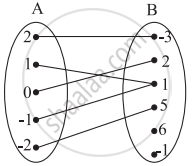
If f(m) = m2 − 3m + 1, find `f(1/2)`
Find x, if g(x) = 0 where g(x) = `(18 -2x^2)/7`
Express the area A of circle as a function of its diameter d
Check the injectivity and surjectivity of the following function.
f : R → R given by f(x) = x3
Express the following logarithmic equation in exponential form
ln 1 = 0
Write the following expression as a single logarithm.
`1/3 log (x - 1) + 1/2 log (x)`
Given that log 2 = a and log 3 = b, write `log sqrt(96)` in terms of a and b
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
If f(x) = 3x + 5, g(x) = 6x − 1, then find (fg) (3)
Select the correct answer from given alternatives.
Let the function f be defined by f(x) = `(2x + 1)/(1 - 3x)` then f–1 (x) is ______.
Answer the following:
Let f: R → R be a function defined by f(x) = 5x3 – 8 for all x ∈ R, show that f is one-one and onto. Hence find f –1
Answer the following:
A function f is defined as f(x) = 4x + 5, for – 4 ≤ x < 0. Find the values of f(–1), f(–2), f(0), if they exist
Answer the following:
Let f : R → R be given by f(x) = x3 + 1 for all x ∈ R. Draw its graph
Answer the following:
Show that, `log |sqrt(x^2 + 1) + x | + log | sqrt(x^2 + 1) - x|` = 0
Answer the following:
If b2 = ac. prove that, log a + log c = 2 log b
Answer the following:
Find value of `(3 + log_10 343)/(2 + 1/2 log_10 (49/4) + 1/2 log_10 (1/25)`
Answer the following:
Show that, logy x3 . logz y4 . logx z5 = 60
Answer the following:
If `log_2"a"/4 = log_2"b"/6 = log_2"c"/(3"k")` and a3b2c = 1 find the value of k
Find the domain of the following function.
f(x) = `sqrtlog(x^2 - 6x + 6)`
Answer the following:
Find the range of the following function.
f(x) = 1 + 2x + 4x
A graph representing the function f(x) is given in it is clear that f(9) = 2

What is the image of 6 under f?
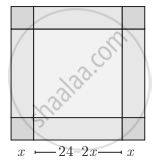
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
The range of the function f(x) = `(x^2 - 3x + 2)/(x^3 - 4x^2 + 5x - 2)` is ______
If a function f(x) is given as f(x) = x2 – 6x + 4 for all x ∈ R, then f(–3) = ______.
Let f : R → R be defined by
f(x) = `{(3x; x > 2),(2x^2; 1 ≤ x ≤ 2), (4x; x < 1):}`
Then f(-2) + f(1) + f(3) is ______
Redefine the function f(x) = x − 2 + 2 + x , – 3 ≤ x ≤ 3
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f + g)(x)
Let f be a function with domain [–3, 5] and let g(x) = | 3x + 4 |. Then, the domain of (fog) (x) is ______.
