Advertisements
Advertisements
प्रश्न
Find the range of the following functions given by f(x) = 1 – |x – 2|
उत्तर
For real value of f
|x – 2| ≥ 0
Adding negative sign, we get
Or –|x – 2| ≤ 0
Adding 1 we get
⇒ 1 – |x – 2| ≤ 1
Or f(x) ≤ 1
⇒ f(x) ∈ `(–oo, 1]`
Therefore, the range of f = `(–oo, 1]`
APPEARS IN
संबंधित प्रश्न
Define a function as a correspondence between two sets.
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
et A = (12, 13, 14, 15, 16, 17) and f : A → Z be a function given by
f(x) = highest prime factor of x.
Find range of f.
If \[f\left( x \right) = \frac{1}{1 - x}\] , show that f[f[f(x)]] = x.
Let \[f\left( x \right) = \frac{\alpha x}{x + 1}, x \neq - 1\] . Then write the value of α satisfying f(f(x)) = x for all x ≠ −1.
Write the domain and range of the function \[f\left( x \right) = \frac{x - 2}{2 - x}\] .
Write the domain and range of function f(x) given by
If f(x) = cos (loge x), then \[f\left( \frac{1}{x} \right)f\left( \frac{1}{y} \right) - \frac{1}{2}\left\{ f\left( xy \right) + f\left( \frac{x}{y} \right) \right\}\] is equal to
If f(x) = sin [π2] x + sin [−π]2 x, where [x] denotes the greatest integer less than or equal to x, then
The domain of definition of \[f\left( x \right) = \sqrt{4x - x^2}\] is
The domain of definition of \[f\left( x \right) = \sqrt{x - 3 - 2\sqrt{x - 4}} - \sqrt{x - 3 + 2\sqrt{x - 4}}\] is
Which of the following relations are functions? If it is a function determine its domain and range:
{(0, 0), (1, 1), (1, −1), (4, 2), (4, −2), (9, 3), (9, −3), (16, 4), (16, −4)}
Check if the following relation is a function.
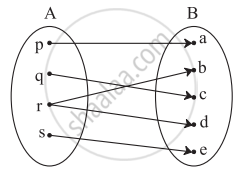
Check if the relation given by the equation represents y as function of x:
x + y2 = 9
Check if the relation given by the equation represents y as function of x:
x2 − y = 25
Find x, if g(x) = 0 where g(x) = x3 − 2x2 − 5x + 6
lf f(x) = 3(4x+1), find f(– 3)
Write the following expression as a single logarithm.
5 log x + 7 log y − log z
If f(x) = ax2 − bx + 6 and f(2) = 3 and f(4) = 30, find a and b
Select the correct answer from given alternatives
The domain of `1/([x] - x)` where [x] is greatest integer function is
Answer the following:
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b
Find the range of the following functions given by `|x - 4|/(x - 4)`
Find the range of the following functions given by `sqrt(16 - x^2)`
If f(x) = `x^3 - 1/x^3`, then `f(x) + f(1/x)` is equal to ______.
Find the range of the following functions given by f(x) = 1 + 3 cos2x
(Hint: –1 ≤ cos 2x ≤ 1 ⇒ –3 ≤ 3 cos 2x ≤ 3 ⇒ –2 ≤ 1 + 3cos 2x ≤ 4)
The domain and range of the function f given by f(x) = 2 – |x – 5| is ______.
If f : R – {2} `rightarrow` R i s a function defined by f(x) = `(x^2 - 4)/(x - 2)`, then its range is ______.
The period of the function
f(x) = `(sin 8x cos x - sin 6x cos 3x)/(cos 2x cos x - sin 3x sin 4x)` is ______.
