Advertisements
Advertisements
Question
If sec 2A = cosec (A – 42°) where 2A is an acute angle. Find the value of A.
Solution
We know that (sec (90 – θ)) = cosec θ
Sec 2A = sec (90 – (A – 42))
Sec 2A = sec (90 – A + 42)
Sec 2A = sec (132 – A)
Now equating both the angles we get
2A = 132 – A
`3A = 132/3`
A= 44
APPEARS IN
RELATED QUESTIONS
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
if `sec A = 5/4` verify that `(3 sin A - 4 sin^3 A)/(4 cos^3 A - 3 cos A) = (3 tan A - tan^3 A)/(1- 3 tan^2 A)`
If A = B = 60°, verify that sin (A − B) = sin A cos B − cos A sin B
If x = cosec A +cos A and y = cosec A – cos A then prove that `(2/(x+y))^2 + ((x-y)/2)^2` = 1
Using the formula, sin A = `sqrt((1-cos 2A)/2) ` find the value of sin 300, it being given that cos 600 = `1/2`
If 3x = cosecθ = and `3/x= cottheta` find the value of 3`(x^2-1/x^2)`.
`(cos 28°)/(sin 62°)` = ?
In triangle ABC, AB = AC = 15 cm and BC = 18 cm, find cos ∠ABC.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: sin P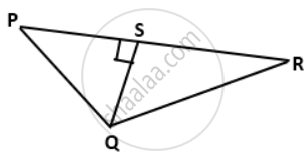
If cosec θ = `(29)/(20)`, find the value of: cosec θ - `(1)/("cot" θ)`
