Advertisements
Advertisements
Question
In the given figure, AM ⊥ BC and AN is the bisector of ∠A. If ∠B = 65° and ∠C = 33°, find ∠MAN.
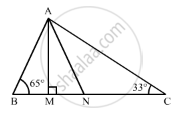
Solution
In the given ΔABC, AM⊥BC, ANis the bisector of ∠A, ∠B = 65 and ∠C = 33
We need to find ∠MAN
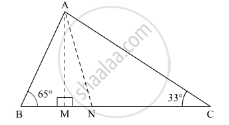
Now, using the angle sum property of the triangle
In ΔAMC, we get,
∠MAC + ∠AMC + ∠ACM = 180°
∠MAC + 90° + 33° = 180°
∠MAC + 123° = 180
∠MAC= 180° - 123°
∠MAC = 57°…….(1)
Similarly,
In ΔABM, we get,
∠ABM + ∠AMB+ ∠BAM = 180°
∠BAM + 90°+ 65° = 180°
∠AM + 155° = 180°
∠BAM = 180° - 155°
∠BAM …..(2)
So, adding (1) and (2)
∠BAM + ∠MAC = 25° + 57°
∠BAM + ∠MAC = 82°
Now, since AN is the bisector of ∠A
∠BAN = ∠NAC
Thus,
∠BAN + ∠NAC = 82°
2∠BAN =82°
` ∠BAN = (82°)/2`
= 41
Now,
∠MAN = ∠BAN - ∠BAM
= 41 - 25
= 16
Therefore, ∠MAN = 16.
APPEARS IN
RELATED QUESTIONS
If the bisectors of the base angles of a triangle enclose an angle of 135°, prove that the triangle is a right triangle.
Can a triangle have All angles more than 60°? Justify your answer in case.
AB is a line segment. P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (See Fig. 10.26). Show that the line PQ is perpendicular bisector of AB.

The sum of two angles of a triangle is equal to its third angle. Determine the measure of the third angle.
In a triangle, an exterior angle at a vertex is 95° and its one of the interior opposite angle is 55°, then the measure of the other interior angle is
State, if the triangle is possible with the following angles :
125°, 40°, and 15°
Calculate the unknown marked angles of the following figure :

The length of the sides of the triangle is given. Say what types of triangles they are 3.4 cm, 3.4 cm, 5 cm.
The angles of the triangle are 3x – 40, x + 20 and 2x – 10 then the value of x is
If an angle of a triangle is equal to the sum of the other two angles, find the type of the triangle
