Advertisements
Advertisements
Question
The interval in which the function f(x) = 2x3 + 9x2 + 12x – 1 is decreasing is ______.
Options
(– 1, ∞)
(– 2, – 1)
(– ∞, – 2)
[– 1, 1]
Solution
The interval in which the function f(x) = 2x3 + 9x2 + 12x – 1 is decreasing is (– 2, – 1).
Explanation:
Given,
f(x) = 2x3 + 9x2 + 12x – 1
f'(x) = 6x2 + 18x + 12 = 6(x2 + 3x + 2)
For increasing or decreasing, f'(x) = a
x2 + 3x + 2 = 0
`\implies` x = – 1, – 2
Sign scheme indicates
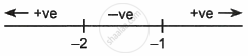
So the function is decreasing in (– 2, – 1).
APPEARS IN
RELATED QUESTIONS
Find the intervals in which the function f(x) = 3x4 − 4x3 − 12x2 + 5 is
(a) strictly increasing
(b) strictly decreasing
Find the intervals in which f(x) = sin 3x – cos 3x, 0 < x < π, is strictly increasing or strictly decreasing.
Find the value of c in Rolle's theorem for the function `f(x) = x^3 - 3x " in " (-sqrt3, 0)`
Water is dripping out from a conical funnel of semi-verticle angle `pi/4` at the uniform rate of `2 cm^2/sec`in the surface, through a tiny hole at the vertex of the bottom. When the slant height of the water level is 4 cm, find the rate of decrease of the slant height of the water.
Find the interval in which the following function are increasing or decreasing f(x) = 5x3 − 15x2 − 120x + 3 ?
Show that f(x) = cos x is a decreasing function on (0, π), increasing in (−π, 0) and neither increasing nor decreasing in (−π, π) ?
Prove that the function f given by f(x) = log cos x is strictly increasing on (−π/2, 0) and strictly decreasing on (0, π/2) ?
Write the set of values of 'a' for which f(x) = loga x is decreasing in its domain ?
Find the set of values of 'a' for which f(x) = x + cos x + ax + b is increasing on R ?
If g (x) is a decreasing function on R and f(x) = tan−1 [g (x)]. State whether f(x) is increasing or decreasing on R ?
Write the set of values of a for which f(x) = cos x + a2 x + b is strictly increasing on R ?
In the interval (1, 2), function f(x) = 2 | x − 1 | + 3 | x − 2 | is
The price P for demand D is given as P = 183 + 120 D – 3D2.
Find D for which the price is increasing.
Find the intervals in which function f given by f(x) = 4x3 - 6x2 - 72x + 30 is (a) strictly increasing, (b) strictly decresing .
Prove that function f(x) = `x - 1/x`, x ∈ R and x ≠ 0 is increasing function
Find the values of x for which the function f(x) = x3 – 6x2 – 36x + 7 is strictly increasing
Choose the correct alternative:
The function f(x) = x3 – 3x2 + 3x – 100, x ∈ R is
The total cost function for production of articles is given as C = 100 + 600x – 3x2, then the values of x for which the total cost is decreasing is ______
Show that the function f(x) = `(x - 2)/(x + 1)`, x ≠ – 1 is increasing
The function f(x) = 9 - x5 - x7 is decreasing for
The function f(x) = sin x + 2x is ______
If f(x) = x3 – 15x2 + 84x – 17, then ______.
If f(x) = sin x – cos x, then interval in which function is decreasing in 0 ≤ x ≤ 2 π, is:
Let h(x) = f(x) - [f(x)]2 + [f(x)]3 for every real number x. Then ____________.
The interval in which `y = x^2e^(-x)` is increasing with respect to `x` is
Show that function f(x) = tan x is increasing in `(0, π/2)`.
If f(x) = x + cosx – a then ______.
Function f(x) = x100 + sinx – 1 is increasing for all x ∈ ______.
Find the interval in which the function f(x) = x2e–x is strictly increasing or decreasing.
