Advertisements
Advertisements
प्रश्न
Sketch the region bounded by the curves `y=sqrt(5-x^2)` and y=|x-1| and find its area using integration.
उत्तर
Consider the given equation
`y=sqrt(5-x^2)`
This equation represents a semicircle with centre at
the origin and radius = sqrt5 units
Given that the region is bounded by the above
semicircle and the line y = |x-1|
Let us find the point of intersection of the
given curve meets the line y= |x - 1|
`=>sqrt(5-x^2)=|x-1|`
Squaring both the sides, we have,
`5-x^2=|x-1|^2`
`=>5-x^2=x^2+1-2x`
`=>2x^2-2x-5+1=0`
`=>2x^2-2x-4=0`
`=>x^2-x-2=0`
`=>x^2-2x+x-2=0`
`=>x(x-2)+1(x-2)=0`
`=>(x+1)(x-2)=0`
`=>x=-1, x=2`
When x = -1,y = 2
When x = 2,y = 1
Consider the following figure.
Thus the intersection points are ( -1,2) and (2,1)
Consider the following sketch of the bounded region.
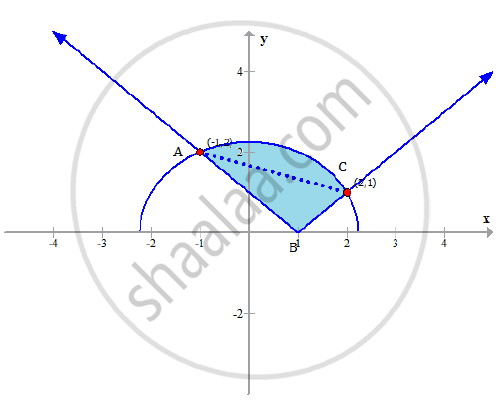
Required Area, A= `int_(-1)^2(y_2-y_1)dx`
=`=int_(-1)^1[sqrt(5-x^2)+(x-1)]dx+int_1^2[sqrt(5-x^2)-(x-1)]dx`
`=int_(-1)^1sqrt(5-x^2)dx+int_(-1)^1xdx-int_(-1)^1dx+int_1^2sqrt(5-x^2)dx-int_1^2xdx+int_1^2dx`
`=[x/2sqrt(5-x^2)+5/2sin^-1(x/sqrt5)]_(-1)^1+(x^2/2)_(-1)^1-(x)_(-1)^1+[x/2sqrt(5-x^2)+5/2sin^-1(x/sqrt5)]_1^2-(x^2/2)_(1)^2+(x)_(1)^2`
`=5/2 sin^-1 (1/sqrt5)+5/2 sin^-1 (2/sqrt5)-1/2`
Required area= `[5/2 sin^-1 (1/sqrt5)+5/2 sin^-1 (2/sqrt5)-1/2 ] sq.units`
APPEARS IN
संबंधित प्रश्न
Using the method of integration find the area of the region bounded by lines: 2x + y = 4, 3x – 2y = 6 and x – 3y + 5 = 0
Find the area of the region lying in the first quandrant bounded by the curve y2= 4x, X axis and the lines x = 1, x = 4
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5.
Using integration, find the area of the region bounded by the line 2y = 5x + 7, x-axis and the lines x = 2 and x = 8.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Sketch the graph y = | x + 1 |. Evaluate\[\int\limits_{- 4}^2 \left| x + 1 \right| dx\]. What does the value of this integral represent on the graph?
Find the area of the region in the first quadrant bounded by the parabola y = 4x2 and the lines x = 0, y = 1 and y = 4.
Using integration, find the area of the region bounded by the triangle whose vertices are (2, 1), (3, 4) and (5, 2).
Find the area of the region between the circles x2 + y2 = 4 and (x − 2)2 + y2 = 4.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Prove that the area common to the two parabolas y = 2x2 and y = x2 + 4 is \[\frac{32}{3}\] sq. units.
Find the area bounded by the parabola y = 2 − x2 and the straight line y + x = 0.
If An be the area bounded by the curve y = (tan x)n and the lines x = 0, y = 0 and x = π/4, then for x > 2
The area of the region bounded by the parabola (y − 2)2 = x − 1, the tangent to it at the point with the ordinate 3 and the x-axis is _________ .
The area bounded by the curve y = x4 − 2x3 + x2 + 3 with x-axis and ordinates corresponding to the minima of y is _________ .
The area of the region \[\left\{ \left( x, y \right) : x^2 + y^2 \leq 1 \leq x + y \right\}\] is __________ .
The area of the region (in square units) bounded by the curve x2 = 4y, line x = 2 and x-axis is
The area bounded by the curve y = x |x| and the ordinates x = −1 and x = 1 is given by
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
Sketch the graphs of the curves y2 = x and y2 = 4 – 3x and find the area enclosed between them.
The area of the region bounded by the curve y = x2 + x, x-axis and the line x = 2 and x = 5 is equal to ______.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
The area of the region bounded by the curve x = 2y + 3 and the y lines. y = 1 and y = –1 is ______.
Area of the region bounded by the curve `y^2 = 4x`, `y`-axis and the line `y` = 3 is:
Find the area of the region bounded by the curve `y = x^2 + 2, y = x, x = 0` and `x = 3`
Let f : [–2, 3] `rightarrow` [0, ∞) be a continuous function such that f(1 – x) = f(x) for all x ∈ [–2, 3]. If R1 is the numerical value of the area of the region bounded by y = f(x), x = –2, x = 3 and the axis of x and R2 = `int_-2^3 xf(x)dx`, then ______.
Let P(x) be a real polynomial of degree 3 which vanishes at x = –3. Let P(x) have local minima at x = 1, local maxima at x = –1 and `int_-1^1 P(x)dx` = 18, then the sum of all the coefficients of the polynomial P(x) is equal to ______.
Evaluate:
`int_0^1x^2dx`
Hence find the area bounded by the curve, y = x |x| and the coordinates x = −1 and x = 1.
