Question

Sketch the region bounded by the curves `y=sqrt(5-x^2)` and y=|x-1| and find its area using integration.

Answer 1

Consider the given equation

`y=sqrt(5-x^2)`

This equation represents a semicircle with centre at

the origin and radius = sqrt5 units

Given that the region is bounded by the above
semicircle and the line y = |x-1|
Let us find the point of intersection of the
given curve meets the line y= |x - 1|

`=>sqrt(5-x^2)=|x-1|`

Squaring both the sides, we have,

`5-x^2=|x-1|^2`

`=>5-x^2=x^2+1-2x`

`=>2x^2-2x-5+1=0`

`=>2x^2-2x-4=0`

`=>x^2-x-2=0`

`=>x^2-2x+x-2=0`

`=>x(x-2)+1(x-2)=0`

`=>(x+1)(x-2)=0`

`=>x=-1, x=2`

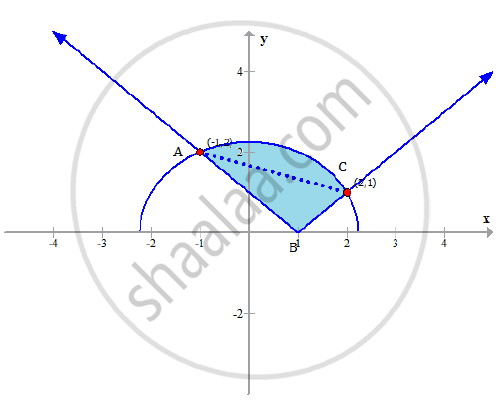
When x = -1,y = 2
When x = 2,y = 1
Consider the following figure.

Thus the intersection points are ( -1,2) and (2,1)
Consider the following sketch of the bounded region.

Required Area, A= `int_(-1)^2(y_2-y_1)dx`

=`=int_(-1)^1[sqrt(5-x^2)+(x-1)]dx+int_1^2[sqrt(5-x^2)-(x-1)]dx`

`=int_(-1)^1sqrt(5-x^2)dx+int_(-1)^1xdx-int_(-1)^1dx+int_1^2sqrt(5-x^2)dx-int_1^2xdx+int_1^2dx`

`=[x/2sqrt(5-x^2)+5/2sin^-1(x/sqrt5)]_(-1)^1+(x^2/2)_(-1)^1-(x)_(-1)^1+[x/2sqrt(5-x^2)+5/2sin^-1(x/sqrt5)]_1^2-(x^2/2)_(1)^2+(x)_(1)^2`

`=5/2 sin^-1 (1/sqrt5)+5/2 sin^-1 (2/sqrt5)-1/2`

Required area= `[5/2 sin^-1 (1/sqrt5)+5/2 sin^-1 (2/sqrt5)-1/2 ] sq.units`