Advertisements
Advertisements
प्रश्न
A (5, 4), B (-3, -2) and C (1, -8) are the vertices of a triangle ABC. Find the equations of the median AD and line parallel to AC passing through the point B.
A(5, 4), B(–3, –2) and C(1, –8) are the vertices of a triangle ABC. Find the equation of median AD
उत्तर १
AD is the median
∴ CD BD ... (∵ D is the midpoint of BC)
Coordinates of D can be found by using section formula.
Let (x,y) be coordinates of the centre of the circle.
`(x,y)=((1-3)/(1+1),(-8-2)/(1+1))=(-1,-5)`
Coordinates of point D are (-1,-5)
Let m be the slope of AD.
Coordinates of A(5, 4) and D(–1, –5)
`m=(y_2-y_1)/(x_2-x_1)=(4-(-5))/(5-(-1))=9/6=3/2`
Equation of line is y = mx+c, where c is the y int ercept.
`4=(3 xx 5)/2+c ...(" Substituting the coordinates of A ")`
c=-7/2
Equation of line of AD is
`y="3x"/2-7/2`
2y =3x - 7 is equation of the median AD.
The coordinates of A (5,4) and C (1, -8)
Slope of AC = `(y_2-y_1)/(x_2-x_1)=-12/-4=3`
Substituting the coordinates of A(5,4) in the equation y = mx + c
4 = (3x5)+c
c=-11
Line parallel to AC and pas sing through B(-3, -2) has slope 3
Substituting the coordinates of B 3, 2 in the equation y=mx+c
-2=(3x -3)+c
c=7
Equation of line parallel to AC and passing through B(-3,-2) is y=3x+7
उत्तर २
Let A(5,4) ≡ `(x_1,y_1)`; B(-3, -2) ≡ `(x_2,y_2)` and C(1,-8) ≡ `(x_3,y_3)`
D(x, y) is the midpoint of BC.
∴ the coordinates of `D = ((x_2 + x_3)/2, (y_2+y+_3)/2)`
`= ((-3+1)/2, (-2-8)/2) = (-2/2, -10/2) = (-1,-5)`
Let D(-1,-5) ≡ `(x_4,y_4)`
The equation of median AD is
`(x - x_1)/(x_1-x_4) = (y - y_1)/(y_1 - y_4)`
`:. (x-5)/(5-(-1)) = (y-4)/(4-(-5))`
`:. (x-5)/(5+1) = (y-4)/(4+5)`
`:. (x - 5)/6 = (y-4)/9`
`:. (x-5)/2 = (y -4)/3`
Multiplying both the sides by 6,
3(x - 5) = 2(y - 4) ∴ 3x - 15 = 2y - 8
∴ 3x - 2y - 15 + 8 = 0 ∴ 3x - 2y - 7 = 0
APPEARS IN
संबंधित प्रश्न
If (4,-3) is a point on the line AB and slope of the line is (-2), write the equation of the line AB.
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Given 3x + 2y + 4 = 0
(i) express the equation in the form y = mx + c
(ii) Find the slope and y-intercept of the line 3x + 2y + 4 = 0
The equation of a line is x – y = 4. Find its slope and y-intercept. Also, find its inclination.
Is the line 3x + 2y = 5 parallel to the line x + 2y = 1?
Find the equation of the perpendicular bisector of the line segment obtained on joining the points (6, −3) and (0, 3).
B(−5, 6) and D(1, 4) are the vertices of rhombus ABCD. Find the equations of diagonals BD and AC.
- Write down the equation of the line AB, through (3, 2) and perpendicular to the line 2y = 3x + 5.
- AB meets the x-axis at A and the y-axis at B. Write down the co-ordinates of A and B. Calculate the area of triangle OAB, where O is the origin.
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
The points A, B and C are (4, 0), (2, 2) and (0, 6) respectively. Find the equations of AB and BC. If AB cuts the y-axis at P and BC cuts the x-axis at Q, find the co-ordinates of P and Q.
Find the point on the X–axis which is equidistant from A(–3, 4) and B(1, –4).
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
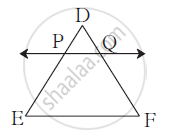
In Δ DEF, line PQ || side EF, If DP = 2.4,
PE = 7.2, DQ = 1 then find QF.
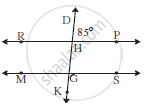
In the adjoining figure line RP ||line MS , line DK is a transversal . If ∠DHP = 85° find ∠RHG and ∠HGS.
Line PQ is parallel to line RS where points P,Q,R and S have
co-ordinates (2, 4), (3, 6), (3, 1) and (5, k) respectively. Find value of k.
A straight line passes through the points P(–1, 4) and Q(5, –2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find:
- the equation of the line.
- the co-ordinates of point A and B.
- the co-ordinates of point M.
In the given figure, line AB meets y-axis at point A. Line through C(2, 10) and D intersects line AB at right angle at point P. Find:
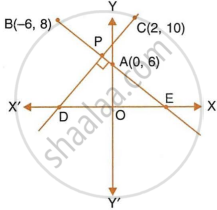
- equation of line AB.
- equation of line CD.
- co-ordinates of points E and D.
If (4,-3) is a point on line 5x +8y = c, find the value of c.
