Advertisements
Advertisements
प्रश्न
Answer the following:
Find the domain of the following function.
f(x) = `sqrt(x - x^2) + sqrt(5 - x)`
उत्तर
f(x) = `sqrt(x - x^2) + sqrt(5 - x)`
x – x2 ≥ 0
∴ x2 – x ≤ 0
∴ x(x – 1) ≤ 0
∴ 0 ≤ x ≤ 1 ...(i)
5 – x ≥ 0
∴ x ≤ 5 ...(ii)
Intersection of intervals given in (i) and (ii) gives
Solution set = [0, 1]
∴ Domain = [0, 1]
APPEARS IN
संबंधित प्रश्न
f, g, h are three function defined from R to R as follow:
(iii) h(x) = x2 + 1
Find the range of function.
If for non-zero x, af(x) + bf \[\left( \frac{1}{x} \right) = \frac{1}{x} - 5\] , where a ≠ b, then find f(x).
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iii) f g
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(i) f + g
If f, g and h are real functions defined by
Write the domain and range of function f(x) given by
Let A and B be two sets such that n(A) = p and n(B) = q, write the number of functions from A to B.
Let f and g be two functions given by
f = {(2, 4), (5, 6), (8, −1), (10, −3)} and g = {(2, 5), (7, 1), (8, 4), (10, 13), (11, −5)}.
Find the domain of f + g
Let A = {1, 2, 3} and B = {2, 3, 4}. Then which of the following is a function from A to B?
If f : Q → Q is defined as f(x) = x2, then f−1 (9) is equal to
If f(x) = cos (log x), then the value of f(x2) f(y2) −
If \[f\left( x \right) = \log \left( \frac{1 + x}{1 - x} \right)\] , then \[f\left( \frac{2x}{1 + x^2} \right)\] is equal to
If x ≠ 1 and \[f\left( x \right) = \frac{x + 1}{x - 1}\] is a real function, then f(f(f(2))) is
Let f(x) = x, \[g\left( x \right) = \frac{1}{x}\] and h(x) = f(x) g(x). Then, h(x) = 1
If \[f\left( x \right) = 64 x^3 + \frac{1}{x^3}\] and α, β are the roots of \[4x + \frac{1}{x} = 3\] . Then,
The domain of definition of \[f\left( x \right) = \sqrt{\frac{x + 3}{\left( 2 - x \right) \left( x - 5 \right)}}\] is
The domain of definition of the function f(x) = log |x| is
Let \[f\left( x \right) = \sqrt{x^2 + 1}\ ] . Then, which of the following is correct?
Which of the following relations are functions? If it is a function determine its domain and range:
{(0, 0), (1, 1), (1, −1), (4, 2), (4, −2), (9, 3), (9, −3), (16, 4), (16, −4)}
If f(x) = 3x + a and f(1) = 7 find a and f(4).
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 3), (4, 1), (2, 2)}
Check if the relation given by the equation represents y as function of x:
x + y2 = 9
Find the domain and range of the following function.
f(x) = 7x2 + 4x − 1
Express the area A of circle as a function of its radius r
Check the injectivity and surjectivity of the following function.
f : Z → Z given by f(x) = x2
Express the following logarithmic equation in exponential form
`log_(1/2) (8)` = – 3
Given that log 2 = a and log 3 = b, write `log sqrt(96)` in terms of a and b
Answer the following:
If f(x) = 3x + a and f(1) = 7 find a and f(4)
Answer the following:
Find x, if x = 33log32
Answer the following:
Find the range of the following function.
f(x) = [x] – x
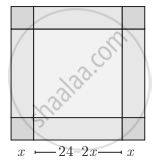
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
The data in the adjacent table depicts the length of a person's forehand and their corresponding height. Based on this data, a student finds a relationship between the height (y) and the forehand length (x) as y = ax + b, where a, b are constant.
| Length ‘x’ of forehand (in cm) |
Height 'y' (in inches) |
| 35 | 56 |
| 45 | 65 |
| 50 | 69.5 |
| 55 | 74 |
Find the length of forehand of a person if the height is 53.3 inches
If f(x) = `(x - 1)/(x + 1), x ≠ - 1` Show that f(f(x)) = `- 1/x`, Provided x ≠ 0
Redefine the function which is given by f(x) = `|x - 1| + |1 + x|, -2 ≤ x ≤ 2`
Find the domain of the following functions given by f(x) = `1/sqrt(x + |x|)`
Range of f(x) = `1/(1 - 2 cosx)` is ______.
Let f be a function with domain [–3, 5] and let g(x) = | 3x + 4 |. Then, the domain of (fog) (x) is ______.
Range of the function f(x) = `x/(1 + x^2)` is ______.
