Advertisements
Advertisements
प्रश्न
Compare the areas under the curves y = cos2 x and y = sin2 x between x = 0 and x = π.
उत्तर
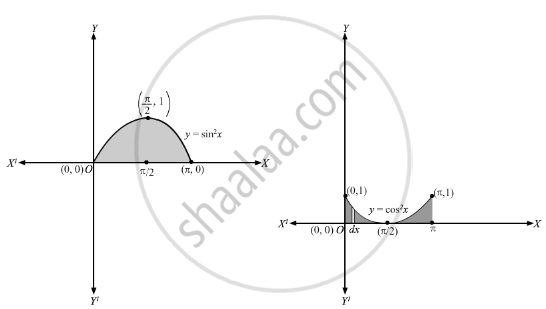
|
X |
0 | \[\frac{\pi}{4}\] |
\[\frac{\pi}{3}\]
|
\[\frac{\pi}{2}\]
|
\[\frac{2\pi}{3}\]
|
\[\frac{5\pi}{6}\]
|
\[\pi\]
|
|
\[y = \cos^2 x\]
|
1 | 0.5 | 0.25 | 0 | 0.25 | 0.75 | 1 |
|
\[y = \sin^2 x\]
|
0 | 0.5 | 0.75 | 1 | 0.75 | 0.25 | 0 |
Let A1 be the area of curve \[y = \cos^2 x\text{ between }x = 0 \text{ and }x = \pi\]
Let A2 be the area of curve \[y = \sin^2 x \text{ between }x = 0\text{ and }x = \pi\]
Consider, a vertical strip of length \[= \left| y \right|\] and width \[= dx\] in the shaded region of both the curves
The area of approximating rectangle \[= \left| y \right| dx\]
\[\text{The approximating rectangle moves from}x = 0\text{ to }x = \pi\]
\[ A_1 = \int_0^\pi \left| y \right| dx\]
\[ \Rightarrow A_1 = \int_0^\pi y dx ..................\left[ 0 \leq x \leq \pi , y > 0 \Rightarrow \left| y \right| = y \right]\]
\[ \Rightarrow A_1 = \int_0^\pi \cos^2 x dx\]
\[ \Rightarrow A_1 = \int_0^\pi \left( 1 + cos 2x \right) dx .................\left[ \cos^2 x = \left( 1 + \cos 2x \right) \right]\]
\[ \Rightarrow A_1 = \frac{1}{2} \left[ x + \frac{\sin 2x}{2} \right]_0^\pi \]
\[ \Rightarrow A_1 = \frac{1}{2}\left[ \pi + \frac{\sin 2\pi}{2} - 0 \right]\]
\[ \Rightarrow A_1 = \frac{\pi}{2} \text{ Sq . units }\]
Also,
\[ A_2 = \int_0^\pi \left| y \right| dx\]
\[ \Rightarrow A_2 = \int_0^\pi y dx .................\left[ 0 \leq x \leq \pi , y > 0 \Rightarrow \left| y \right| = y \right]\]
\[ \Rightarrow A_2 = \int_0^\pi \sin^2 x dx\]
\[ \Rightarrow A_2 = \left[ \frac{x}{2} - \frac{1}{2}\frac{\sin 2x}{2} \right]_0^\pi \]
\[ \Rightarrow A_2 = \frac{\pi}{2} - \left( \frac{1}{2}\frac{\sin 2\pi}{2} \right)\]
\[ \Rightarrow A_2 = \frac{\pi}{2} sq . units\]
\[ \therefore\text{ Area of curves }y = \cos^2 x\text{ and area of curve }y = \sin^2 x \text{ are both equal to }\frac{\pi}{2}\text{ sq . units }\]
APPEARS IN
संबंधित प्रश्न
Find the area bounded by the curve y2 = 4ax, x-axis and the lines x = 0 and x = a.
triangle bounded by the lines y = 0, y = x and x = 4 is revolved about the X-axis. Find the volume of the solid of revolution.
Find the area of the region common to the circle x2 + y2 =9 and the parabola y2 =8x
Sketch the region bounded by the curves `y=sqrt(5-x^2)` and y=|x-1| and find its area using integration.
Find the area bounded by the curve y = sin x between x = 0 and x = 2π.
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5
Find the area under the curve y = \[\sqrt{6x + 4}\] above x-axis from x = 0 to x = 2. Draw a sketch of curve also.
Draw a rough sketch of the curve y = \[\frac{\pi}{2} + 2 \sin^2 x\] and find the area between x-axis, the curve and the ordinates x = 0, x = π.
Find the area of the region bounded by x2 = 16y, y = 1, y = 4 and the y-axis in the first quadrant.
Calculate the area of the region bounded by the parabolas y2 = x and x2 = y.
Using integration, find the area of the region bounded by the triangle whose vertices are (2, 1), (3, 4) and (5, 2).
Find the area of the region {(x, y) : y2 ≤ 8x, x2 + y2 ≤ 9}.
Find the area of the region between the circles x2 + y2 = 4 and (x − 2)2 + y2 = 4.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Find the area of the region bounded by the curves y = x − 1 and (y − 1)2 = 4 (x + 1).
Using integration find the area of the region:
\[\left\{ \left( x, y \right) : \left| x - 1 \right| \leq y \leq \sqrt{5 - x^2} \right\}\]
Make a sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 3; 0 ≤ y ≤ 2x + 3; 0 ≤ x ≤ 3} and find its area using integration.
Find the area enclosed by the curves 3x2 + 5y = 32 and y = | x − 2 |.
If the area bounded by the parabola \[y^2 = 4ax\] and the line y = mx is \[\frac{a^2}{12}\] sq. units, then using integration, find the value of m.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
The area bounded by the curve y = loge x and x-axis and the straight line x = e is ___________ .
The area bounded by y = 2 − x2 and x + y = 0 is _________ .
The area bounded by the curves y = sin x between the ordinates x = 0, x = π and the x-axis is _____________ .
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
The area bounded by the curve y = 4x − x2 and the x-axis is __________ .
The area bounded by the y-axis, y = cos x and y = sin x when 0 ≤ x ≤ \[\frac{\pi}{2}\] is _________ .
Find the area of the region above the x-axis, included between the parabola y2 = ax and the circle x2 + y2 = 2ax.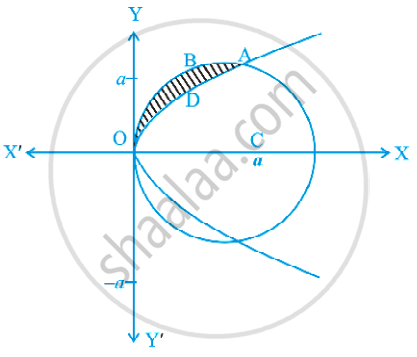
Find the area of the region bounded by the curves y2 = 9x, y = 3x
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
Area of the region bounded by the curve y = |x + 1| + 1, x = –3, x = 3 and y = 0 is
Area of the region bounded by the curve `y^2 = 4x`, `y`-axis and the line `y` = 3 is:
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.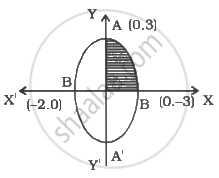
The area (in square units) of the region bounded by the curves y + 2x2 = 0 and y + 3x2 = 1, is equal to ______.
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
