Advertisements
Advertisements
प्रश्न
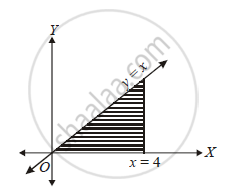
triangle bounded by the lines y = 0, y = x and x = 4 is revolved about the X-axis. Find the volume of the solid of revolution.
उत्तर
Given line is y = x
`V=piint_0^4 y^2dx`
`=pi int_0^4x^2dx`
`=pi/3[x^3]_0^4`
`=(64pi)/3 cubic units.`
APPEARS IN
संबंधित प्रश्न
Using integration, find the area bounded by the curve x2 = 4y and the line x = 4y − 2.
Find the area of the sector of a circle bounded by the circle x2 + y2 = 16 and the line y = x in the ftrst quadrant.
Prove that the curves y2 = 4x and x2 = 4y divide the area of square bounded by x = 0, x = 4, y = 4 and y = 0 into three equal parts.
Using the method of integration find the area of the region bounded by lines: 2x + y = 4, 3x – 2y = 6 and x – 3y + 5 = 0
Area bounded by the curve y = x3, the x-axis and the ordinates x = –2 and x = 1 is ______.
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Sketch the graph y = | x + 3 |. Evaluate \[\int\limits_{- 6}^0 \left| x + 3 \right| dx\]. What does this integral represent on the graph?
Find the area of the region bounded by the curve xy − 3x − 2y − 10 = 0, x-axis and the lines x = 3, x = 4.
Find the area bounded by the curve y = cos x, x-axis and the ordinates x = 0 and x = 2π.
Find the area of the region bounded by y =\[\sqrt{x}\] and y = x.
Find the area bounded by the curve y = 4 − x2 and the lines y = 0, y = 3.
Prove that the area common to the two parabolas y = 2x2 and y = x2 + 4 is \[\frac{32}{3}\] sq. units.
Find the area of the region bounded by \[y = \sqrt{x}\] and y = x.
Find the area bounded by the parabola y = 2 − x2 and the straight line y + x = 0.
Using integration find the area of the region bounded by the curves \[y = \sqrt{4 - x^2}, x^2 + y^2 - 4x = 0\] and the x-axis.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
The area of the region formed by x2 + y2 − 6x − 4y + 12 ≤ 0, y ≤ x and x ≤ 5/2 is ______ .
The area of the region (in square units) bounded by the curve x2 = 4y, line x = 2 and x-axis is
The area bounded by the curve y = f (x), x-axis, and the ordinates x = 1 and x = b is (b −1) sin (3b + 4). Then, f (x) is __________ .
The area bounded by the y-axis, y = cos x and y = sin x when 0 ≤ x ≤ \[\frac{\pi}{2}\] is _________ .
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
Area of the region bounded by the curve y2 = 4x, y-axis and the line y = 3, is
Find the area of the region bounded by the curve ay2 = x3, the y-axis and the lines y = a and y = 2a.
Find the area of the region bounded by the curve y2 = 4x, x2 = 4y.
Find the area of the region bounded by y = `sqrt(x)` and y = x.
Find the area enclosed by the curve y = –x2 and the straight lilne x + y + 2 = 0
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
The area of the region bounded by the curve x2 = 4y and the straight line x = 4y – 2 is ______.
The curve x = t2 + t + 1,y = t2 – t + 1 represents
Area of the region bounded by the curve y = |x + 1| + 1, x = –3, x = 3 and y = 0 is
Find the area of the region bounded by `y^2 = 9x, x = 2, x = 4` and the `x`-axis in the first quadrant.
What is the area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3.
Make a rough sketch of the region {(x, y): 0 ≤ y ≤ x2, 0 ≤ y ≤ x, 0 ≤ x ≤ 2} and find the area of the region using integration.
Find the area of the region bounded by curve 4x2 = y and the line y = 8x + 12, using integration.
The area enclosed by y2 = 8x and y = `sqrt(2x)` that lies outside the triangle formed by y = `sqrt(2x)`, x = 1, y = `2sqrt(2)`, is equal to ______.
Let the curve y = y(x) be the solution of the differential equation, `("dy")/("d"x) = 2(x + 1)`. If the numerical value of area bounded by the curve y = y(x) and x-axis is `(4sqrt(8))/3`, then the value of y(1) is equal to ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
