Advertisements
Advertisements
प्रश्न
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2
उत्तर
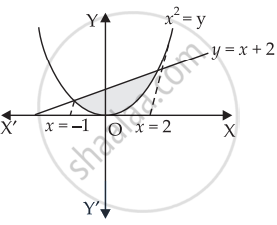
Here, x2 = y and y = x + 2
∴ x2 = x + 2
⇒ x2 – x – 2 = 0
⇒ x2 – 2x + x – 2 = 0
⇒ x(x –2) + 1(x – 2) = 0
⇒ (x – 2)(x + 1) = 0
∴ x = –1, 2
Graph of y = x + 2
| x | 0 | –2 |
| y | 2 | 0 |
Area of the required region
= `int_(-1)^2 (x + 2)"d"x - int_(-1)^2 x^2 "d"x`
= `[x^2/2 + 2x]_(-1)^2 - 1/3[x^3]_-1^2`
= `[(4/2 + 4) - (1/2 - 2)] - 1/3 [8 - (-1)]`
= `(6 + 3/2) - 1/3(9)`
= `15/2 - 3`
= `9/2` sq.units
Hence, the required area = `9/2` sq.units
APPEARS IN
संबंधित प्रश्न
Using the method of integration, find the area of the triangular region whose vertices are (2, -2), (4, 3) and (1, 2).
The area bounded by the curve y = x | x|, x-axis and the ordinates x = –1 and x = 1 is given by ______.
[Hint: y = x2 if x > 0 and y = –x2 if x < 0]
Find the area of ellipse `x^2/1 + y^2/4 = 1`
Sketch the graph of y = \[\sqrt{x + 1}\] in [0, 4] and determine the area of the region enclosed by the curve, the x-axis and the lines x = 0, x = 4.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Sketch the graph y = | x − 5 |. Evaluate \[\int\limits_0^1 \left| x - 5 \right| dx\]. What does this value of the integral represent on the graph.
Find the area of the region bounded by y =\[\sqrt{x}\] and y = x.
Using integration, find the area of the region bounded by the triangle ABC whose vertices A, B, C are (−1, 1), (0, 5) and (3, 2) respectively.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Using integration find the area of the region:
\[\left\{ \left( x, y \right) : \left| x - 1 \right| \leq y \leq \sqrt{5 - x^2} \right\}\]
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using horizontal strips.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
If the area above the x-axis, bounded by the curves y = 2kx and x = 0, and x = 2 is \[\frac{3}{\log_e 2}\], then the value of k is __________ .
The area bounded by the curve y = loge x and x-axis and the straight line x = e is ___________ .
The area bounded by the parabola y2 = 4ax and x2 = 4ay is ___________ .
The area bounded by the parabola y2 = 4ax, latusrectum and x-axis is ___________ .
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
Area of the region bounded by the curve y2 = 4x, y-axis and the line y = 3, is
Find the coordinates of a point of the parabola y = x2 + 7x + 2 which is closest to the straight line y = 3x − 3.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using vertical strips.
The area of the region bounded by the curve y = x2 and the line y = 16 ______.
The area of the region bounded by the curve y = x2 + x, x-axis and the line x = 2 and x = 5 is equal to ______.
Sketch the region `{(x, 0) : y = sqrt(4 - x^2)}` and x-axis. Find the area of the region using integration.
Find the area bounded by the lines y = 4x + 5, y = 5 – x and 4y = x + 5.
The area bounded by `y`-axis, `y = cosx` and `y = sinx, 0 ≤ x - (<pi)/2` is
The area enclosed by y2 = 8x and y = `sqrt(2x)` that lies outside the triangle formed by y = `sqrt(2x)`, x = 1, y = `2sqrt(2)`, is equal to ______.
The area of the region S = {(x, y): 3x2 ≤ 4y ≤ 6x + 24} is ______.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
