Advertisements
Advertisements
प्रश्न
Solve for x.
2 log10 x = `1 + log_10 (x + 11/10)`
उत्तर
2 log10 x = `1 + log_10 (x + 11/10)`
∴ `log_10 x^2 - log_10 (x + 11/10)` = 1 ...[n log m = log mn]
∴ `log_10 x^2 - log_10((10x + 11)/10)` = 1
∴ `log_10 (x^2/((10 x + 11)/10))` = 1 ...`[log"m" - log "n" = log "m"/"n"]`
∴ `log_10 ((10x^2)/(10x + 11))` = log10 10 ...[∵ loga a = 1]
∴ `(10x^2)/(10x + 11)` = 10
∴ `x^2/(10x + 11)` = 1
∴ x2 = 10x + 11
∴ x2 – 10x – 11 = 0
∴ (x – 11)(x + 1) = 0
∴ x = 11 or x = – 1
But log of negative number does not exist
∴ x ≠ – 1
∴ x = 11
APPEARS IN
संबंधित प्रश्न
Let A = {9, 10, 11, 12, 13} and let f: A → N be defined by f(n) = the highest prime factor of n. Find the range of f.
f, g, h are three function defined from R to R as follow:
(iii) h(x) = x2 + 1
Find the range of function.
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(c) f3 = {(1, 5), (2, 9), (3, 1), (4, 5), (2, 11)}
If f(x) = (x − a)2 (x − b)2, find f(a + b).
If \[y = f\left( x \right) = \frac{ax - b}{bx - a}\] , show that x = f(y).
If \[f\left( x \right) = \frac{2x}{1 + x^2}\] , show that f(tan θ) = sin 2θ.
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(iii) \[\frac{f}{g}\]
Let A = {1, 2, 3} and B = {2, 3, 4}. Then which of the following is a function from A to B?
If f(x) = cos (log x), then the value of f(x2) f(y2) −
Let f(x) = x, \[g\left( x \right) = \frac{1}{x}\] and h(x) = f(x) g(x). Then, h(x) = 1
If \[f\left( x \right) = \frac{\sin^4 x + \cos^2 x}{\sin^2 x + \cos^4 x}\] for x ∈ R, then f (2002) =
The function f : R → R is defined by f(x) = cos2 x + sin4 x. Then, f(R) =
If f : [−2, 2] → R is defined by \[f\left( x \right) = \begin{cases}- 1, & \text{ for } - 2 \leq x \leq 0 \\ x - 1, & \text{ for } 0 \leq x \leq 2\end{cases}\] , then
{x ∈ [−2, 2] : x ≤ 0 and f (|x|) = x} =
If f(m) = m2 − 3m + 1, find f(− x)
Which of the following relations are functions? If it is a function determine its domain and range:
{(0, 0), (1, 1), (1, −1), (4, 2), (4, −2), (9, 3), (9, −3), (16, 4), (16, −4)}
If f(m) = m2 − 3m + 1, find `(("f"(2 + "h") - "f"(2))/"h"), "h" ≠ 0`
Find the domain and range of the following function.
f(x) = `sqrt((x - 2)(5 - x)`
An open box is made from a square of cardboard of 30 cms side, by cutting squares of length x centimeters from each corner and folding the sides up. Express the volume of the box as a function of x. Also find its domain
Check the injectivity and surjectivity of the following function.
f : Z → Z given by f(x) = x2
Check the injectivity and surjectivity of the following function.
f : N → N given by f(x) = x3
Express the following logarithmic equation in exponential form
`log_(1/2) (8)` = – 3
Solve for x.
log2 + log(x + 3) – log(3x – 5) = log3
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
Answer the following:
Identify the following relation is the function? If it is a function determine its domain and range
{(12, 1), (3, 1), (5, 2)}
Answer the following:
A function f is defined as : f(x) = 5 – x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 5
Answer the following:
Find x, if x = 33log32
Answer the following:
If f(x) = log(1 – x), 0 ≤ x < 1 show that `"f"(1/(1 + x))` = f(1 – x) – f(– x)
Answer the following:
Find the range of the following function.
f(x) = 1 + 2x + 4x
A graph representing the function f(x) is given in it is clear that f(9) = 2
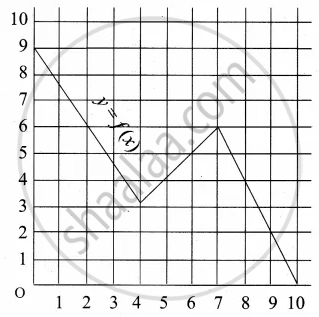
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
The function f and g are defined by f(x) = 6x + 8; g(x) = `(x - 2)/3`
Calculate the value of `"gg" (1/2)`
Let A = {1, 2, 3, 4} and B = N. Let f : A → B be defined by f(x) = x3 then, find the range of f
If f(x) = 5x - 3, then f-1(x) is ______
Find the range of the following functions given by f(x) = |x − 3|
The domain of the function f(x) = `sin^-1((|x| + 5)/(x^2 + 1))` is (–∞, –a] ≈ [a, ∞). Then a is equal to ______.
The expression \[\begin{array}{cc}\log_p\log_p\sqrt[p]{\sqrt[p]{\sqrt[p]{\text{...........}\sqrt[p]{p}}}}\\
\phantom{...........}\ce{\underset{n radical signs}{\underline{\uparrow\phantom{........}\uparrow}}}
\end{array}\]where p ≥ 2, p ∈ N; ∈ N when simplified is ______.
If f: R `rightarrow` R be a function defined by f(x) = 4x3 – 7. Then ______.
