Advertisements
Advertisements
प्रश्न
Using integration, find the area of the triangle whose vertices are (2, 3), (3, 5) and (4, 4).
उत्तर १
The Vertices of ΔABC are A (2, 3), B (3, 5), and C (4, 4)
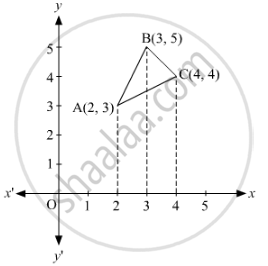
Equation of line segment AB is
`("y" - 5) = (5-3)/(3-2) ("x" -3)`
=`"y" -5 = 2 ("x" -3)`
=`"y" = 2"x" -1`
Equation of line segment BC is
`("y" - 5) = (5-3)/(3-4) ("x" -3)`
=`"y" -5 = -1 ("x" -3)`
=`"y" = -"x" + 8`
Equation of line segment AC is
`("y" - 4) = (4-3)/(4-2) ("x" -4)`
=`"y" -4 = (1)/(2) ("x" -4)`
= `"y" = ("x")/(2) + 2`
∴ Area of ΔABC = `int_2^3 [(2"x" -1) - ("x"/2+2)] d"x" + int_3^4 [(-"x" + 8) - ("x"/2 + 2)] . d"x"`
= `int_2^3 ((3"x")/2 -3) . d"x" + int_3^4 ((-3"x")/2 + 6) . d"x"`
= `[(3"x"^2)/(4) - 3"x"]_2^3 + [ (-3"x"^2)/4 + 6"x"]_3^4`
= `(27/4 - 9) - (3 -6) + (-12 + 24) - (-27/4 + 18)`
= `(3)/(2) "sq. units"`.
उत्तर २
The Vertices of ΔABC are A (2, 3), B (3, 5), and C (4, 4)
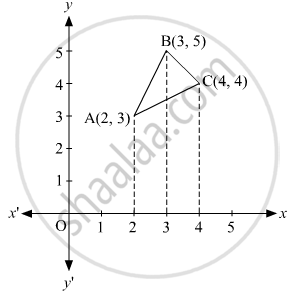
Equation of line segment AB is
`("y" - 5) = (5-3)/(3-2) ("x" -3)`
=`"y" -5 = 2 ("x" -3)`
=`"y" = 2"x" -1`
Equation of line segment BC is
`("y" - 5) = (5-3)/(3-4) ("x" -3)`
=`"y" -5 = -1 ("x" -3)`
=`"y" = -"x" + 8`
Equation of line segment AC is
`("y" - 4) = (4-3)/(4-2) ("x" -4)`
=`"y" -4 = (1)/(2) ("x" -4)`
= `"y" = ("x")/(2) + 2`
∴ Area of ΔABC = `int_2^3 [(2"x" -1) - ("x"/2+2)] d"x" + int_3^4 [(-"x" + 8) - ("x"/2 + 2)] . d"x"`
= `int_2^3 ((3"x")/2 -3) . d"x" + int_3^4 ((-3"x")/2 + 6) . d"x"`
= `[(3"x"^2)/(4) - 3"x"]_2^3 + [ (-3"x"^2)/4 + 6"x"]_3^4`
= `(27/4 - 9) - (3 -6) + (-12 + 24) - (-27/4 + 18)`
= `(3)/(2) "sq. units"`.
APPEARS IN
संबंधित प्रश्न
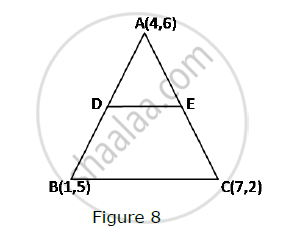
In Fig. 8, the vertices of ΔABC are A(4, 6), B(1, 5) and C(7, 2). A line-segment DE is drawn to intersect the sides AB and AC at D and E respectively such that `(AD)/(AB)=(AE)/(AC)=1/3 `Calculate th area of ADE and compare it with area of ΔABCe.
In Fig. 6, ABC is a triangle coordinates of whose vertex A are (0, −1). D and E respectively are the mid-points of the sides AB and AC and their coordinates are (1, 0) and (0, 1) respectively. If F is the mid-point of BC, find the areas of ∆ABC and ∆DEF.

The coordinates of A, B, C are (6, 3), (–3, 5) and (4, – 2) respectively and P is any point (x, y). Show that the ratio of the areas of triangle PBC and ABC is
Find the area of the triangle whose vertices are: (2, 3), (-1, 0), (2, -4)
Find the area of the triangle whose vertices are: (–5, –1), (3, –5), (5, 2)
Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0, -1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle
Determine the ratio in which the line 2x + y – 4 = 0 divides the line segment joining the points A(2, – 2) and B(3, 7).
Find a relation between x and y if the points (x, y), (1, 2) and (7, 0) are collinear.
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
The area of a triangle is 5 sq units. Two of its vertices are (2, 1) and (3, –2). If the third vertex is (`7/2`, y). Find the value of y
For what value of a point (a, 1), (1, -1) and (11, 4) are collinear?
Prove that the points (a, b), (a1, b1) and (a −a1, b −b1) are collinear if ab1 = a1b.
Find the area of Δ ABC whose vertices are:
A (1,2) B (-2,3) and C (-3,-4)
Find the area of ΔABC whose vertices are:
A(10,-6) , B (2,5) and C(-1,-3)
Find the value of x for which points A(x, 2), B(-3, -4) and C(7, -5) are collinear.
Prove that the points A (a,0), B( 0,b) and C (1,1) are collinear, if `( 1/a+1/b) =1`.
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
If the sides of a triangle are 3 cm, 4 cm and 5 cm, then the area is
The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| 20 cm | 40 cm | ? |
A field is in the shape of a right angled triangle whose base is 25 m and height 20 m. Find the cost of levelling the field at the rate of ₹ 45 per sq.m2
Let ∆ = `|("A"x, x^2, 1),("B"y, y^2, 1),("C"z, z^2, 1)|`and ∆1 = `|("A", "B", "C"),(x, y, z),(zy, zx, xy)|`, then ______.
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
The area of the triangle whose vertices are A(1, 2), B(-2, 3) and C(-3, -4) is ______.
If `D((-1)/2, 5/2), E(7, 3)` and `F(7/2, 7/2)` are the midpoints of sides of ∆ABC, find the area of the ∆ABC.
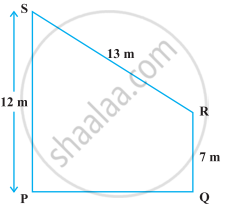
Find the area of the trapezium PQRS with height PQ given in the following figure.
The area of a trapezium is 475 cm2 and the height is 19 cm. Find the lengths of its two parallel sides if one side is 4 cm greater than the other.
Area of triangle MNO in the figure is ______.
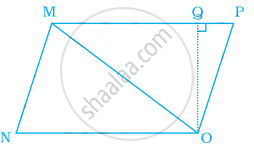
In the given figure, ΔMNO is a right-angled triangle. Its legs are 6 cm and 8 cm long. Length of perpendicular NP on the side MO is ______.

Observe all the four triangles FAB, EAB, DAB and CAB as shown in the given figure.

- All triangles have the same base and the same altitude.
- All triangles are congruent.
- All triangles are equal in area.
- All triangles may not have the same perimeter.
Ratio of the area of ∆WXY to the area of ∆WZY is 3:4 in the given figure. If the area of ∆WXZ is 56 cm2 and WY = 8 cm, find the lengths of XY and YZ.

