Advertisements
Advertisements
Question
If `sqrt(3)` sec 2θ = 2 and θ< 90°, find the value of
cos 3θ
Solution
`sqrt(3)`sec 2θ = 2
⇒ sec2θ = `(2)/sqrt(3)`
⇒ sec2θ = sec30°
⇒ 2θ = 30°
⇒ θ =15°
∴ cos3θ
= cos3 x 15°
= cos45°
= `(1)/sqrt(2)`.
APPEARS IN
RELATED QUESTIONS
Calculate the value of A, if cos 3A. (2 sin 2A - 1) = 0
If sin 3A = 1 and 0 < A < 90°, find `tan^2A - (1)/(cos^2 "A")`
If θ < 90°, find the value of: `tan^2θ - (1)/cos^2θ`
Find the value of 'x' in each of the following: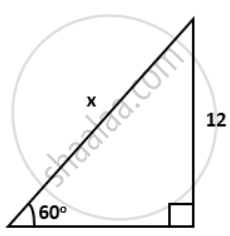
Find the value of 'x' in each of the following:
Find the value 'x', if: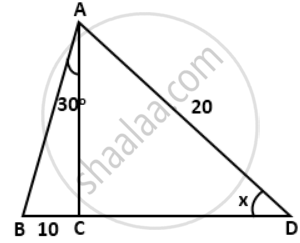
Find the value 'x', if:
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: cosec64° + sec70°
If sin(θ - 15°) = cos(θ - 25°), find the value of θ if (θ-15°) and (θ - 25°) are acute angles.
If secθ= cosec30° and θ is an acute angle, find the value of 4 sin2θ - 2 cos2θ.
