Advertisements
Advertisements
Question
If A, B, C are the angles of a triangle, then ∆ = `|(sin^2"A", cot"A", 1),(sin^2"B", cot"B", 1),(sin^2"C", cot"C", 1)|` = ______.
Solution
If A, B, C are the angles of a triangle, then ∆ = `|(sin^2"A", cot"A", 1),(sin^2"B", cot"B", 1),(sin^2"C", cot"C", 1)|` = 0.
Explanation:
Apply R2 → R2 – R1, R3 → R3 – R1.
APPEARS IN
RELATED QUESTIONS
If A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.
Prove that the points (2, – 2), (–3, 8) and (–1, 4) are collinear
For what value of k are the points (k, 2 – 2k), (–k + 1, 2k) and (–4 – k, 6 – 2k) are collinear ?
Find the area of the triangle whose vertices are: (2, 3), (-1, 0), (2, -4)
Find equation of line joining (1, 2) and (3, 6) using the determinant.
The four vertices of a quadrilateral are (1, 2), (−5, 6), (7, −4) and (k, −2) taken in order. If the area of the quadrilateral is zero, find the value of k.
Prove that the points (2a, 4a), (2a, 6a) and `(2a + sqrt3a, 5a)` are the vertices of an equilateral triangle.
The area of a triangle is 5. Two of its vertices are (2, 1) and (3, −2). The third vertex lies on y = x + 3. Find the third vertex.
Four points A (6, 3), B (−3, 5), C(4, −2) and D (x, 3x) are given in such a way that `(ΔDBG) /(ΔABG)=1/2,` find x
Find the angle subtended at the origin by the line segment whose end points are (0, 100) and (10, 0).
Find the area of a triangle whose sides are 9 cm, 12 cm and 15 cm ?
Show that the points are the vertices of an isosceles right triangle.
If G(-2, 1) is the centroid of a ΔABC and two of its vertices are A(1, -6) and B(-5, 2) , find the third vertex of the triangle.
Show that the following points are collinear:
A(5,1), B(1, -1) and C(11, 4)
Find the area of ΔABC with vertices A(0, -1), B(2,1) and C(0, 3). Also, find the area of the triangle formed by joining the midpoints of its sides. Show that the ratio of the areas of two triangles is 4:1.
In ☐ABCD, l(AB) = 13 cm, l(DC) = 9 cm, l(AD) = 8 cm, find the area of ☐ABCD.

Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
Using integration, find the area of the triangle whose vertices are (2, 3), (3, 5) and (4, 4).
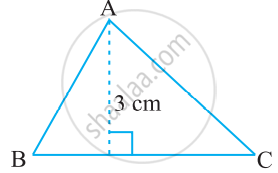
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
In a triangle ABC, if `|(1, 1, 1),(1 + sin"A", 1 + sin"B", 1 + sin"C"),(sin"A" + sin^2"A", sin"B" + sin^2"B", sin"C" + sin^2"C")|` = 0, then prove that ∆ABC is an isoceles triangle.
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
If the points (3, -2), (x, 2), (8, 8) are collinear, then find the value of x.
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
Find the area of the triangle whose vertices are (–8, 4), (–6, 6) and (–3, 9).
The dimensions of a rectangle ABCD are 51 cm × 25 cm. A trapezium PQCD with its parallel sides QC and PD in the ratio 9 : 8, is cut off from the rectangle as shown in the following figure. If the area of the trapezium PQCD is `5/6` th part of the area of the rectangle, find the lengths QC and PD.

In the given figure, if PR = 12 cm, QR = 6 cm and PL = 8 cm, then QM is ______.

In the given figure, ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of ΔACD.
Ratio of the area of ∆WXY to the area of ∆WZY is 3:4 in the given figure. If the area of ∆WXZ is 56 cm2 and WY = 8 cm, find the lengths of XY and YZ.

Find the missing value:
| Base | Height | Area of Triangle |
| 22 cm | ______ | 170.5 cm2 |
