Advertisements
Advertisements
Question
If f : R → R is defined by f(x) = x2, find f−1 (−25).
Solution
\[Let f^{- 1} \left( - 25 \right) = x\]
\[ \Rightarrow f\left( x \right) = - 25\]
\[ \Rightarrow x^2 = - 25\]
\[\text{We cannot find x } \in R,\text{such that} x^2 = - 25 \left( \text{ as x}^2 \geq0 \text{ for all } x \in R \right)\]
\[So, f^{- 1} \left( - 25 \right) = \phi\]
APPEARS IN
RELATED QUESTIONS
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f: R → R defined by f(x) = 1 + x2
Find the number of all onto functions from the set {1, 2, 3, …, n} to itself.
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Show that the exponential function f : R → R, given by f(x) = ex, is one-one but not onto. What happens if the co-domain is replaced by`R0^+` (set of all positive real numbers)?
Suppose f1 and f2 are non-zero one-one functions from R to R. Is `f_1 / f^2` necessarily one - one? Justify your answer. Here,`f_1/f_2 : R → R is given by (f_1/f_2) (x) = (f_1(x))/(f_2 (x)) for all x in R .`
Given A = {2, 3, 4}, B = {2, 5, 6, 7}. Construct an example of each of the following:
(i) an injective map from A to B
(ii) a mapping from A to B which is not injective
(iii) a mapping from A to B.
if `f (x) = sqrt(1-x)` and g(x) = `log_e` x are two real functions, then describe functions fog and gof.
Let
f (x) =`{ (1 + x, 0≤ x ≤ 2) , (3 -x , 2 < x ≤ 3):}`
Find fof.
Consider f : {1, 2, 3} → {a, b, c} and g : {a, b, c} → {apple, ball, cat} defined as f (1) = a, f (2) = b, f (3) = c, g (a) = apple, g (b) = ball and g (c) = cat. Show that f, g and gof are invertible. Find f−1, g−1 and gof−1and show that (gof)−1 = f −1o g−1
Show that the function f : Q → Q, defined by f(x) = 3x + 5, is invertible. Also, find f−1
If f : R → R be defined by f(x) = x3 −3, then prove that f−1 exists and find a formula for f−1. Hence, find f−1(24) and f−1 (5).
If f : R → (−1, 1) defined by `f (x) = (10^x- 10^-x)/(10^x + 10 ^-x)` is invertible, find f−1.
If f : R → (0, 2) defined by `f (x) =(e^x - e^(x))/(e^x +e^(-x))+1`is invertible , find f-1.
Let \[f : \left( - \frac{\pi}{2}, \frac{\pi}{2} \right) \to R\] be a function defined by f(x) = cos [x]. Write range (f).
Let `f : R - {- 3/5}` → R be a function defined as `f (x) = (2x)/(5x +3).`
f-1 : Range of f → `R -{-3/5}`.
Let f be an invertible real function. Write ( f-1 of ) (1) + ( f-1 of ) (2) +..... +( f-1 of ) (100 )
Write whether f : R → R, given by `f(x) = x + sqrtx^2` is one-one, many-one, onto or into.
What is the range of the function
`f (x) = ([x - 1])/(x -1) ?`
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. State whether f is one-one or not.
Let f, g : R → R be defined by f(x) = 2x + l and g(x) = x2−2 for all x
∈ R, respectively. Then, find gof. [NCERT EXEMPLAR]
The function
f : A → B defined by
f (x) = - x2 + 6x - 8 is a bijection if
If a function\[f : [2, \infty )\text{ to B defined by f}\left( x \right) = x^2 - 4x + 5\] is a bijection, then B =
\[f : R \to R\] is defined by
\[f\left( x \right) = \frac{e^{x^2} - e^{- x^2}}{e^{x^2 + e^{- x^2}}} is\]
The function
The function \[f : R \to R\] defined by
\[f\left( x \right) = 6^x + 6^{|x|}\] is
If \[g\left( x \right) = x^2 + x - 2\text{ and} \frac{1}{2} gof\left( x \right) = 2 x^2 - 5x + 2\] is equal to
Let
\[f : R \to R\] be given by \[f\left( x \right) = x^2 - 3\] Then, \[f^{- 1}\] is given by
Write about strlen() function.
For sets A, B and C, let f: A → B, g: B → C be functions such that g o f is injective. Then both f and g are injective functions.
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
g = {(1, 4), (2, 4), (3, 4)}
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
k(x) = x2
Let f: `[2, oo)` → R be the function defined by f(x) = x2 – 4x + 5, then the range of f is ______.
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.
Answer the following questions using the above information.
- Let f: {1,2,3,....} → {1,4,9,....} be defined by f(x) = x2 is ____________.
If f; R → R f(x) = 10x + 3 then f–1(x) is:
Prove that the function f is surjective, where f: N → N such that `f(n) = {{:((n + 1)/2",", if "n is odd"),(n/2",", if "n is even"):}` Is the function injective? Justify your answer.
For x ∈ R, x ≠ 0, let f0(x) = `1/(1 - x)` and fn+1 (x) = f0(fn(x)), n = 0, 1, 2, .... Then the value of `f_100(3) + f_1(2/3) + f_2(3/2)` is equal to ______.
Find the domain of sin–1 (x2 – 4).
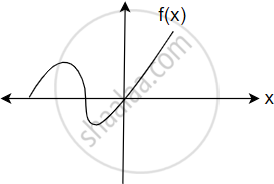
The given function f : R → R is not ‘onto’ function. Give reason.
