Advertisements
Advertisements
Question
In the adjoining figure, seg DE is the chord of the circle with center C. seg CF⊥ seg DE and DE = 16 cm, then find the length of DF?
Solution
In given figure, seg CF ⊥ Chord DE
∴ DF ≅ DE ......[[A perpendicular drawn from the centre of a circle on its chord bisects the chord]
∴ DF = `1/2` DE
= `1/2 xx 16` .....[Given]
∴ DF = 8 cm
APPEARS IN
RELATED QUESTIONS
Prove that there is one and only one tangent at any point on the circumference of a circle.
In fig., circles C(O, r) and C(O’, r/2) touch internally at a point A and AB is a chord of the circle C (O, r) intersecting C(O’, r/2) at C, Prove that AC = CB.
Write True or False. Give reasons for your answers.
A chord of a circle, which is twice as long as its radius, is a diameter of the circle.
If from any point on the common chord of two intersecting circles, tangents be drawn to circles, prove that they are equal.
From an external point P, tangents PA and PB are drawn to the circle with centre O. If CD is the tangent to the circle at point E and PA = 14 cm. Find the perimeter of ABCD.
Fill in the blank:
A point whose distance from the centre of a circle is greater than its radius lies in ..................... of the circle.
Find the length of tangent drawn to a circle with radius 8 cm form a point 17 cm away from the center of the circle
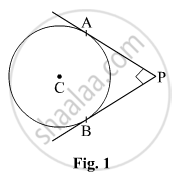
In Fig. 1, PA and PB are two tangents drawn from an external point P to a circle with centre C and radius 4 cm. If PA ⊥ PB, then the length of each tangent is:
In the given figure, AB and CD are diameters of a circle with centre O. If ∠OBD = 50°, find ∠AOC.

In the given figure, if ∠ABC = 45°, then ∠AOC =
The length of three concesutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm, and 7 cm respectively. Determine the length of the fourth side.
In an equilateral triangle, prove that the centroid and center of the circum-circle (circumcentre) coincide.
Use the figure given below to fill in the blank:
Diameter of a circle is ______.

Find the length of the chord AC where AB and CD are the two diameters perpendicular to each other of a circle with radius `4sqrt(2)` cm and also find ∠OAC and ∠OCA
A part of circumference of a circle is called as _______
In the figure, O is the centre of a circle, AB is a chord, and AT is the tangent at A. If ∠AOB = 100°, then ∠BAT is equal to ______

If angle between two tangents drawn from a point P to a circle of radius a and centre O is 60°, then OP = `asqrt(3)`
Two chords AB and AC of a circle subtends angles equal to 90º and 150º, respectively at the centre. Find ∠BAC, if AB and AC lie on the opposite sides of the centre.
Is every diameter of a circle also a chord?
