Advertisements
Advertisements
Question
Write the range of the real function f(x) = |x|.
Solution
Given:
f (x) = | x |, x ∈ R
We know that
\[\left| x \right| = \begin{cases}x, & x \geq 0 \\ - x & x < 0\end{cases}\]
It can be observed that the range of f (x) = | x | is all real numbers except negative real numbers.
∴ The range of f is [0, ∞) .
APPEARS IN
RELATED QUESTIONS
Define a function as a correspondence between two sets.
If f : R → R be defined by f(x) = x2 + 1, then find f−1 [17] and f−1 [−3].
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iii) f g
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iv) \[\frac{f}{g}\]
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
Which one of the following is not a function?
If f(x) = cos (log x), then value of \[f\left( x \right) f\left( 4 \right) - \frac{1}{2} \left\{ f\left( \frac{x}{4} \right) + f\left( 4x \right) \right\}\] is
The domain of the function \[f\left( x \right) = \sqrt{\frac{\left( x + 1 \right) \left( x - 3 \right)}{x - 2}}\] is
Check if the following relation is function:
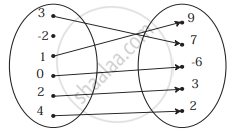
If f(x) = 3x + a and f(1) = 7 find a and f(4).
Check if the relation given by the equation represents y as function of x:
x2 − y = 25
Check if the relation given by the equation represents y as function of x:
2y + 10 = 0
Find x, if g(x) = 0 where g(x) = x3 − 2x2 − 5x + 6
Find x, if f(x) = g(x) where f(x) = x4 + 2x2, g(x) = 11x2
Express the area A of circle as a function of its diameter d
Express the following logarithmic equation in exponential form
log2 64 = 6
Express the following logarithmic equation in exponential form
`log_5 1/25` = – 2
Solve for x.
log2 x + log4 x + log16 x = `21/4`
If f(x) = 3x + 5, g(x) = 6x − 1, then find (fg) (3)
Select the correct answer from given alternatives
If f(x) = 2x2 + bx + c and f(0) = 3 and f(2) = 1, then f(1) is equal to
Answer the following:
A function f : R → R defined by f(x) = `(3x)/5 + 2`, x ∈ R. Show that f is one-one and onto. Hence find f–1
Answer the following:
Find x, if x = 33log32
Answer the following:
If `log (("a" + "b")/2) = 1/2(log"a" + log"b")`, then show that a = b
Answer the following:
If b2 = ac. prove that, log a + log c = 2 log b
Answer the following:
If `log ((x - y)/5) = 1/2 logx + 1/2 log y`, show that x2 + y2 = 27xy
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
Answer the following:
If `log_2"a"/4 = log_2"b"/6 = log_2"c"/(3"k")` and a3b2c = 1 find the value of k
Answer the following:
Find the domain of the following function.
f(x) = x!
Answer the following:
Find the domain of the following function.
f(x) = 5–xPx–1
Given the function f: x → x2 – 5x + 6, evaluate f(2)
The data in the adjacent table depicts the length of a person's forehand and their corresponding height. Based on this data, a student finds a relationship between the height (y) and the forehand length (x) as y = ax + b, where a, b are constant.
| Length ‘x’ of forehand (in cm) |
Height 'y' (in inches) |
| 35 | 56 |
| 45 | 65 |
| 50 | 69.5 |
| 55 | 74 |
Find the height of a person whose forehand length is 40 cm
Find the domain of the following function.
f(x) = [x] + x
The ratio `(2^(log_2 1/4 a) - 3^(log_27(a^2 + 1)^3) - 2a)/(7^(4log_49a) - a - 1)` simplifies to ______.
Which of the following functions is NOT one-one?
If f : R – {2} `rightarrow` R i s a function defined by f(x) = `(x^2 - 4)/(x - 2)`, then its range is ______.
lf f : [0, ∞) `rightarrow` [0, ∞) and f(x) = `x/(1 + x)`, then f is ______.
Range of the function f(x) = `x/(1 + x^2)` is ______.
