FLIP-FLOPS

A flip-flop, or bistable multivibrator, is essential in digital circuits like registers, counters, and memory. Constructed using gates, it serves as a memory element with two states: "set" (1) or "reset" (0). 

R-S Flip-Flop 

It is the basic circuit, which requires for constructing the other flip-flop circuits. It has two inputs 'S' and 'R' and two outputs denoted by 'Q' and `bar Q` where Q is called normal output and `bar Q` is called as complementary output.  

Truth Table & Symbol:  

R-S Flip Flop using Gates 

Due to integrated circuit technology, the flip-flop can be fabricated in the form of gates. Since NOR and NAND are building blocks, the working of a gate circuit can be explained with their 'disable' and 'enable' state. 

Disable State: If one NOR gate input is 1(high), the output is 0, regardless of the other input. This is the Disable state of NOR gate. 

Enable State: If one NAND gate input is 0(low), the output is 1, regardless of the other input. This is the Enable state of NAND gate. 

Working of NOR Flip-Flop: 

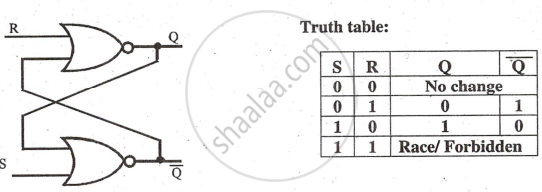
Case 1: When S = 0 and R = 0, the flip-flop remains in its last state ("No Change"). 

Case 2: When S = 0 and R = 1, the upper NOR gate is disabled, producing Q = 0. The lower NOR gate then produces Q = 1 ("Reset"). 

Case 3: When S = 1 and R = 0, the lower NOR gate is disabled, producing Q = 0. The upper NOR gate then produces Q = 1 ("Set"). 

Case 4: When S = 1 and R = 1, both NOR gates are disabled, leading to an unpredictable state ("Race" or forbidden state). 

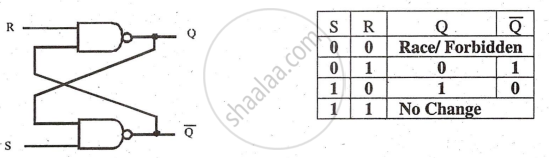
The NAND S flip-flop works similarly, considering the "Enable" state. When S = 1 and R = 1, there is no change (N/C). When S = 0 and R = 0, both NAND gates try to enable, causing a "Race" condition. This is the main difference 

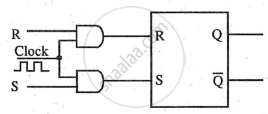
Clocked R-S Flip-Flop 

Theoretical circuits transmit inputs to outputs without control. Practically, a control input is added to R and S inputs for synchronization with the main circuit, using a common "CLOCK" signal. Fig. (2.23) shows a clocked R-S flip-flop and its truth table. 

Circuit 

Truth Table 

The flip-flop is enabled only when the clock is high; if the clock is low (0), the flip-flop remains in its last state (N/C). When the clock is absent or low, both AND gates are disabled, resulting in no input to the internal R-S flip-flop, thus no change in output. This state is indicated as "X," meaning "don't care." 

Clocked D Flip-Flop (Delay Flip-Flop) 

The D flip-flop can be derived from R-S flip-flop. The R-S flip-flop has two drawbacks:

1. It requires two separate inputs 'S' and 'R' 
2. The possibility of forbidden or race condition.

These drawbacks are eliminated in D flip-flop; this flip-flop needs a single data input 'D' 

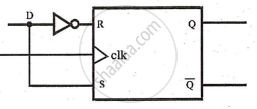
Circuit  

Truth Table 

When CLK = 1, D = 1 sets the flip-flop (Q=1, Q=0); D = 0 resets it. D flip-flops are used in registers and ring counters. 

J-K Flip Flop (JUMP and KEY Flip-Flop) 

The J-K flip-flop, built from an R-S flip-flop, toggles when J = 1 and K = 1, changing to the opposite state with each clock pulse. 

Circuit 

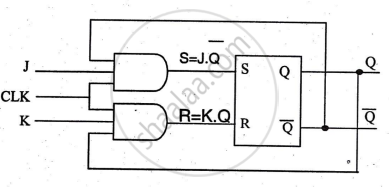
Truth Table 

In counter circuits, J-K flip-flops use edge-triggered clocks, either positive or negative edge. Fig (2.27) shows the J-K flip-flop symbol with terminals. Edge triggering, preferred to avoid false triggers, is achieved with an R-C differentiator circuit. 

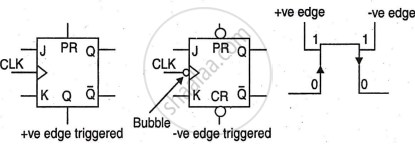
In a positive edge-triggered flip-flop, the output changes at the rising clock edge (0 to 1). In a negative edge-triggered flip-flop, it changes at the falling edge (1 to 0). The J-K flip-flop has PR (PRESET) to set the output and CR (CLEAR) to reset the output independently of J, K, and the clock.