Advertisements
Advertisements
प्रश्न
A 6⋅5 m long ladder rests against a vertical wall reaching a height of 6⋅0 m. A 60 kg man stands half way up the ladder.
- Find the torque of the force exerted by the man on the ladder about the upper end of the ladder.
- Assuming the weight of the ladder to be negligible as compared to the man and assuming the wall to be smooth, find the force exerted by the ground on the ladder.
उत्तर
Given
Mass of the man = m = 60 kg
Ladder length = 6.5 m
Height of the wall = 6 m
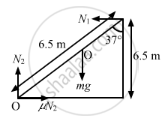
(a) We have to find the torque due to the weight of the body about the upper end of the ladder.
\[\tau = 60 \times 10 \times \frac{6 . 5}{2}\sin\theta\]
\[ \Rightarrow \tau = 600 \times \frac{6 . 5}{2} \times \sqrt{\left( 1 - \cos^2 \theta \right)}\]
\[ \Rightarrow \tau = 600 \times \left( \frac{6 . 5}{2} \right) \times \sqrt{\left\{ 1 - \left( \frac{6}{6 . 5} \right)^2 \right\}}\]
\[ \Rightarrow \tau = 740 N - m\]
(b) Let us find the vertical force exerted by the ground on the ladder.
\[N_2 = mg = 60 \times 9 . 8 = 588 N\]
Vertical force exerted by the ground on the ladder = \[\mu N_2 = N_1\]
As system is in rotational equilibrium, we have
\[\tau_{\text{net}} = 0 ............\left(\text{about O} \right)\]
\[\Rightarrow 6 . 5 N_1 \cos\theta = 60g \times \frac{6 . 5}{2}\sin\theta\]
\[\Rightarrow N_1 = \frac{1}{2}60g\tan\theta\]
\[= \frac{1}{2}60g \times \left( \frac{2 . 5}{6} \right) ...........\left[\text{using, }\tan\theta = \frac{2.5}{6} \right]\]
\[ \Rightarrow N_1 = \frac{25}{2}g\]
\[ \Rightarrow N_1 = 122 . 5 N \approx 120 N\]
APPEARS IN
संबंधित प्रश्न
Find the components along the x, y, z axes of the angular momentum l of a particle, whose position vector is r with components x, y, z and momentum is p with components px, py and 'p_z`. Show that if the particle moves only in the x-y plane the angular momentum has only a z-component.
The torque of a force \[\overrightarrow F \] about a point is defined as \[\overrightarrow\Gamma = \overrightarrow r \times \overrightarrow F.\] Suppose \[\overrightarrow r, \overrightarrow F\] and \[\overrightarrow \Gamma\] are all nonzero. Is \[r \times \overrightarrow\Gamma || \overrightarrow F\] always true? Is it ever true?
A heavy particle of mass m falls freely near the earth's surface. What is the torque acting on this particle about a point 50 cm east to the line of motion? Does this torque produce any angular acceleration in the particle?
If the resultant torque of all the forces acting on a body is zero about a point, is it necessary that it will be zero about any other point?
A rectangular brick is kept on a table with a part of its length projecting out. It remains at rest if the length projected is slightly less than half the total length but it falls down if the length projected is slightly more than half the total length. Give reason.
When a body is weighed on an ordinary balance we demand that the arum should be horizontal if the weights on the two pans are equal. Suppose equal weights are put on the two pans, the arm is kept at an angle with the horizontal and released. Is the torque of the two weights about the middle point (point of support) zero? Is the total torque zero? If so, why does the arm rotate and finally become horizontal?
Equal torques act on the disc A and B of the previous problem, initially both being at rest. At a later instant, the linear speeds of a point on the rim of A and another point on the rim of B are \[\nu_A\] and \[\nu_B\] respectively. We have
The density of a rod gradually decreases from one end to the other. It is pivoted at an end so that it can move about a vertical axis though the pivot. A horizontal force F is applied on the free end in a direction perpendicular to the rod. The quantities, that do not depend on which end of the rod is pivoted, are ________________ .
Calculate the total torque acting on the body shown in the following figure about the point O.

Define torque and mention its unit.
State conservation of angular momentum.
A particle of mass 5 units is moving with a uniform speed of v = `3sqrt 2` units in the XOY plane along the line y = x + 4. Find the magnitude of angular momentum
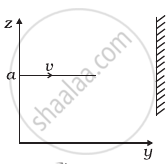
A particle of mass m is moving in yz-plane with a uniform velocity v with its trajectory running parallel to + ve y-axis and intersecting z-axis at z = a (Figure). The change in its angular momentum about the origin as it bounces elastically from a wall at y = constant is ______.
The net external torque on a system of particles about an axis is zero. Which of the following are compatible with it?
- The forces may be acting radially from a point on the axis.
- The forces may be acting on the axis of rotation.
- The forces may be acting parallel to the axis of rotation.
- The torque caused by some forces may be equal and opposite to that caused by other forces.
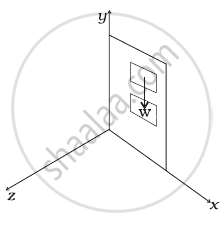
A door is hinged at one end and is free to rotate about a vertical axis (Figure). Does its weight cause any torque about this axis? Give reason for your answer.
A particle of mass 'm' is moving in time 't' on a trajectory given by
`vecr = 10alphat^2hati + 5beta(t - 5)hatj`
Where α and β are dimensional constants.
The angular momentum of the particle becomes the same as it was for t = 0 at time t = ______ seconds.
Angular momentum of a single particle moving with constant speed along the circular path ______.
A solid sphere is rotating in free space. If the radius of the sphere is increased while keeping the mass the same, which one of the following will not be affected?
