Advertisements
Advertisements
प्रश्न
Evaluate the following :
`lim_(x -> 1) [(x + 3x^2 + 5x^3 + ... + (2"n" - 1)x^"n" - "n"^2)/(x - 1)]`
उत्तर
`lim_(x -> 1) [(x + 3x^2 + 5x^3 + ... + (2"n" - 1)x^"n" - "n"^2)/(x - 1)]`
1 + 3 + 5 + … + (2n – 1)
= `sum_("r" = 1)^"n" (2"r" - 1)`
= `2 sum_("r" = 1)^"n" "r" - sum_("r" = 1)^"n" 1`
= `2("n"("n" + 1))/2 - "n"`
= n(n + 1) – n
= n2 + n – n
= n2
∴ n2 = 1 + 3 + 5 + … + (2n – 1).
∴ Required limit
= `lim_(x -> 1) ([ x + 3x^2 + 5x^3 + ... + (2"n" - 1)x^"n"] - [1 + 3 + 5 + ... + (2"n" - 1)])/(x - 1)`
= `lim_(x -> 1) ((x - 1) + (3x^2 - 3) + (5x^2 - 5) + ... + (2"n" - 1)x^"n" - (2"n" - 1))/(x - 1)`
= `lim_(x -> 1) [(x - 1)/(x - 1) + (3(x^2 - 1))/(x - 1) + (5(x^3 - 1))/(x - 1) + ... + ((2"n" - 1)(x^"n" - 1))/(x - 1)]`
= `lim_(x -> 1) ((x^1 - 1^1)/(x - 1)) + 3lim_(x -> 1) ((x^2 - 1^2)/(x - 1)) + 5lim_(x -> 1)((x^3 - 1^3)/(x - 1)) + ... + (2"n" - 1) lim_(x -> 1) ((x^"n" - 1^"n")/(x - 1))`
= 1(1)0 + 3(2)(1)1 + 5(3)(1)2 + … + (2n – 1) n(1)n–1
= 1(1) + 3(2) + 5(3) + … + (2n – 1)n
= `sum_("r" = 1)^"n" (2"r" - 1)"r"`
= `sum_("r" = 1)^"n" (2"r"^2 - "r")`
= `2 sum_("r" = 1)^"n" "r"^2 - sum_("r" = 1)^"n" "r"`
= `2* ("n"("n" + 1)(2"n" + 1))/6 - ("n"("n" + 1))/2`
= `"n"("n" + 1)((2"n" + 1)/3 - 1/2)`
= `"n"("n" + 1) ((4"n" + 2- 3)/6)`
= `("n"("n" + 1) (4"n" - 1))/6`
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
`lim_(y -> -3) [(y^5 + 243)/(y^3 + 27)]`
Evaluate the following limit:
`lim_(x -> 3)[sqrt(2x + 6)/x]`
Evaluate the following limit :
If `lim_(x -> 5) [(x^"k" - 5^"k")/(x - 5)]` = 500, find all possible values of k.
Evaluate the following limit :
`lim_(y -> 1)[(2y - 2)/(root(3)(7 + y) - 2)]`
In the following example, given ∈ > 0, find a δ > 0 such that whenever, |x – a| < δ, we must have |f(x) – l| < ∈.
`lim_(x -> -3) (3x + 2)` = – 7
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (cos x - 1)/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.0001 | 0.01 | 0.1 |
| f(x) | 0.04995 | 0.0049999 | 0.0004999 | – 0.0004999 | – 0.004999 | – 0.04995 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 0) sec x`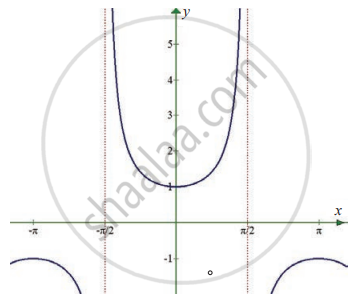
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> x/2) tan x`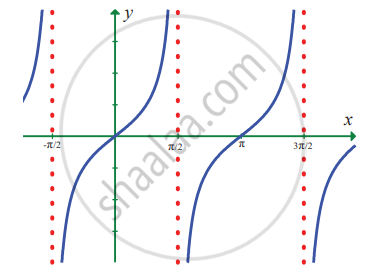
Sketch the graph of f, then identify the values of x0 for which `lim_(x -> x_0)` f(x) exists.
f(x) = `{{:(x^2",", x ≤ 2),(8 - 2x",", 2 < x < 4),(4",", x ≥ 4):}`
If f(2) = 4, can you conclude anything about the limit of f(x) as x approaches 2?
Verify the existence of `lim_(x -> 1) f(x)`, where `f(x) = {{:((|x - 1|)/(x - 1)",", "for" x ≠ 1),(0",", "for" x = 1):}`
Evaluate the following limits:
`lim_(x -> 2) (x^4 - 16)/(x - 2)`
Evaluate the following limits:
`lim_(sqrt(x) -> 3) (x^2 - 81)/(sqrt(x) - 3)`
Evaluate the following limits:
`lim_("h" -> 0) (sqrt(x + "h") - sqrt(x))/"h", x > 0`
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x + 4) - 3)/(x - 5)`
Evaluate the following limits:
`lim_(x -> 1) (sqrt(x) - x^2)/(1 - sqrt(x))`
Evaluate the following limits:
`lim_(x -> 1) (root(3)(7 + x^3) - sqrt(3 + x^2))/(x - 1)`
Evaluate the following limits:
`lim_(x -> "a") (sqrt(x - "b") - sqrt("a" - "b"))/(x^2 - "a"^2) ("a" > "b")`
Find the left and right limits of f(x) = `(x^2 - 4)/((x^2 + 4x+ 4)(x + 3))` at x = – 2
Find the left and right limits of f(x) = tan x at x = `pi/2`
Evaluate the following limits:
`lim_(x -> oo) (x^4 - 5x)/(x^2 - 3x + 1)`
Evaluate the following limits:
`lim_(x ->oo) (x^3/(2x^2 - 1) - x^2/(2x + 1))`
Show that `lim_("n" -> oo) (1^2 + 2^2 + ... + (3"n")^2)/((1 + 2 + ... + 5"n")(2"n" + 3)) = 9/25`
Evaluate the following limits:
`lim_(x -> oo)(1 + 1/x)^(7x)`
Evaluate the following limits:
`lim_(x -> oo) (1 + 3/x)^(x + 2)`
Evaluate the following limits:
`lim_(x -> 0) (3^x - 1)/(sqrt(x + 1) - 1)`
Evaluate the following limits:
`lim_(x -> pi) (sin3x)/(sin2x)`
Evaluate the following limits:
`lim_(x -> 0) ("e"^("a"x) - "e"^("b"x))/x`
Evaluate the following limits:
`lim_(x -> ) (sinx(1 - cosx))/x^3`
Evaluate the following limits:
`lim_(x -> 0) (tan x - sin x)/x^3`
Choose the correct alternative:
`lim_(x -> oo) sinx/x`
Choose the correct alternative:
`lim_(x -> 0) (x"e"^x - sin x)/x` is
Choose the correct alternative:
`lim_(x -> 0) ("e"^(sin x) - 1)/x` =
Choose the correct alternative:
The value of `lim_(x -> 0) sinx/sqrt(x^2)` is
`lim_(x -> 0) ((2 + x)^5 - 2)/((2 + x)^3 - 2)` = ______.
`lim_(x -> 5) |x - 5|/(x - 5)` = ______.
The value of `lim_(x→0)(sin(ℓn e^x))^2/((e^(tan^2x) - 1))` is ______.
