Advertisements
Advertisements
प्रश्न
Let f: R → R be the functions defined by f(x) = x3 + 5. Then f–1(x) is ______.
विकल्प
`(x + 5)^(1/3)`
`(x - 5)^(1/3)`
`(5 - x)^(1/3)`
5 – x
उत्तर
Let f: R → R be the functions defined by f(x) = x3 + 5. Then f–1(x) is `(x - 5)^(1/3)`.
Explanation:
We have, f(x) = x3 + 5 = y ....(Let)
⇒ y = x3 + 5
⇒ x3 = y – 5
⇒ x = `(y - 5)^(1/3)`
⇒ f–1(x) = `(x - 5)^(1/3)`
APPEARS IN
संबंधित प्रश्न
Check the injectivity and surjectivity of the following function:
f: N → N given by f(x) = x2
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f: R → R defined by f(x) = 1 + x2
Give examples of two functions f: N → Z and g: Z → Z such that g o f is injective but gis not injective.
(Hint: Consider f(x) = x and g(x) =|x|)
Let A = {−1, 0, 1, 2}, B = {−4, −2, 0, 2} and f, g: A → B be functions defined by f(x) = x2 − x, x ∈ A and g(x) = `2|x - 1/2|- 1, x in A`. Are f and g equal?
Justify your answer. (Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) ∀ a ∈ A are called equal functions).
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 3 − 4x
Let f : N → N be defined by
`f(n) = { (n+ 1, if n is odd),( n-1 , if n is even):}`
Show that f is a bijection.
[CBSE 2012, NCERT]
Find gof and fog when f : R → R and g : R → R is defined by f(x) = x2 + 8 and g(x) = 3x3 + 1 .
Let f = {(3, 1), (9, 3), (12, 4)} and g = {(1, 3), (3, 3) (4, 9) (5, 9)}. Show that gof and fog are both defined. Also, find fog and gof.
Find fog and gof if : f (x) = x+1, g(x) = `e^x`
.
Find fog and gof if : f (x) = x+1, g (x) = sin x .
Let f be any real function and let g be a function given by g(x) = 2x. Prove that gof = f + f.
Let \[f : \left( - \frac{\pi}{2}, \frac{\pi}{2} \right) \to R\] be a function defined by f(x) = cos [x]. Write range (f).
Let `f : R - {- 3/5}` → R be a function defined as `f (x) = (2x)/(5x +3).`
f-1 : Range of f → `R -{-3/5}`.
Let f : R → R, g : R → R be two functions defined by f(x) = x2 + x + 1 and g(x) = 1 − x2. Write fog (−2).
The function
f : A → B defined by
f (x) = - x2 + 6x - 8 is a bijection if
Let
f : R → R be given by
\[f\left( x \right) = \left[ x^2 \right] + \left[ x + 1 \right] - 3\]
where [x] denotes the greatest integer less than or equal to x. Then, f(x) is
(d) one-one and onto
Let f be an injective map with domain {x, y, z} and range {1, 2, 3}, such that exactly one of the following statements is correct and the remaining are false.
\[f\left( x \right) = 1, f\left( y \right) \neq 1, f\left( z \right) \neq 2 .\]
The value of
\[f^{- 1} \left( 1 \right)\] is
Let
\[f : R \to R\] be a function defined by
\[f : R \to R\] is defined by
\[f\left( x \right) = \frac{e^{x^2} - e^{- x^2}}{e^{x^2 + e^{- x^2}}} is\]
The function
Let \[f\left( x \right) = \frac{1}{1 - x} . \text{Then}, \left\{ f o \left( fof \right) \right\} \left( x \right)\]
If \[f : R \to \left( - 1, 1 \right)\] is defined by
\[f\left( x \right) = \frac{- x|x|}{1 + x^2}, \text{ then } f^{- 1} \left( x \right)\] equals
Which function is used to check whether a character is alphanumeric or not?
Let R be the set of real numbers and f: R → R be the function defined by f(x) = 4x + 5. Show that f is invertible and find f–1.
Let the function f: R → R be defined by f(x) = cosx, ∀ x ∈ R. Show that f is neither one-one nor onto
If A = {x ∈ R: |x – 2| > 1}, B = `{x ∈ R : sqrt(x^2 - 3) > 1}`, C = {x ∈ R : |x – 4| ≥ 2} and Z is the set of all integers, then the number of subsets of the set (A ∩ B ∩ C) C ∩ Z is ______.
Let f(x) be a polynomial of degree 3 such that f(k) = `-2/k` for k = 2, 3, 4, 5. Then the value of 52 – 10f(10) is equal to ______.
For x ∈ R, x ≠ 0, let f0(x) = `1/(1 - x)` and fn+1 (x) = f0(fn(x)), n = 0, 1, 2, .... Then the value of `f_100(3) + f_1(2/3) + f_2(3/2)` is equal to ______.
Let f(x) be a polynomial function of degree 6 such that `d/dx (f(x))` = (x – 1)3 (x – 3)2, then
Assertion (A): f(x) has a minimum at x = 1.
Reason (R): When `d/dx (f(x)) < 0, ∀ x ∈ (a - h, a)` and `d/dx (f(x)) > 0, ∀ x ∈ (a, a + h)`; where 'h' is an infinitesimally small positive quantity, then f(x) has a minimum at x = a, provided f(x) is continuous at x = a.
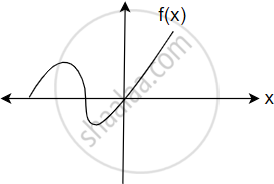
The given function f : R → R is not ‘onto’ function. Give reason.
