Advertisements
Advertisements
प्रश्न
The decomposition of N2O5(g) at 320K according to the following equation follows first order reaction:
`N_2O_(5(g))->2NO_(2(g))+1/2O_(2(g))`
The initial concentration of N2O5(g) is 1.24 x 10-2 mol. L-1 and after 60 minutes 0.20x10-2 molL-1. Calculate the rate constant of the reaction at 320K.
उत्तर
Data: `N_2O_(5(g))->2NO_(2(g))+1/2O_2`
`[N_2O_5]_@=1.24xx10^-2molL^-1`
`[N_2O_5]_t=0.20xx10^-2molL^-1`
k=? t=60min
Solution :
`k=2.303/t log""([N_2O_5])/([N_2O_5])`
`k=2.303/60log""((1.24xx10^-2))/((0.2xx10^-2))`
k=0.0383x0.7924
k= 0.303min-1
APPEARS IN
संबंधित प्रश्न
What is pseudo first order reaction? Give one· example of it.
Write molecularity of the following reaction:
2NO(g)+O2(g)→2NO2(g)
Write two factors that affect the rate of reaction.
For a reaction A + B ⟶ P, the rate is given by
Rate = k [A] [B]2
What is the overall order of reaction if A is present in large excess?
For a reaction : 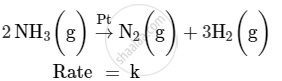
(i) Write the order and molecularity of this reaction.
(ii) Write the unit of k.
For a chemical reaction R → P, the variation in the concentration (R) vs. time (t) plot is given as:
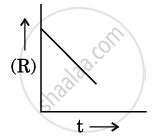
(i) Predict the order of the reaction.
(ii) What is the slope of the curve ?
(iii) Write the unit of rate constant for this reaction.
The following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume :
SO2Cl2 (g) → SO2 (g) + Cl2 (g)
| Experiment | Time/s–1 | Total pressure/atm |
| 1 | 0 | 0.4 |
| 2 | 100 | 0.7 |
Calculate the rate constant.
(Given : log 4 = 0.6021, log 2 = 0.3010)
From the rate expression for the following reaction, determine the order of reaction and the dimension of the rate constant.
\[\ce{H2O2_{( aq)} + 3I^-_{( aq)} + 2H^+ -> 2H2O_{(l)} + I^-_3}\] Rate = k[H2O2][I−]
From the rate expression for the following reaction, determine the order of reaction and the dimension of the rate constant.
\[\ce{C2H5Cl_{(g)} -> C2H4_{(g)} + HCl_{(g)}}\] Rate = k [C2H5Cl]
A reaction is first order in A and second order in B. Write the differential rate equation.
A reaction is first order in A and second order in B. How is the rate affected on increasing the concentration of B three times?
In a reaction between A and B, the initial rate of reaction (r0) was measured for different initial concentrations of A and B as given below:
| A/mol L−1 | 0.20 | 0.20 | 0.40 |
| B/mol L−1 | 0.30 | 0.10 | 0.05 |
| r0/mol L−1 s−1 | 5.07 × 10−5 | 5.07 × 10−5 | 1.43 × 10−4 |
What is the order of the reaction with respect to A and B?
Define the following terms:
Pseudo first-order reaction
What is the order of a reaction which has a rate expression; Rate = `"k"["A"]^(3/2)["B"]^1`?
Molecularity of a reaction _____________.
Compounds ‘A’ and ‘B’ react according to the following chemical equation.
\[\ce{A(g) + 2B(g) -> 2C(g)}\]
Concentration of either ‘A’ or ‘B’ were changed keeping the concentrations of one of the reactants constant and rates were measured as a function of initial concentration. Following results were obtained. Choose the correct option for the rate equations for this reaction.
| Experiment | Initial concentration of [A]/mol L–¹ |
Initial concentration of [B]/mol L–¹ |
Initial rate of formation of [C]/mol L–¹ s–¹ |
| 1. | 0.30 | 0.30 | 0.10 |
| 2. | 0.30 | 0.60 | 0.40 |
| 3. | 0.60 | 0.30 | 0.20 |
In a reaction if the concentration of reactant A is tripled, the rate of reaction becomes twenty seven times. What is the order of the reaction?
Why does the rate of any reaction generally decreases during the course of the reaction?
Why can’t molecularity of any reaction be equal to zero?
Why molecularity is applicable only for elementary reactions and order is applicable for elementary as well as complex reactions?
Assertion: Order of the reaction can be zero or fractional.
Reason: We cannot determine order from balanced chemical equation.
Use Molecular Orbital theory to determine the bond order in each of species, [He2j+ and [He2]2+?
The rate of a chemical reaction double for every 10° rise in temperature. If the temperature is raised. by 50°C, the rate of relation by about:-
If the 0.05 molar solution of m+ is replaced by a 0.0025 molar m+ solution, then the magnitude of the cell potential would be
The half-life period of a. substance in a certain enzyme catalysed reaction is 138 s. The time required for the concentration of the substance to fall from 1.28 mol–1 to 0.04 mg L–1 is
A reaction is second order with respect to a reactant. How is the rate of reaction affected if the concentration of the reactant is reduced to half?
