Question

If sec `theta = 17/8 ` verify that `((3-4sin^2theta)/(4 cos^2theta -3))=((3-tan^2theta)/(1-tan^2theta))`

Answer 1

It is given that sec 𝜃`=17/8` 

Let us consider a right ΔABC right angled at B and ∠𝐶 = 𝜃
We know that cos 𝜃 =`1/sectheta = 8/17 =(BC)/(AC)`

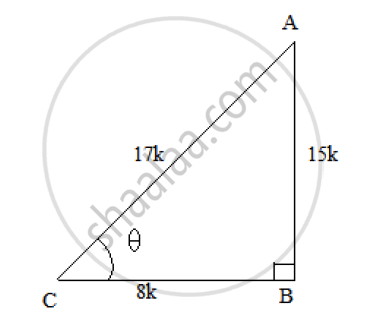
So, if BC = 8k, then AC = 17k, where k is a positive number.
Using Pythagoras theorem, we have:
`AC^2 = AB^2 + BC^2`
`⟹ AB^2 = AC^2 − BC^2 = (17K)^2 − (8K)^2`
`⟹ AB^2 = 289K^2 − 64K^2 = 225K^2`
⟹AB = 15k.
Now, tan 𝜃 =`(AB)/(BC) =15/8 and sin theta =(AB)/(AC) =(15K)/(17K)=15/17`

The given expression is `(3-4 sin^2theta)/(4cos^2 theta-3) = (3-tan^2theta)/(1-3tan^2theta)`

Substituting the values in the above expression, we get:

LHS=`(3-4(15/17)^2)/(4(8/17)^2-3)`

=`(3-(900/289))/((250/289)-3)`

=`(867-900)/(256-867)=-33/-611 =33/611`

RHS = `(3-(15/8)^2)/(1-3(15/8)^2)`

 = `(3-225/64)/(1-675/64)`

= `(192-255)/(64-675)=-33/-611 = 33/611`
∴ LHS = RHS
Hence proved.