Advertisements
Advertisements
प्रश्न
In the Arrhenius equation for a first order reaction, the values of ‘A’ of ‘Ea’ are 4 x 1013 sec-1 and 98.6 kJ mol-1 respectively. At what temperature will its half life period be 10 minutes?
[R = 8.314 J K-1 mol-1]
उत्तर
Given: Frequency factor (A) = 4 x 1013 sec–1
Energy of activation (Ea) = 98.6 kJ mol–1
Half life period (t1/2) = 10 minutes = 10 x 60 = 600 sec.
`-> k=0.693/t_(1/2)`
`k=0.693/600=11.55xx10^-4 sec^-1`
`log_10k=log_10A-E_a/(2.303RT)`
`log_10 11.55 xx10^-4 =log_10 4xx10^13-(98.6xx10^3)/(2.303xx8.314xxT)`
`-2.937=13.602-(98.6xx10^3)/(2.303xx8.314xxT)`
`-2.937=13.602-(5149.632)/T`
`(5149.632)/T=13.602+2.937`
`T=(5149.632)/16.539`
T=311.36K or 38.36°
APPEARS IN
संबंधित प्रश्न
Explain a graphical method to determine activation energy of a reaction.
(b) Rate constant ‘k’ of a reaction varies with temperature ‘T’ according to the equation:
`logk=logA-E_a/2.303R(1/T)`
Where Ea is the activation energy. When a graph is plotted for `logk Vs. 1/T` a straight line with a slope of −4250 K is obtained. Calculate ‘Ea’ for the reaction.(R = 8.314 JK−1 mol−1)
The rate constant for the first-order decomposition of H2O2 is given by the following equation:
`logk=14.2-(1.0xx10^4)/TK`
Calculate Ea for this reaction and rate constant k if its half-life period be 200 minutes.
(Given: R = 8.314 JK–1 mol–1)
The rate of the chemical reaction doubles for an increase of 10 K in absolute temperature from 298 K. Calculate Ea.
The rate constant for the decomposition of hydrocarbons is 2.418 × 10−5 s−1 at 546 K. If the energy of activation is 179.9 kJ/mol, what will be the value of pre-exponential factor?
The decomposition of A into product has value of k as 4.5 × 103 s−1 at 10°C and energy of activation 60 kJ mol−1. At what temperature would k be 1.5 × 104 s−1?
The rate constant of a first order reaction are 0.58 S-1 at 313 K and 0.045 S-1 at 293 K. What is the energy of activation for the reaction?
What is the effect of adding a catalyst on Activation energy (Ea)
A first-order reaction is 50% completed in 40 minutes at 300 K and in 20 minutes at 320 K. Calculate the activation energy of the reaction. (Given : log 2 = 0·3010, log 4 = 0·6021, R = 8·314 JK–1 mol–1)
The decomposition of a hydrocarbon has value of rate constant as 2.5×104s-1 At 27° what temperature would rate constant be 7.5×104 × 3 s-1if energy of activation is 19.147 × 103 J mol-1 ?
The rate of chemical reaction becomes double for every 10° rise in temperature because of ____________.
Activation energy of a chemical reaction can be determined by ______.
Consider figure and mark the correct option.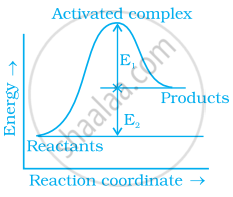
During decomposition of an activated complex:
(i) energy is always released
(ii) energy is always absorbed
(iii) energy does not change
(iv) reactants may be formed
Why does the rate of a reaction increase with rise in temperature?
Thermodynamic feasibility of the reaction alone cannot decide the rate of the reaction. Explain with the help of one example.
Match the statements given in Column I and Column II
| Column I | Column I | |
| (i) | Catalyst alters the rate of reaction | (a) cannot be fraction or zero |
| (ii) | Molecularity | (b) proper orientation is not there always |
| (iii) | Second half life of first order reaction | (c) by lowering the activation energy |
| (iv) | `e^((-E_a)/(RT)` | (d) is same as the first |
| (v) | Energetically favourable reactions (e) total probability is one are sometimes slow | (e) total probability is one |
| (vi) | Area under the Maxwell Boltzman curve is constant | (f) refers to the fraction of molecules with energy equal to or greater than activation energy |
What happens to most probable kinetic energy and the energy of activation with increase in temperature?
In respect of the eqn k = \[\ce{Ae^{{-E_a}/{RT}}}\] in chemical kinetics, which one of the following statement is correct?
The rate constant for a reaction is 1.5 × 10–7 sec–1 at 50°C. What is the value of activation energy?
The activation energy in a chemical reaction is defined as ______.
The activation energy in a chemical reaction is defined as ______.
A schematic plot of ln Keq versus inverse of temperature for a reaction is shown below
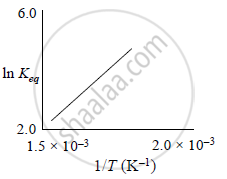
The reaction must be:
A first-order reaction is 50% complete in 30 minutes at 300 K and in 10 minutes at 320 K. Calculate activation energy (Ea) for the reaction. [R = 8.314 J K−1 mol−1]
[Given: log 2 = 0.3010, log 3 = 0.4771, log 4 = 0.6021]
What happens to the rate constant k and activation energy Ea as the temperature of a chemical reaction is increased? Justify.
