Question

Find the area of the region bounded by y = | x − 1 | and y = 1.

Answer 1

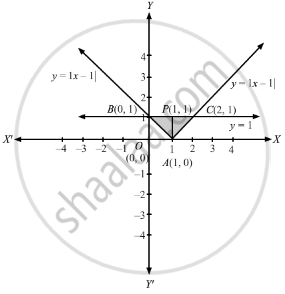
We have,
\[y = \left| x - 1 \right|\]
\[ \Rightarrow y = \begin{cases}x - 1& x \geq 1\\1 - x& x < 1\end{cases}\]
y = x − 1 is a straight line originating from A(1, 0)  and making an angle 45^o with the x-axis
y = 1 − x is a straight line originating from A(1, 0)  and making an angle 135^o with the x-axis

y = x is a straight line parallel to x-axis and passing through B(0, 1)

The point of intersection of  two lines with y = 1 is obtained by solving the simultaneous equations
\[y = 1\]
\[\text{ and }y = x - 1 \]
\[ \Rightarrow 1 = x - 1\]
\[ \Rightarrow x - 2 = 0\]
\[ \Rightarrow x = 2\]
\[ \Rightarrow C\left( 2, 1 \right)\text{ is point of intersection of }y = x - 1\text{ and }y = 1\]
\[y = 1\text{ and }y = 1 - x\]
\[ \Rightarrow 1 = 1 - x\]
\[ \Rightarrow x = 0\]
\[ \Rightarrow B\left( 0, 1 \right)\text{ is point of intersection of }y = 1 - x\text{ and }y = 1\]
\[\text{ Since }y = \left| x - 1 \right|\text{ changes character at A }(1, 0) ,\text{ Consider point P }(1, 1)\text{ on BC such that PA is perpendicular to }x -\text{ axis }. \]
\[\text{ Required shaded area }\left( ABCA \right) =\text{ area }\left( ABPA \right) + \text{ area }\left( PCAP \right)\]
\[ = \int_0^1 \left[ 1 - \left( 1 - x \right) \right]dx + \int_1^2 \left[ 1 - \left( x - 1 \right) \right]dx\]
\[ = \int_0^1 x dx + \int_1^2 \left( 2 - x \right) dx\]
\[ = \left[ \frac{x^2}{2} \right]_0^1 + \left[ 2x - \frac{x^2}{2} \right]_1^2 \]
\[ = \frac{1}{2} + \left[ 4 - 2 - 2 + \frac{1}{2} \right]\]
\[ = \frac{1}{2} + \frac{1}{2} = 1\text{ sq . unit }\]