Question

Find the area of the region enclosed by the parabola x² = y and the line y = x + 2.

Answer 1

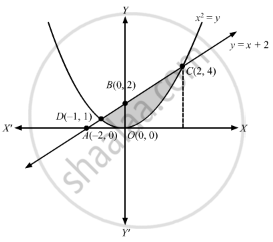
 The points of intersection C and D are obtained by solving the two equations
\[\therefore x^2 = x + 2\]
\[ \Rightarrow x^2 - x - 2 = 0\]
\[ \Rightarrow \left( x - 2 \right)\left( x + 1 \right) = 0\]
\[ \Rightarrow x = 2\text{ or }x = - 1\]
\[ \Rightarrow y = 2^2 = 4\text{ or }y = \left( - 1 \right)^2 = 1\]
\[\text{ Thus }C(\left( 2, 4 \right)\text{ and }D\left( - 1, 1 \right) \text{ are the points of intesection of two curves }\]
\[\text{ Consider a vertical steip of length }\left| y_2 - y_1 \right| \text{ and width dx where }P\left( x, y_2 \right)\text{ lies on straight line and }Q\left( x, y_1 \right)\text{ lies on the parabola }. \]
\[\text{ Area of approximating rectangle }= \left| y_2 - y_1 \right| dx ,\text{ and it moves from }x = - 1\text{ to }x = 2\]
\[\text{ Required area = area }\left( ODBCO \right) = \int_{- 1}^2 \left| y_2 - y_1 \right| dx\]
\[ = \int_{- 1}^2 \left( y_2 - y_1 \right) dx .............\left\{ \because \left| y_2 - y_1 \right| = y_2 - y_1 as y_2 > y_1 \right\}\]
\[ = \int_{- 1}^2 \left( \left( x + 2 \right) - x^2 \right) dx\]
\[ = \int_{- 1}^2 \left( x + 2 - x^2 \right) dx\]
\[ = \left[ \frac{x^2}{2} + 2x - \frac{x^3}{3} \right]_{- 1}^2 \]
\[ = \frac{4}{2} + 4 - \frac{8}{3} - \frac{1}{2} + 2 - \frac{1}{3}\]
\[ = \frac{9}{2}\text{ sq units }\]
\[\text{ Area enclosed by the line and given parabola }= \frac{9}{2}\text{ sq units }\]