Advertisements
Advertisements
Question
Find the value of x, if tan `[sec^(-1) (1/x) ] = sin ( tan^(-1) 2) , x > 0 `.
Solution
Let sec-1 `(1/x) = theta`
` ⇒ sec theta = 1/x`
⇒ cos θ = x
⇒ tan ` (sec^(-1) (1/x)) = tan theta = sqrt(1 -x^2 ) /x ` ...(1)
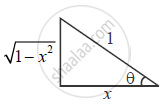
Now consider,
sin ( tan -1 2 )
Let tan-1 2 = Φ
tan Φ = 2
sin ( tan-1 2) = sin Φ = `2/sqrt(5) ` ...(ii)
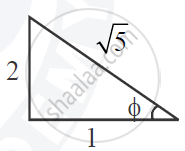
From (i) and (ii)
`sqrt(1- x^2 )/x = 2/sqrt(5)`
5(1 - x2 ) = 4x2
`x = +- sqrt(5)/3 " but " x > 0 ⇒ x = sqrt(5)/3`
APPEARS IN
RELATED QUESTIONS
Write the value of `tan(2tan^(-1)(1/5))`
Solve the equation for x:sin−1x+sin−1(1−x)=cos−1x
Solve the following for x:
`sin^(-1)(1-x)-2sin^-1 x=pi/2`
`sin^-1(sin4)`
Evaluate the following:
`sec^-1(sec (25pi)/6)`
Evaluate the following:
`cosec^-1(cosec (6pi)/5)`
Evaluate the following:
`cot^-1(cot (4pi)/3)`
Evaluate the following:
`cot^-1(cot (9pi)/4)`
Write the following in the simplest form:
`tan^-1(x/(a+sqrt(a^2-x^2))),-a<x<a`
Write the following in the simplest form:
`sin^-1{(x+sqrt(1-x^2))/sqrt2},-1<x<1`
Evaluate the following:
`cot(cos^-1 3/5)`
Evaluate:
`cot(sin^-1 3/4+sec^-1 4/3)`
Evaluate:
`sin(tan^-1x+tan^-1 1/x)` for x > 0
Evaluate:
`cos(sec^-1x+\text(cosec)^-1x)`,|x|≥1
`5tan^-1x+3cot^-1x=2x`
Prove the following result:
`tan^-1 1/4+tan^-1 2/9=sin^-1 1/sqrt5`
Find the value of `tan^-1 (x/y)-tan^-1((x-y)/(x+y))`
Solve the following equation for x:
`tan^-1((x-2)/(x-4))+tan^-1((x+2)/(x+4))=pi/4`
Solve the following:
`sin^-1x+sin^-1 2x=pi/3`
Solve the following equation for x:
`cos^-1((x^2-1)/(x^2+1))+1/2tan^-1((2x)/(1-x^2))=(2x)/3`
Write the value of
\[\cos^{- 1} \left( \frac{1}{2} \right) + 2 \sin^{- 1} \left( \frac{1}{2} \right)\].
Write the range of tan−1 x.
Write the value of cos−1 \[\left( \cos\frac{5\pi}{4} \right)\]
Find the value of \[2 \sec^{- 1} 2 + \sin^{- 1} \left( \frac{1}{2} \right)\]
The positive integral solution of the equation
\[\tan^{- 1} x + \cos^{- 1} \frac{y}{\sqrt{1 + y^2}} = \sin^{- 1} \frac{3}{\sqrt{10}}\text{ is }\]
Let f (x) = `e^(cos^-1){sin(x+pi/3}.`
Then, f (8π/9) =
The value of \[\sin^{- 1} \left( \cos\frac{33\pi}{5} \right)\] is
If \[\sin^{- 1} \left( \frac{2a}{1 - a^2} \right) + \cos^{- 1} \left( \frac{1 - a^2}{1 + a^2} \right) = \tan^{- 1} \left( \frac{2x}{1 - x^2} \right),\text{ where }a, x \in \left( 0, 1 \right)\] , then, the value of x is
If 2 tan−1 (cos θ) = tan−1 (2 cosec θ), (θ ≠ 0), then find the value of θ.
The value of sin `["cos"^-1 (7/25)]` is ____________.
