Advertisements
Advertisements
Question
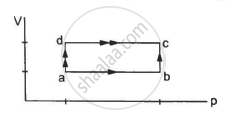
When a system is taken through the process abc shown in figure, 80 J of heat is absorbed by the system and 30 J of work is done by it. If the system does 10 J of work during the process adc, how much heat flows into it during the process?
Solution
Initial point is a and the final point is c.
As internal energy is a state function so it depends only on the initial and final points and not on the path followed by the system. This implies that change in internal energy for path abc and path adc is the same.
Using the first law of thermodynamics, we get
∆Q = ∆U + ∆W
Here, ∆Q is the amount of heat absorbed and ∆U is the change in internal energy of the system. Also, ∆W is the work done by the system.
For path abc:-
∆Q = 80 J, ∆W = 30 J
∆U = (80 − 30) J = 50 J
For path abc:-
∆U =50 J ..............(same as for path abc)
∆W = 10 J
∴ ∆Q = 10 J + 50 J = 60 J ..........(∆U = 50 J)
APPEARS IN
RELATED QUESTIONS
An electric heater supplies heat to a system at a rate of 100W. If the system performs work at a rate of 75 Joules per second. At what rate is the internal energy increasing?
Write the mathematical expression of the First Law of Thermodynamics for Isothermal Process
Write the mathematical expression of the First Law of Thermodynamics for the Isobaric process.
The first law of thermodynamics is a statement of ____________ .
A system can be taken from the initial state p1, V1 to the final state p2, V2 by two different methods. Let ∆Q and ∆W represent the heat given to the system and the work done by the system. Which of the following must be the same in both the methods?
Refer to figure. Let ∆U1 and ∆U2 be the change in internal energy in processes A and B respectively, ∆Q be the net heat given to the system in process A + B and ∆W be the net work done by the system in the process A + B.

(a) ∆U1 + ∆U2 = 0
(b) ∆U1 − ∆U2 = 0
(c) ∆Q − ∆W = 0
(d) ∆Q + ∆W = 0
The internal energy of a gas is given by U = 1.5 pV. It expands from 100 cm3 to 200 cm3against a constant pressure of 1.0 × 105 Pa. Calculate the heat absorbed by the gas in the process.
A gas is enclosed in a cylindrical vessel fitted with a frictionless piston. The gas is slowly heated for some time. During the process, 10 J of heat is supplied and the piston is found to move out 10 cm. Find the increase in the internal energy of the gas. The area of cross section of the cylinder = 4 cm2 and the atmospheric pressure = 100 kPa.
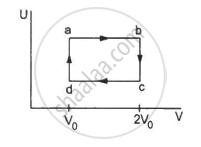
Figure shows the variation in the internal energy U with the volume V of 2.0 mol of an ideal gas in a cyclic process abcda. The temperatures of the gas at b and c are 500 K and 300 K respectively. Calculate the heat absorbed by the gas during the process.
Answer the following in one or two sentences.
State the first law of thermodynamics.
The process, in which no heat enters or leaves the system, is termed as ____________.
For a particular reaction, the system absorbs 8 kJ of heat and does 2.5 kJ of work on its surrounding. What will be the change in internal energy of the system?
A sample of gas absorbs 4000 kJ of heat and surrounding does 2000 J of work on sample, what is the value of ΔU?
Is it possible to increase the temperature of a gas without adding heat to it? Explain.
Air pressure in a car tyre increases during driving. Explain.
Consider a P-V diagram in which the path followed by one mole of perfect gas in a cylindrical container is shown in figure.

- Find the work done when the gas is taken from state 1 to state 2.
- What is the ratio of temperature T1/T2, if V2 = 2V1?
- Given the internal energy for one mole of gas at temperature T is (3/2) RT, find the heat supplied to the gas when it is taken from state 1 to 2, with V2 = 2V1.
A cycle followed by an engine (made of one mole of an ideal gas in a cylinder with a piston) is shown in figure. Find heat exchanged by the engine, with the surroundings for each section of the cycle. (Cv = (3/2)R)
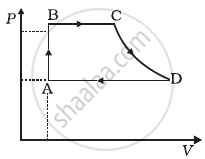
- AB : constant volume
- BC : constant pressure
- CD : adiabatic
- DA : constant pressure
Which among the following equations represents the first law of thermodynamics under isobaric conditions?
Define the isothermal process.
What is an isothermal process?
