ISC (Commerce)
ISC (Arts)
ISC (Science)
Academic Year: 2023-2024
Date & Time: 20th February 2024, 2:00 pm
Duration: 3h
Advertisements
Let L be a set of all straight lines in a plane. The relation R on L defined as 'perpendicular to' is ______.
Symmetric and Transitive
Transitive
Symmetric
Equivalence
Chapter: [0.01] Relations and Functions (Section A)
The order and degree of the differential equation `[1 + ((dy)/(dx))^2] = (d^2y)/(dx^2)` are ______.
`2, 3/2`
2, 3
2, 1
3, 4
Chapter: [0.034] Differential Equations
Let A be a non-empty set.
Statement 1: Identity relation on A is Reflexive.
Statement 2: Every Reflexive relation on A is an Identity relation.
Both the statements are true.
Both the statements are false.
Statement 1 is true and Statement 2 is false.
Statement 1 is false and Statement 2 is true.
Chapter: [0.01] Relations and Functions (Section A)
The graph of the function f is shown below.

Of the following options, at what values of x is the function f NOT differentiable?
At x = 0 and x = 2
At x = 1 and x = 3
At x = – 1 and x = 1
At x = – 1.5 and x = 1.5
Chapter: [0.031] Continuity, Differentiability and Differentiation
The value of cosec `[sin^-1((-1)/2)] - sec[cos^-1((-1)/2)]` is equal to ______.
–4
0
–1
4
Chapter: [0.01] Relations and Functions (Section A)
\[\frac{\pi}{2}\]
\[\frac{2\pi}{3}\]
Chapter: [0.033] Integrals
Assertion: Let the matrices A = `((-3, 2),(-5, 4))` and B = `((4, -2),(5, -3))` be such that A100B = BA100
Reason: AB = BA implies AB = BA for all positive integers n.
Both Assertion and Reason are true and Reason is the correct explanation for Assertion.
Both Assertion and Reason are true but Reason is not the correct explanation for Assertion.
Assertion is true and Reason is false.
Assertion is false and Reason is true.
Chapter: [0.021] Matrices and Determinants
If ∫(cot x – cosec2 x)ex dx = ex f(x) + c then f(x) will be ______.
cot x + cosec x
cot2 x
cot x
cosec x
Chapter: [0.033] Integrals
In which one of the following intervals is the function f(x) = x3 – 12x increasing?
(– 2, 2)
(– ∞, – 2) ∪ (2, ∞)
(– 2, ∞)
(– ∞, 2)
Chapter: [0.032] Applications of Derivatives
If A and B are symmetric matrices of the same order, then AB – BA is ______.
Skew – symmetric matrix
Symmetric matrix
Diagonal matrix
Identity matrix
Chapter: [0.021] Matrices and Determinants
Find the derivative of `y = log x + 1/x` with respect to x.
Chapter: [0.031] Continuity, Differentiability and Differentiation
Teena is practising for an upcoming Rifle Shooting tournament. The probability of her shooting the target in the 1st, 2nd, 3rd and 4th shots are 0.4, 0.3, 0.2 and 0.1 respectively. Find the probability of at least one shot of Teena hitting the target.
Chapter: [0.04] Probability (Section A)
Which one of the following graphs is a function of x?
 |
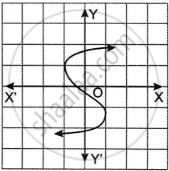 |
| Graph A | Graph B |
Chapter: [0.01] Relations and Functions (Section A)
Evaluate:
`int_0^6 |x + 3|dx`
Chapter: [0.033] Integrals
Given that `1/y + 1/x = 1/12` and y decreases at a rate of 1 cms–1, find the rate of change of x when x = 5 cm and y = 1 cm.
Chapter: [0.032] Applications of Derivatives
Let `f : R {(-1)/3} → R - {0}` be defined as `f(x) = 5/(3x + 1)` is invertible. Find f–1(x).
Chapter: [0.01] Relations and Functions (Section A)
If f : R `rightarrow` R is defined by `f(x) = (2x - 7)/4`, show that f(x) is one-one and onto.
Chapter: [0.01] Relations and Functions (Section A)
Find the value of the determinant given below, without expanding it at any stage.
`|(βγ, 1, α(β + γ)),(γα, 1, β(γ + α)),(αβ, 1, γ(α + β))|`
Chapter: [0.021] Matrices and Determinants
Determine the value of 'k' for which the following function is continuous at x = 3
`f(x) = {(((x + 3)^2 - 36)/(x - 3), x != 3), (k, x = 3):}`
Chapter: [0.031] Continuity, Differentiability and Differentiation
Advertisements
Find a point on the curve y = (x − 2)2 at which the tangent is parallel to the chord joining the points (2, 0) and (4, 4).
Chapter: [0.032] Applications of Derivatives
Evaluate: `int_0^(2π) (1)/(1 + e^(sin x)`dx
Chapter: [0.033] Integrals
Evaluate P(A ∪ B), if 2P(A) = P(B) = `5/13` and P(A | B) = `2/5`
Chapter: [0.04] Probability (Section A)
If y = 3 cos(log x) + 4 sin(log x), show that `x^2 (d^2y)/(dx^2) + x dy/dx + y = 0`
Chapter: [0.031] Continuity, Differentiability and Differentiation
Solve for x: `sin^-1(x/2) + cos^-1x = π/6`
Chapter: [0.01] Relations and Functions (Section A)
If sin–1x + sin–1y + sin–1z = π, show that `x^2 - y^2 - z^2 + 2yzsqrt(1 - x^2) = 0`
Chapter: [0.01] Relations and Functions (Section A)
Evaluate:
`int (x + 7)/(x^2 + 4x + 7)dx`
Chapter: [0.033] Integrals
A jewellery seller has precious gems in white and red colour which he has put in three boxes.
The distribution of these gems is shown in the table given below:
| Box | Number of Gems | |
| White | Red | |
| I | 1 | 2 |
| I | 2 | 3 |
| III | 3 | 1 |
He wants to gift two gems to his mother. So, he asks her to select one box at random and pick out any two gems one after the other without replacement from the selected box. The mother selects one white and one red gem.
Calculate the probability that the gems drawn are from Box II.
Chapter: [0.04] Probability (Section A)
A furniture factory uses three types of wood namely, teakwood, rosewood and satinwood for manufacturing three types of furniture, that are, table, chair and cot.
The wood requirements (in tonnes) for each type of furniture are given below:
| Table | Chair | Cot | |
| Teakwood | 2 | 3 | 4 |
| Rosewood | 1 | 1 | 2 |
| Satinwood | 3 | 2 | 1 |
It is found that 29 tonnes of teakwood, 13 tonnes of rosewood and 16 tonnes of satinwood are available to make all three types of furniture.
Using the above information, answer the following questions:
- Express the data given in the table above in the form of a set of simultaneous equations.
- Solve the set of simultaneous equations formed in subpart (i) by matrix method.
- Hence, find the number of table(s), chair(s) and cot(s) produced.
Chapter: [0.021] Matrices and Determinants
Mrs. Roy designs a window in her son’s study room so that the room gets maximum sunlight. She designs the window in the shape of a rectangle surmounted by an equilateral triangle. If the perimeter of the window is 12 m, find the dimensions of the window that will admit maximum sunlight into the room.
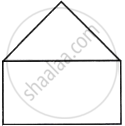
Chapter: [0.032] Applications of Derivatives
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
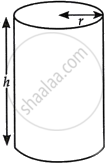
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.
Chapter: [0.032] Applications of Derivatives
Find the particular solution of the differential equation:
2y ex/y dx + (y - 2x ex/y) dy = 0 given that x = 0 when y = 1.
Chapter: [0.034] Differential Equations
For the following differential equation, find a particular solution satisfying the given condition:
\[x\left( x^2 - 1 \right)\frac{dy}{dx} = 1, y = 0\text{ when }x = 2\]
Chapter: [0.034] Differential Equations
A primary school teacher wants to teach the concept of 'larger number' to the students of Class II.
To teach this concept, he conducts an activity in his class. He asks the children to select two numbers from a set of numbers given as 2, 3, 4, 5 one after the other without replacement.
All the outcomes of this activity are tabulated in the form of ordered pairs given below:
| 2 | 3 | 4 | 5 | |
| 2 | (2, 2) | (2, 3) | (2, 4) | |
| 3 | (3, 2) | (3, 3) | (3, 5) | |
| 4 | (4, 2) | (4, 4) | (4, 5) | |
| 5 | (5, 3) | (5, 4) | (5, 5) |
- Complete the table given above.
- Find the total number of ordered pairs having one larger number.
- Let the random variable X denote the larger of two numbers in the ordered pair.
Now, complete the probability distribution table for X given below.
X 3 4 5 P(X = x) - Find the value of P(X < 5)
- Calculate the expected value of the probability distribution.
Chapter: [0.04] Probability (Section A)
If `veca = 3hati - 2hatj + hatk` and `vecb = 2hati - 4hatj - 3hatk` then the value of `|veca - 2vecb|` will be ______.
`sqrt(85)`
`sqrt(86)`
`sqrt(87)`
`sqrt(88)`
Chapter: [0.05] Vectors (Section B)
If a line makes an angle α, β and γ with positive direction of the coordinate axes, then the value of sin2α + sin2β + sin2γ will be ______.
1
3
–2
2
Chapter: [0.06] Three - Dimensional Geometry (Section B)
Advertisements
In the figure given below, if the coordinates of the point P are (a, b, c), then what are the perpendicular distances of P from XY, YZ and ZX planes respectively?
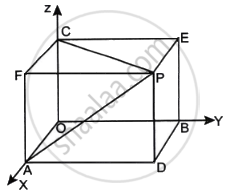
Chapter: [0.06] Three - Dimensional Geometry (Section B)
If `veca = 2hati + hatj + 2hatk` and `vecb = 5hati - 3hatj + hatk`, find the projection of `vecb` on `veca`.
Chapter: [0.05] Vectors (Section B)
Find a vector of magnitude 20 units parallel to the vector `2hati + 5hatj + 4hatk`.
Chapter: [0.05] Vectors (Section B)
If `veca xx vecb = veca xx vecc` where `veca, vecb` and `vecc` are non-zero vectors, then prove that either `vecb = vecc` or `veca` and `(vecb - vecc)` are parallel.
Chapter: [0.05] Vectors (Section B)
If `veca` and `vecb` are two non-zero vectors such that `|veca xx vecb| = veca.vecb`, find the angle between `veca` and `vecb`.
Chapter: [0.05] Vectors (Section B)
A mobile tower is situated at the top of a hill. Consider the surface on which the tower stands as a plane having points A(1, 0, 2), B(3, –1, 1) and C(1, 2, 1) on it. The mobile tower is tied with three cables from the points A, B and C such that it stands vertically on the ground. The top of the tower is at point P(2, 3, 1) as shown in the figure below. The foot of the perpendicular from the point P on the plane is at the point `Q(43/29, 77/29, 9/29)`.
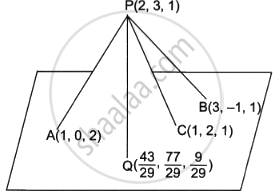
Answer the following questions.
- Find the equation of the plane containing the points A, B and C.
- Find the equation of the line PQ.
- Calculate the height of the tower.
Chapter: [0.06] Three - Dimensional Geometry (Section B)
Using integration, find the area bounded by the curve y2 = 4ax and the line x = a.
Chapter: [0.07] Application of Integrals (Section B)
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
Chapter: [0.07] Application of Integrals (Section B)
A company sells hand towels at ₹ 100 per unit. The fixed cost for the company to manufacture hand towels is ₹ 35,000 and variable cost is estimated to be 30% of total revenue. What will be the total cost function for manufacturing hand towels?
35000 + 3x
35000 + 30x
35000 + 100x
35000 + 10x
Chapter: [0.08] Application of Calculus (Section C)
If the correlation coefficient of two sets of variables (X, Y) is `(-3)/4`, which one of the following statements is true for the same set of variables?
Only one of the two regression lines has a negative coefficient.
Both regression coefficients are positive.
Both regression coefficients are negative.
One of the lines of regression is parallel to the x-axis.
Chapter: [0.09] Linear Regression (Section C)
If the total cost function is given by `C = x + 2x^3 - 7/2x^2`, find the Marginal Average Cost function (MAC).
Chapter: [0.08] Application of Calculus (Section C)
The equations of two lines of regression are 4x + 3y + 7 = 0 and 3x + 4y + 8 = 0. Find the mean value of x and y.
Chapter: [0.09] Linear Regression (Section C)
The manufacturer of a pen fixes its selling price at ₹ 45 and the cost function is C(x) = 30x + 240. The manufacturer will begin to earn profit if he sells more than 16 pens. Why? Give one reason.
Chapter: [0.08] Application of Calculus (Section C)
The Average Cost function associated with producing and marketing x units of an item is given by AC = `x + 5 + 36/x`.
- Find the Total cost function.
- Find the range of values of x for which Average Cost is increasing.
Chapter: [0.08] Application of Calculus (Section C)
A monopolist's demand function is `x = 60 - p/5`. At what level of output will marginal revenue be zero?
Chapter: [0.08] Application of Calculus (Section C)
A monopolist's demand function is `x = 60 - p/5`. At what level of output will marginal revenue be zero?
Chapter: [0.08] Application of Calculus (Section C)
For 50 students of a class, the regression equation of marks in statistics (X) on the marks in accountancy (Y) is 3y – 5x + 180 = 0. The mean marks in accountancy is 44 and the variance of marks in statistics is `(9/16)^(th)` of the variance of marks in accountancy. Find the mean marks in statistics and the correlation coefficient between marks in the two subjects.
Chapter: [0.09] Linear Regression (Section C)
Aman has ₹ 1500 to purchase rice and wheat for his grocery shop. Each sack of rice and wheat costs ₹ 180 and Rupee ₹ 120 respectively. He can store a maximum number of 10 bags in his shop. He will earn a profit of ₹ 11 per bag of rice and ₹ 9 per bag of wheat.
- Formulate a Linear Programming Problem to maximise Aman’s profit.
- Calculate the maximum profit.
Chapter: [0.1] Linear Programming (Section C)
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CISCE previous year question papers Class 12 Mathematics with solutions 2023 - 2024
Previous year Question paper for CISCE Class 12 -2024 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CISCE Class 12.
How CISCE Class 12 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
