Advertisements
Advertisements
प्रश्न
In ΔPQR, ∠P = 30°, ∠Q = 60°, ∠R = 90° and PQ = 12 cm, then find PR and QR.
उत्तर
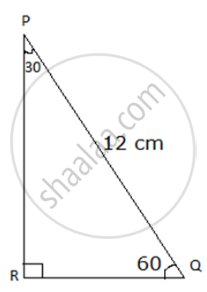
From figure using the definition of sin
sin∠P = `"RQ"/"PQ"`
∴ sin30° = `"RQ"/12`
∴ `1/2 = "RQ"/12`
∴ RQ = 12/2 = 6 cm
∴ QR = 6 cm
sin∠Q = `"PR"/"PQ"`
∴ sin 60° = `"PR"/12`
∴ `sqrt(3)/2 = "PR"/12`
∴ PR = `(12sqrt(3))/2`
∴ PR = `6sqrt3` cm
Therefore PR = `6sqrt3` cm and QR = 6 cm
APPEARS IN
संबंधित प्रश्न
If \[\sin\theta = \frac{7}{25}\], find the values of cosθ and tanθ.
If \[\tan \theta = \frac{3}{4}\], find the values of secθ and cosθ
If \[\cot\theta = \frac{40}{9}\], find the values of cosecθ and sinθ.
If 5 secθ – 12 cosecθ = 0, find the values of secθ, cosθ, and sinθ.
If tanθ = 1 then, find the value of
`(sinθ + cosθ)/(secθ + cosecθ)`
Prove that:
Prove that:
(secθ - cosθ)(cotθ + tanθ) = tanθ.secθ.
Prove that:
Choose the correct alternative answer for the following question.
cosec 45° =?
Choose the correct alternative answer for the following question.
Prove the following.
secθ (1 – sinθ) (secθ + tanθ) = 1
Prove the following.
(secθ + tanθ) (1 – sinθ) = cosθ
Prove the following.
sec2θ + cosec2θ = sec2θ × cosec2θ
Prove the following.
cot2θ – tan2θ = cosec2θ – sec2θ
Prove the following:
sec6x – tan6x = 1 + 3sec2x × tan2x
Prove the following.
\[\frac{\tan\theta}{\sec\theta + 1} = \frac{\sec\theta - 1}{\tan\theta}\]
If sinθ = `8/17`, where θ is an acute angle, find the value of cos θ by using identities.
ΔAMT∼ΔAHE, construct Δ AMT such that MA = 6.3 cm, ∠MAT=120°, AT = 4.9 cm and `"MA"/"HA"=7/5`, then construct ΔAHE.
Prove that: (sec θ – cos θ) (cot θ + tan θ) = tan θ.sec θ
Proof: L.H.S. = (sec θ – cos θ) (cot θ + tan θ)
= `(1/square - cos θ) (square/square + square/square)` ......`[∵ sec θ = 1/square, cot θ = square/square and tan θ = square/square]`
= `((1 - square)/square) ((square + square)/(square square))`
= `square/square xx 1/(square square)` ......`[(∵ square + square = 1),(∴ square = 1 - square)]`
= `square/(square square)`
= tan θ.sec θ
= R.H.S.
∴ L.H.S. = R.H.S.
∴ (sec θ – cos θ) (cot θ + tan θ) = tan θ.sec θ
