English Medium
Academic Year: 2018-2019
Date: March 2019
Duration: 3h
Advertisements
For what values of k does the quadratic equation 4x2 − 12x − k = 0 has no real roots?
Chapter: [0.023] Quadratic Equations
Find the distance between the points (a, b) and (−a, −b).
Chapter: [0.053] Some Applications of Trigonometry
Find a rational number between `sqrt(2) "and" sqrt(7)`.
Chapter: [0.011000000000000001] Real Numbers
Write the number of zeroes in the end of a number whose prime factorization is 22 × 53 × 32 × 17.
Chapter: [0.023] Quadratic Equations
Let ∆ ABC ∽ ∆ DEF and their areas be respectively, 64 cm2 and 121 cm2. If EF = 15⋅4 cm, find BC.
Chapter: [0.023] Quadratic Equations
Evaluate:
`(tan 65^circ)/(cot 25^circ)`
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Express (sin 67° + cos 75°) in terms of trigonometric ratios of the angle between 0° and 45°.
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Find the number of terms in the A.P.: 18, `15 1/2, 13, ...., -47.`
Chapter: [0.024] Arithmetic Progressions
A bag contains 15 balls, out of which some are white and the others are black. If the probability of drawing a black ball at random from the bag is `2/3`, then find how many white balls are there in the bag.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
A card is drawn at random from a pack of 52 playing cards. Find the probability of drawing a card that is neither a spade nor a king.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
Find the solution of the pair of the equation :
`3/x + 8/y = - 1; 1/x - 2/y = 2`, x, y ≠ 0
Chapter: [0.022000000000000002] Pair of Linear Equations in Two Variables
Find the value (s) of k for which the pair of equations
Chapter:
How many multiples of 4 lie between 10 and 205?
Chapter: [0.024] Arithmetic Progressions
Advertisements
Determine the A.P. whose 3rd term is 16 and the 7th term exceeds the 5th term by 12.
Chapter: [0.024] Arithmetic Progressions
Use Euclid's division algorithm to find the HCF of 255 and 867.
Chapter: [0.011000000000000001] Real Numbers
The point R divides the line segment AB, where A(−4, 0) and B(0, 6) such that AR=34AB.">AR = `3/4`AB. Find the coordinates of R.
Chapter: [0.031] Lines (In Two-dimensions) [0.031] Lines (In Two-dimensions)
Prove that:
(sin θ + 1 + cos θ) (sin θ − 1 + cos θ) . sec θ cosec θ = 2
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Prove that:
`sqrt((sectheta - 1)/(sec theta + 1)) + sqrt((sectheta + 1)/(sectheta - 1)) = 2cosectheta`
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
In what ratio does the point P(−4, y) divides the line segment joining the points A(−6, 10) and B(3, −8)? Hence find the value of y.
Chapter: [0.031] Lines (In Two-dimensions)
Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
Chapter: [0.031] Lines (In Two-dimensions)
ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
Chapter: [0.042] Circles
In the given figure, BL and CM are medians of a ∆ABC right-angled at A. Prove that 4 (BL2 + CM2) = 5 BC2.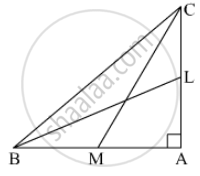
Chapter: [0.040999999999999995] Triangles
Prove that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals
Chapter: [0.040999999999999995] Triangles
In Figure 2, two concentric circles with centre O, have radii 21 cm and 42 cm. If ∠AOB = 60°, find the area of the shaded region.

Chapter: [0.061] Areas Related to Circles
Calculate the mode of the following distribution:
| Class | 10 − 15 | 15 − 20 | 20 − 25 | 25 − 30 | 30 − 35 |
| Frequency | 4 | 7 | 20 | 8 | 1 |
Chapter: [0.071] Statistics
A cone of height 24 cm and radius of base 6 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the radius of the sphere and hence find the surface area of this sphere.
Chapter: [0.062] Surface Areas and Volumes
Advertisements
A farmer connects a pipe of internal diameter 20 cm form a canal into a cylindrical tank in his field, which is 10 m in diameter and 2 m deep. If water flows through the pipe at the rate of 3 km/h, in how much time will the tank be filled?
Chapter: [0.062] Surface Areas and Volumes
Prove that `2 + 3sqrt(3)` is an irrational number when it is given that `sqrt(3)` is an irrational number.
Chapter: [0.011000000000000001] Real Numbers
Sum of the areas of two squares is 157 m2. If the sum of their perimeters is 68 m, find the sides of the two squares.
Chapter: [0.061] Areas Related to Circles
Find the quadratic polynomial, sum, and product of whose zeroes are −1 and −20 respectively. Also, find the zeroes of the polynomial so obtained.
Chapter: [0.021] Polynomials
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km away on time, it has to increase its speed by 250 km/hr from its usual speed. Find the usual speed of the plane.
Chapter: [0.061] Areas Related to Circles
Find the dimensions of a rectangular park whose perimeter is 60 m and area 200 m2.
Chapter: [0.061] Areas Related to Circles
Find the value of x, when in the A.P. given below 2 + 6 + 10 + ... + x = 1800.
Chapter: [0.024] Arithmetic Progressions
If sec θ + tan θ = m, show that `(m^2 - 1)/(m^2 + 1) = sin theta`
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
In ∆ ABC, AD ⊥ BC.
Prove that AC2 = AB2 +BC2 − 2BC x BD
Chapter: [0.040999999999999995] Triangles
A moving boat is observed from the top of a 150 m high cliff moving away from the cliff. The angle of depression of the boat changes from 60° to 45° in 2 minutes. Find the speed of the boat in m/min.
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
There are two poles, one each on either bank of a river just opposite to each other. One pole is 60 m high. From the top of this pole, the angle of depression of the top and foot of the other pole are 30° and 60° respectively. Find the width of the river and height of the other pole.
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Construct a triangle with sides 5 cm, 6 cm, and 7 cm and then another triangle whose sides are `3/5` of the corresponding sides of the first triangle.
Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
Calculate the mean of the following frequency distribution :
| Class: | 10-30 | 30-50 | 50-70 | 70-90 | 90-110 | 110-130 |
| Frequency: | 5 | 8 | 12 | 20 | 3 | 2 |
Chapter: [0.071] Statistics
The following table gives production yield in kg per hectare of wheat of 100 farms of a village :
| Production yield (kg/hectare) : |
40−45 | 45−50 | 50−55 | 55−60 | 60−65 | 65−70 |
| Number of farms | 4 | 6 | 16 | 20 | 30 | 24 |
Change the distribution to a 'more than type' distribution, and draw its ogive.
Chapter: [0.031] Lines (In Two-dimensions)
A container opened at the top and made up of a metal sheet, is in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm respectively. Find the cost of milk which can completely fill the container, at the rate of ₹ 50 per litre. Also find the cost of metal sheet used to make the container, if it costs ₹ 10 per 100 cm2. (Take π = 3⋅14)
Chapter: [0.062] Surface Areas and Volumes
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CBSE previous year question papers Class 10 Mathematics with solutions 2018 - 2019
Previous year Question paper for CBSE Class 10 Maths-2019 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CBSE Class 10.
How CBSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
