Advertisements
Advertisements
प्रश्न
Find the area of the region bounded by the curve \[x = a t^2 , y = 2\text{ at }\]between the ordinates corresponding t = 1 and t = 2.
उत्तर
The curve \[x = a t^2 , y = 2\text{ at }\] represents the parametric equation of the parabola.
Eliminating the parameter t, we get \[y^2 = 4ax\]
This represents the Cartesian equation of the parabola opening towards the positive x-axis with focus at (a, 0).
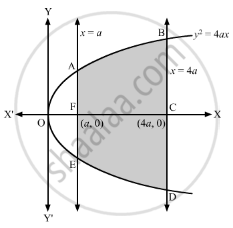
When t = 1, x = a
When t = 2, x = 4a
∴ Required area = Area of the shaded region
= 2 × Area of the region ABCFA
\[= 2 \int_a^{4a} y_{\text{ parabola }} dx\]
\[ = 2 \int_a^{4a} \sqrt{4ax}dx\]
\[ = \left.2 \times {2\sqrt{a} \times \frac{x^\frac{3}{2}}{\frac{3}{2}}}\right|_a^{4a} \]
\[ = \frac{8\sqrt{a}}{3}\left[ \left( 4a \right)^\frac{3}{2} - a^\frac{3}{2} \right]\]
\[ = \frac{8\sqrt{a}}{3}\left( 8a\sqrt{a} - a\sqrt{a} \right)\]
\[ = \frac{8\sqrt{a}}{3} \times 7a\sqrt{a}\]
\[ = \frac{56}{3} a^2\text{ square units }\]
APPEARS IN
संबंधित प्रश्न
Find the area bounded by the curve y2 = 4ax, x-axis and the lines x = 0 and x = a.
Find the area of ellipse `x^2/1 + y^2/4 = 1`
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Find the area lying above the x-axis and under the parabola y = 4x − x2.
Draw a rough sketch of the graph of the curve \[\frac{x^2}{4} + \frac{y^2}{9} = 1\] and evaluate the area of the region under the curve and above the x-axis.
Draw a rough sketch of the curve y = \[\frac{\pi}{2} + 2 \sin^2 x\] and find the area between x-axis, the curve and the ordinates x = 0, x = π.
Find the area of the minor segment of the circle \[x^2 + y^2 = a^2\] cut off by the line \[x = \frac{a}{2}\]
Find the area of the region in the first quadrant bounded by the parabola y = 4x2 and the lines x = 0, y = 1 and y = 4.
Find the area of the region bounded by x2 = 4ay and its latusrectum.
Find the area of the region \[\left\{ \left( x, y \right): \frac{x^2}{a^2} + \frac{y^2}{b^2} \leq 1 \leq \frac{x}{a} + \frac{y}{b} \right\}\]
Draw a rough sketch of the region {(x, y) : y2 ≤ 3x, 3x2 + 3y2 ≤ 16} and find the area enclosed by the region using method of integration.
Find the area of the region bounded by \[y = \sqrt{x}, x = 2y + 3\] in the first quadrant and x-axis.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Using integration find the area of the region:
\[\left\{ \left( x, y \right) : \left| x - 1 \right| \leq y \leq \sqrt{5 - x^2} \right\}\]
Find the area bounded by the lines y = 4x + 5, y = 5 − x and 4y = x + 5.
Find the area enclosed by the parabolas y = 4x − x2 and y = x2 − x.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
The area included between the parabolas y2 = 4x and x2 = 4y is (in square units)
The area bounded by the parabola y2 = 4ax and x2 = 4ay is ___________ .
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
Area bounded by the curve y = x3, the x-axis and the ordinates x = −2 and x = 1 is ______.
Find the coordinates of a point of the parabola y = x2 + 7x + 2 which is closest to the straight line y = 3x − 3.
Using integration, find the area of the region bounded by the line x – y + 2 = 0, the curve x = \[\sqrt{y}\] and y-axis.
Find the area of the region bounded by the curves y2 = 9x, y = 3x
Find the area of the region bounded by the parabola y2 = 2px, x2 = 2py
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2
Draw a rough sketch of the region {(x, y) : y2 ≤ 6ax and x 2 + y2 ≤ 16a2}. Also find the area of the region sketched using method of integration.
Draw a rough sketch of the given curve y = 1 + |x +1|, x = –3, x = 3, y = 0 and find the area of the region bounded by them, using integration.
The area of the region bounded by the y-axis, y = cosx and y = sinx, 0 ≤ x ≤ `pi/2` is ______.
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is ______.
The area of the region bounded by the ellipse `x^2/25 + y^2/16` = 1 is ______.
Using integration, find the area of the region `{(x, y): 0 ≤ y ≤ sqrt(3)x, x^2 + y^2 ≤ 4}`
The area of the region enclosed by the parabola x2 = y, the line y = x + 2 and the x-axis, is
The region bounded by the curves `x = 1/2, x = 2, y = log x` and `y = 2^x`, then the area of this region, is
Find the area bounded by the curve y = |x – 1| and y = 1, using integration.
Using integration, find the area of the region bounded by the curves x2 + y2 = 4, x = `sqrt(3)`y and x-axis lying in the first quadrant.
Find the area of the region enclosed by the curves y2 = x, x = `1/4`, y = 0 and x = 1, using integration.
Area (in sq.units) of the region outside `|x|/2 + |y|/3` = 1 and inside the ellipse `x^2/4 + y^2/9` = 1 is ______.
Find the area of the region bounded by the curve x2 = 4y and the line x = 4y – 2.
