Advertisements
Advertisements
प्रश्न
The range of f(x) = cos [x], for π/2 < x < π/2 is
पर्याय
(a) {−1, 1, 0}
(b) {cos 1, cos 2, 1}
(c) {cos 1, −cos 1, 1}
(d) [−1, 1]
उत्तर
(b) {cos 1, cos 2, 1}
Since, f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\]
\[- \frac{\pi}{2} < x < \frac{\pi}{2}\]
\[ \Rightarrow - 1 . 57 < x < 1 . 57\]
\[ \Rightarrow [x] \in { - 1, 0, 1, 2}\]
\[\text{ Thus } , \cos [x] = {\cos ( - 1), \cos 0, \cos1, \cos 2 }\]
\[\text{ Range of } f(x) = {\cos 1, 1, \cos 2}\]
APPEARS IN
संबंधित प्रश्न
Define a function as a correspondence between two sets.
f, g, h are three function defined from R to R as follow:
(ii) g(x) = sin x
Find the range of function.
If f : R → R be defined by f(x) = x2 + 1, then find f−1 [17] and f−1 [−3].
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(v) \[\frac{g}{f}\]
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(i) f + g
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(iii) \[\frac{f}{g}\]
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(iv) \[\frac{g}{f}\] Also, find (f + g) (−1), (fg) (0),
Write the domain and range of function f(x) given by
Write the domain and range of function f(x) given by \[f\left( x \right) = \sqrt{\left[ x \right] - x}\] .
Find the set of values of x for which the functions f(x) = 3x2 − 1 and g(x) = 3 + x are equal.
Which one of the following is not a function?
If \[f\left( x \right) = \log \left( \frac{1 + x}{1 - x} \right)\] , then \[f\left( \frac{2x}{1 + x^2} \right)\] is equal to
If f(x) = cos (log x), then value of \[f\left( x \right) f\left( 4 \right) - \frac{1}{2} \left\{ f\left( \frac{x}{4} \right) + f\left( 4x \right) \right\}\] is
If \[f\left( x \right) = \frac{2^x + 2^{- x}}{2}\] , then f(x + y) f(x − y) is equal to
If f(m) = m2 − 3m + 1, find f(0)
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(3)
If f(x) =` (2x−1)/ (5x−2) , x ≠ 2/5` Verify whether (fof) (x) = x
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(2)
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 2), (2, −1), (3, 1), (4, 3)}
Check if the relation given by the equation represents y as function of x:
2y + 10 = 0
Let f be a subset of Z × Z defined by f = {(ab, a + b) : a, b ∈ Z}. Is f a function from Z to Z? Justify?
Check the injectivity and surjectivity of the following function.
f : N → N given by f(x) = x2
Check the injectivity and surjectivity of the following function.
f : Z → Z given by f(x) = x2
Express the following exponential equation in logarithmic form
`9^(3/2)` = 27
Express the following exponential equation in logarithmic form
`"e"^(1/2)` = 1.6487
Express the following logarithmic equation in exponential form
In `1/2` = – 0.693
Prove that alogcb = blogca
If x = loga bc, y = logb ca, z = logc ab then prove that `1/(1 + x) + 1/(1 + y) + 1/(1 + z)` = 1
Answer the following:
Find whether the following function is one-one
f : R → R defined by f(x) = x2 + 5
Answer the following:
A function f : R → R defined by f(x) = `(3x)/5 + 2`, x ∈ R. Show that f is one-one and onto. Hence find f–1
Answer the following:
If f(x) = log(1 – x), 0 ≤ x < 1 show that `"f"(1/(1 + x))` = f(1 – x) – f(– x)
Find the domain of the following function.
f(x) = `sqrtlog(x^2 - 6x + 6)`
A graph representing the function f(x) is given in it is clear that f(9) = 2
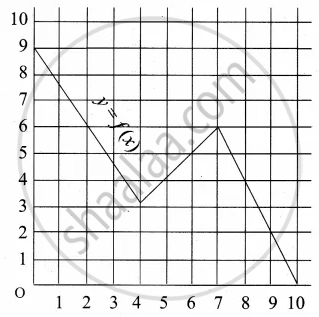
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
The range of the function f(x) = `(x - 3)/(5 - x)`, x ≠ 5 is ______.
The domain of the function f defined by f(x) = `1/sqrt(x - |x|)` is ______.
Let A and B be any two sets such that n(B) = p, n(A) = q then the total number of functions f : A → B is equal to ______.
Domain of `sqrt(a^2 - x^2) (a > 0)` is ______.
The ratio `(2^(log_2 1/4 a) - 3^(log_27(a^2 + 1)^3) - 2a)/(7^(4log_49a) - a - 1)` simplifies to ______.
Which of the following functions is NOT one-one?
