Question

Find the area of the circle x² + y² = 16 which is exterior to the parabola y² = 6x.

Answer 1

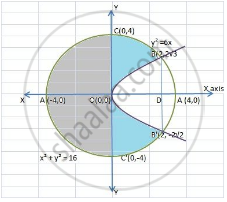
Points of intersection of the parabola and the circle is obtained by solving the simultaneous equations
$$x^2+y^2=16\text{ and }{y}^2=6x$$
$$\Rightarrow x^2+6x=16$$
$$\Rightarrow x^2+6x-16=0$$
$$\Rightarrow (x+8)(x-2)=0$$
$$\Rightarrow x=2\text{ or }x=-8,\text{ which is not the possible solution . }$$
$$\therefore \text{ When }x=2,y=\pm \sqrt{6\times 2}=\pm \sqrt{12}=\pm 2\sqrt{3}$$
$$\therefore \text{ B }(2,2\sqrt{3})\text{ and B' }(2,-2\sqrt{3})\text{ are points of intersection of the parabola and circle . }$$
$$\text{ Required area = Area }\left(O{B}^{\prime }{C}^{\prime }{A}^{\prime }CBO\right)=\text{ area of circle - area }\left(OBA{B}^{\prime }O\right)$$
$$\text{ Area of circle with radius }4=\pi \times 4^2=16\pi$$
 Now,
$$\text{ Area OBAB'O = 2area }\left(OBAO\right)$$
$$=2\{\text{ area }\left(OBDO\right)+\text{ area }\left(DBAD\right)\}$$
$$=2\times [{\int }_0^2\sqrt{6x}dx+{\int }_2^4\sqrt{16-x^2}dx]$$
$$=2\times \{{\left[\sqrt{6}\frac{x^{\frac{3}{2}}}{\frac{3}{2}}\right]}_0^2+{[\frac{1}{2}x\sqrt{16-x^2}+\frac{1}{2}\times 16{\sin }^{-1}\left(\frac{x}{a}\right)]}_2^4\}$$
$$=2\times \{(\sqrt{6}\times \frac{2}{3}\times 2^{\frac{3}{2}}-0)+(\frac{1}{2}4\sqrt{16-{\left(4\right)}^2}+\frac{1}{2}\times 16{\sin }^{-1}\frac{4}{4}-\frac{1}{2}\times 2\sqrt{16-2^2}-\frac{1}{2}\times 16{\sin }^{-1}\frac{2}{4})\}$$
$$=2\times \{(\sqrt{6}\times \frac{2}{3}\times 2\sqrt{2})+0+8{\sin }^{-1}\left(1\right)-\sqrt{12}-8{\sin }^{-1}\left(\frac{1}{2}\right)\}$$
$$=2\times [\frac{8\sqrt{3}}{3}+8\times \frac{\pi }{2}-2\sqrt{3}-8\frac{\pi }{6}]$$
$$=2\{\frac{8\sqrt{3}-6\sqrt{3}}{3}+8(\frac{\pi }{2}-\frac{\pi }{6})\}$$
$$=2\{\frac{2\sqrt{3}}{3}+8\left(\frac{2\pi }{6}\right)\}$$
$$=\frac{4\sqrt{3}}{3}+\frac{16\pi }{3}$$
$$\text{ Shaded area }=16\pi -(\frac{4\sqrt{3}}{3}+\frac{16\pi }{3})$$
$$=\frac{48\pi -16\pi }{3}-\frac{4\sqrt{3}}{3}$$
$$=\frac{32\pi }{3}-\frac{4\sqrt{3}}{3}$$
$$=\frac{4}{3}(8\pi -\sqrt{3})\text{ sq units }$$