Advertisements
Advertisements
Question
The area bounded by the curve y = loge x and x-axis and the straight line x = e is ___________ .
Options
e sq. units
1 sq. units
1−\[\frac{1}{e}\] sq. units
1+\[\frac{1}{e}\] sq. units
Advertisements
Solution
1 sq. units
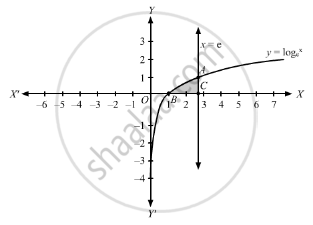
The point of intersection of the curve and the straight line is A(e, 1).
Therefore, the area of the required region ABC,
\[A = \int_0^1 \left( x_1 - x_2 \right) d y ..........\left(\text{ where, }x_1 = e\text{ and }x_2 = e^y \right)\]
\[ = \int_0^1 \left( e - e^y \right) d y\]
\[ = \left[ ey - e^y \right]_0^1 \]
\[ = \left\{ e\left( 1 \right) - e^\left( 1 \right) \right\} - \left\{ e\left( 0 \right) - e^\left( 0 \right) \right\}\]
\[ = e - e + 1\]
\[ = 1\text{ square unit }\]
APPEARS IN
RELATED QUESTIONS
Find the area bounded by the curve y2 = 4ax, x-axis and the lines x = 0 and x = a.
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
Sketch the graph of y = \[\sqrt{x + 1}\] in [0, 4] and determine the area of the region enclosed by the curve, the x-axis and the lines x = 0, x = 4.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Sketch the graph y = | x − 5 |. Evaluate \[\int\limits_0^1 \left| x - 5 \right| dx\]. What does this value of the integral represent on the graph.
Find the area of the region bounded by x2 + 16y = 0 and its latusrectum.
Calculate the area of the region bounded by the parabolas y2 = x and x2 = y.
Find the area of the region bounded by y =\[\sqrt{x}\] and y = x.
Using integration, find the area of the triangular region, the equations of whose sides are y = 2x + 1, y = 3x+ 1 and x = 4.
Find the area of the region bounded by \[y = \sqrt{x}, x = 2y + 3\] in the first quadrant and x-axis.
Find the area enclosed by the curve \[y = - x^2\] and the straight line x + y + 2 = 0.
Find the area of the circle x2 + y2 = 16 which is exterior to the parabola y2 = 6x.
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2.
Find the area of the region enclosed between the two curves x2 + y2 = 9 and (x − 3)2 + y2 = 9.
Find the area enclosed by the parabolas y = 4x − x2 and y = x2 − x.
In what ratio does the x-axis divide the area of the region bounded by the parabolas y = 4x − x2 and y = x2− x?
The area bounded by the parabola y2 = 4ax, latusrectum and x-axis is ___________ .
The area of the region (in square units) bounded by the curve x2 = 4y, line x = 2 and x-axis is
The area of the circle x2 + y2 = 16 enterior to the parabola y2 = 6x is
Find the area of the region bounded by the parabolas y2 = 6x and x2 = 6y.
Find the area of the region bounded by the curve y = x3 and y = x + 6 and x = 0
Find the area of the region bounded by the curve y2 = 4x, x2 = 4y.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
The area of the region bounded by parabola y2 = x and the straight line 2y = x is ______.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
The curve x = t2 + t + 1,y = t2 – t + 1 represents
Find the area of the region bounded by `y^2 = 9x, x = 2, x = 4` and the `x`-axis in the first quadrant.
Find the area of the region bounded by the curve `y = x^2 + 2, y = x, x = 0` and `x = 3`
The area of the region S = {(x, y): 3x2 ≤ 4y ≤ 6x + 24} is ______.
Let f : [–2, 3] `rightarrow` [0, ∞) be a continuous function such that f(1 – x) = f(x) for all x ∈ [–2, 3]. If R1 is the numerical value of the area of the region bounded by y = f(x), x = –2, x = 3 and the axis of x and R2 = `int_-2^3 xf(x)dx`, then ______.
Let a and b respectively be the points of local maximum and local minimum of the function f(x) = 2x3 – 3x2 – 12x. If A is the total area of the region bounded by y = f(x), the x-axis and the lines x = a and x = b, then 4A is equal to ______.
Using integration, find the area of the region bounded by y = mx (m > 0), x = 1, x = 2 and the X-axis.
Make a rough sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 1, 0 ≤ y ≤ x + 1, 0 ≤ x ≤ 2} and find the area of the region, using the method of integration.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
