Advertisements
Advertisements
Question
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
Solution
`log((x + y)/3) = 1/2 log x + 1/2 logy`
∴ `log((x + y)/3) = 1/2 (log x + logy)`
∴ `log((x + y)/3) = 1/2 log xy`
∴ `log((x + y)/3) = log(xy)^(1/2)`
∴ `(x + y)/3 = (xy)^(1/2)`
Squaring both sides, we get,
`((x + y)/3)^2` = xy
∴ `(x + y)^2/9` = xy
∴ x2 + 2xy + y2 = 9xy
∴ x2 + y2 = 7xy
Dividing both sides by xy, we get,
`(x^2)/(xy) + (y^2)/(xy) = (7xy)/(xy)`
∴ `x/y + y/x` = 7
APPEARS IN
RELATED QUESTIONS
Which of the following relations are functions? Give reasons. If it is a function, determine its domain and range.
- {(2, 1), (5, 1), (8, 1), (11, 1), (14, 1), (17, 1)}
- {(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
- {(1, 3), (1, 5), (2, 5)}
Let A = [p, q, r, s] and B = [1, 2, 3]. Which of the following relations from A to B is not a function?
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
Write the domain and range of the function \[f\left( x \right) = \frac{x - 2}{2 - x}\] .
If f, g, h are real functions given by f(x) = x2, g(x) = tan x and h(x) = loge x, then write the value of (hogof)\[\left( \sqrt{\frac{\pi}{4}} \right)\] .
Let A and B be two sets such that n(A) = p and n(B) = q, write the number of functions from A to B.
Find the set of values of x for which the functions f(x) = 3x2 − 1 and g(x) = 3 + x are equal.
If f(x) = cos (log x), then the value of f(x2) f(y2) −
If \[f\left( x \right) = \frac{2^x + 2^{- x}}{2}\] , then f(x + y) f(x − y) is equal to
The range of the function \[f\left( x \right) = \frac{x^2 - x}{x^2 + 2x}\] is
If f : R → R be given by for all \[f\left( x \right) = \frac{4^x}{4^x + 2}\] x ∈ R, then
The domain of the function
The domain of the function \[f\left( x \right) = \sqrt{\frac{\left( x + 1 \right) \left( x - 3 \right)}{x - 2}}\] is
The domain of definition of the function f(x) = log |x| is
The domain of the function \[f\left( x \right) = \sqrt{5 \left| x \right| - x^2 - 6}\] is
Check if the following relation is function:
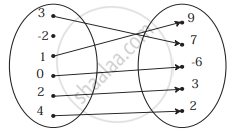
If f(m) = m2 − 3m + 1, find `f(1/2)`
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(0)
Check if the following relation is a function.
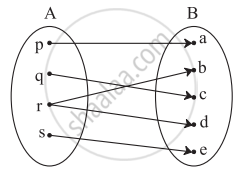
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 2), (2, −1), (3, 1), (4, 3)}
Show that if f : A → B and g : B → C are onto, then g ° f is also onto
Express the following exponential equation in logarithmic form
25 = 32
Find the domain of f(x) = ln (x − 5)
Solve for x.
2 log10 x = `1 + log_10 (x + 11/10)`
If f(x) = 3x + 5, g(x) = 6x − 1, then find (f − g) (2)
Select the correct answer from given alternatives.
Let the function f be defined by f(x) = `(2x + 1)/(1 - 3x)` then f–1 (x) is ______.
Answer the following:
Simplify, log (log x4) – log (log x)
Find the domain of the following function.
f(x) = `sqrtlog(x^2 - 6x + 6)`
Given the function f: x → x2 – 5x + 6, evaluate f(x – 1)
A graph representing the function f(x) is given in it is clear that f(9) = 2
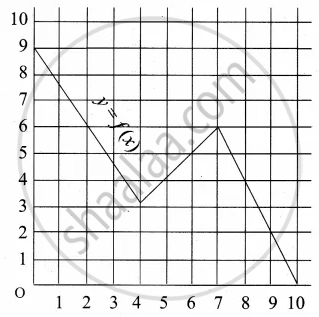
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
A function f is defined by f(x) = 2x – 3 find `("f"(0) + "f"(1))/2`
A function f is defined by f(x) = 2x – 3 find x such that f(x) = 0
The domain of the function f(x) = log3+x (x2 - 1) is ______.
Find the domain of the following functions given by f(x) = `1/sqrt(1 - cos x)`
Find the domain of the following functions given by f(x) = `(x^3 - x + 3)/(x^2 - 1)`
Find the domain of the following function given by:
f(x) = `(3x)/(2x - 8)`
If f(x) = y = `(ax - b)/(cx - a)`, then prove that f(y) = x.
Range of the function f(x) = `x/(1 + x^2)` is ______.
