Topics
Rational Numbers
- Rational Numbers
- Closure Property of Rational Numbers
- Commutative Property of Rational Numbers
- Associative Property of Rational Numbers
- Distributive Property of Multiplication Over Addition for Rational Numbers
- Identity of Addition and Multiplication of Rational Numbers
- Negative Or Additive Inverse of Rational Numbers
- Concept of Reciprocal or Multiplicative Inverse
- Rational Numbers on a Number Line
- Rational Numbers Between Two Rational Numbers
Linear Equations in One Variable
- Variable of Equation
- Concept of Equation
- Expressions with Variables
- Balancing an Equation
- The Solution of an Equation
- Linear Equation in One Variable
- Solving Equations Which Have Linear Expressions on One Side and Numbers on the Other Side
- Some Applications Solving Equations Which Have Linear Expressions on One Side and Numbers on the Other Side
- Solving Equations Having the Variable on Both Sides
- Some More Applications on the Basis of Solving Equations Having the Variable on Both Sides
- Reducing Equations to Simpler Form
- Equations Reducible to the Linear Form
Understanding Quadrilaterals
- Concept of Curves
- Different Types of Curves - Closed Curve, Open Curve, Simple Curve.
- Concept of Polygons
- Classification of Polygons
- Properties of a Quadrilateral
- Interior Angles of a Polygon
- Exterior Angles of a Polygon and Its Property
- Concept of Quadrilaterals
- Properties of Trapezium
- Properties of Kite
- Properties of a Parallelogram
- Properties of Rhombus
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Property: The adjacent angles in a parallelogram are supplementary.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Property: The diagonals of a rhombus are perpendicular bisectors of one another.
- Property: The Diagonals of a Rectangle Are of Equal Length.
- Properties of Rectangle
- Properties of a Square
- Property: The diagonals of a square are perpendicular bisectors of each other.
Practical Geometry
- Introduction to Geometric Tool
- Constructing a Quadrilateral When the Lengths of Four Sides and a Diagonal Are Given
- Constructing a Quadrilateral When Two Diagonals and Three Sides Are Given
- Constructing a Quadrilateral When Two Adjacent Sides and Three Angles Are Known
- Constructing a Quadrilateral When Three Sides and Two Included Angles Are Given
- Some Special Cases
Data Handling
- Concept of Data Handling
- Interpretation of a Pictograph
- Interpretation of Bar Graphs
- Drawing a Bar Graph
- Interpretation of a Double Bar Graph
- Drawing a Double Bar Graph
- Organisation of Data
- Frequency Distribution Table
- Graphical Representation of Data as Histograms
- Concept of Pie Graph (Or a Circle-graph)
- Interpretation of Pie Diagram
- Chance and Probability - Chance
- Basic Ideas of Probability
Squares and Square Roots
- Concept of Square Number
- Properties of Square Numbers
- Some More Interesting Patterns of Square Number
- Finding the Square of a Number
- Concept of Square Roots
- Finding Square Root Through Repeated Subtraction
- Finding Square Root Through Prime Factorisation
- Finding Square Root by Division Method
- Square Root of Decimal Numbers
- Estimating Square Root
Cubes and Cube Roots
Comparing Quantities
- Concept of Ratio
- Basic Concept of Percentage
- Increase Or Decrease as Percent
- Concept of Discount
- Estimation in Percentages
- Basic Concepts of Profit and Loss
- Sales Tax, Value Added Tax, and Good and Services Tax
- Calculation of Interest
- Concept of Compound Interest
- Deducing a Formula for Compound Interest
- Rate Compounded Annually Or Half Yearly (Semi Annually)
- Applications of Compound Interest Formula
Algebraic Expressions and Identities
- Algebraic Expressions
- Terms, Factors and Coefficients of Expression
- Types of Algebraic Expressions as Monomials, Binomials, Trinomials, and Polynomials
- Like and Unlike Terms
- Addition of Algebraic Expressions
- Subtraction of Algebraic Expressions
- Multiplication of Algebraic Expressions
- Multiplying Monomial by Monomials
- Multiplying a Monomial by a Binomial
- Multiplying a Monomial by a Trinomial
- Multiplying a Binomial by a Binomial
- Multiplying a Binomial by a Trinomial
- Concept of Identity
- Expansion of (a + b)2 = a2 + 2ab + b2
- Expansion of (a - b)2 = a2 - 2ab + b2
- Expansion of (a + b)(a - b) = a2-b2
- Expansion of (x + a)(x + b)
Mensuration
Visualizing Solid Shapes
Exponents and Powers
Direct and Inverse Proportions
Factorization
- Factors and Multiples
- Factorising Algebraic Expressions
- Factorisation by Taking Out Common Factors
- Factorisation by Regrouping Terms
- Factorisation Using Identities
- Factors of the Form (x + a)(x + b)
- Dividing a Monomial by a Monomial
- Dividing a Polynomial by a Monomial
- Dividing a Polynomial by a Polynomial
- Concept of Find the Error
Introduction to Graphs
- Concept of Bar Graph
- Interpretation of Bar Graphs
- Drawing a Bar Graph
- Concept of Double Bar Graph
- Interpretation of a Double Bar Graph
- Drawing a Double Bar Graph
- Concept of Pie Graph (Or a Circle-graph)
- Graphical Representation of Data as Histograms
- Concept of a Line Graph
- Linear Graphs
- Some Application of Linear Graphs
Playing with Numbers
Notes
Finding Square Root by Division Method:
When the numbers are large, even the method of finding square root by prime factorization becomes lengthy and difficult. To overcome this problem we use Long Division Method.
Consider the following steps to find the square root of 529.
Step 1: Place a bar over every pair of digits starting from the digit at one’s place. If the number of digits in it is odd, then the left-most single-digit too will have a bar. Thus we have, `bar(5) bar(29)`.
Step 2: Find the largest number whose square is less than or equal to the number under the extreme left bar (22 < 5 < 32). Take this number as the divisor and the quotient with the number under the extreme left bar as the dividend (here 5).
Divide and get the remainder (1 in this case).
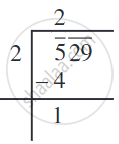
Step 3: Bring down the number under the next bar (i.e., 29 in this case) to the right of the remainder. So the new dividend is 129.
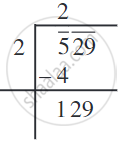
Step 4: Double the quotient and enter it with a blank on its right.
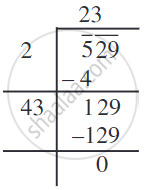
Step 5: Guess the largest possible digit to fill the blank which will also become the new digit in the quotient, such that when the new divisor is multiplied to the new quotient the product is less than or equal to the dividend.
In this case 42 × 2 = 84.
As 43 × 3 = 129 so we choose the new digit as 3. Get the remainder.

Step 6: Since the remainder is 0 and no digits are left in the given number, therefore, `sqrt(529)` = 23.
Now consider `sqrt(4096)`.
Step 1: Place a bar over every pair of digits starting from the one’s digit. `(bar(40) bar(96))`.
Step 2: Find the largest number whose square is less than or equal to the number under the left-most bar (62 < 40 < 72). Take this number as the divisor and the number under the leftmost bar as the dividend.
Divide and get the remainder i.e., 4 in this case.
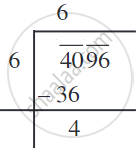
Step 3: Bring down the number under the next bar (i.e., 96) to the right of the remainder. The new dividend is 496.
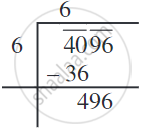
Step 4: Double the quotient and enter it with a blank on its right.
Step 5: Guess the largest possible digit to fill the blank which also becomes the new digit in the quotient such that when the new digit is multiplied to the new quotient the product is less than or equal to the dividend. In this case, we see that 124 × 4 = 496.
So the new digit in the quotient is 4. Get the remainder.

Step 6: Since the remainder is 0 and no bar left, therefore, `sqrt(4096) = 64.`

Example
Find the square root of 1296.

Therefore, `sqrt(1296) = 36`.
Example
Find the greatest 4-digit number which is a perfect square.
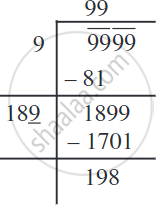
Greatest number of 4-digits = 9999.
We find `sqrt(9999)` by long division method. The remainder is 198.
This shows 992 is less than 9999 by 198.
This means if we subtract the remainder from the number, we get a perfect square.
Therefore, the required perfect square is 9999 - 198 = 9801.
And, `sqrt(9801) = 99.`
If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | Finding Square Root by Long Division Method
to track your progress
Series: Finding Square Root by Long Division Method
0%
