Advertisements
Advertisements
प्रश्न
An isosceles triangle of vertical angle 2 \[\theta\] is inscribed in a circle of radius a. Show that the area of the triangle is maximum when \[\theta\] = \[\frac{\pi}{6}\] .
Advertisements
उत्तर
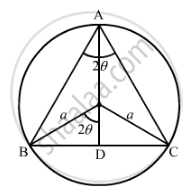
Let ABC be an isosceles triangle inscribed in the circle with radius a such that AB = AC.
\[AD = AO + OD = a + a\cos2\theta = a\left( 1 + \cos2\theta \right)and\]
\[BC = 2BD = 2a\sin2\theta\]
\[\text { As, area of the triangle } AC, A = \frac{1}{2}BC \times AD\]
\[ \Rightarrow A\left( \theta \right) = \frac{1}{2} \times 2a\sin2\theta \times a\left( 1 + \cos2\theta \right)\]
\[ = a^2 \sin2\theta\left( 1 + \cos2\theta \right)\]
\[ = a^2 \sin2\theta + a^2 \sin2\theta\cos2\theta\]
\[ \Rightarrow A\left( \theta \right) = a^2 \sin2\theta + \frac{a^2 \sin4\theta}{2}\]
\[ \Rightarrow A'\left( \theta \right) = 2 a^2 \cos2\theta + \frac{4 a^2 \cos4\theta}{2}\]
\[ \Rightarrow A'\left( \theta \right) = 2 a^2 \cos2\theta + 2 a^2 \cos4\theta\]
\[ \Rightarrow A'\left( \theta \right) = 2 a^2 \left( \cos2\theta + \cos4\theta \right)\]
\[\text { For maxima or minima }, A'\left( \theta \right) = 0\]
\[ \Rightarrow 2 a^2 \left( \cos2\theta + \cos4\theta \right) = 0\]
\[ \Rightarrow \cos2\theta + \cos4\theta = 0\]
\[ \Rightarrow \cos2\theta = - \cos4\theta\]
\[ \Rightarrow \cos2\theta = \cos\left( \pi - 4\theta \right)\]
\[ \Rightarrow 2\theta = \pi - 4\theta\]
\[ \Rightarrow 6\theta = \pi\]
\[ \Rightarrow \theta = \frac{\pi}{6}\]
\[\text { Also,} A''\left( \theta \right) = 2 a^2 \left( - \sin2\theta - \sin4\theta \right) = - 2 a^2 \left( \sin2\theta + \sin4\theta \right) < 0 \text { at }\theta = \frac{\pi}{6} . \]
\[\text { So, the area of the triangle is maximum at } \theta = \frac{\pi}{6} .\]
