Advertisements
Advertisements
प्रश्न
Find the interval in which the following function are increasing or decreasing \[f\left( x \right) = \left\{ x(x - 2) \right\}^2\] ?
उत्तर
\[\text { When } \left( x - a \right)\left( x - b \right)>0 \text { with }a < b, x < a \text { or }x>b.\]
\[\text { When } \left( x - a \right)\left( x - b \right)<0 \text { with } a < b, a < x < b .\]
\[f\left( x \right) = \left\{ x\left( x - 2 \right) \right\}^2 \]
\[ = \left( x^2 - 2x \right)^2 \]
\[ = x^4 + 4 x^2 - 4 x^3 \]
\[f'\left( x \right) = 4 x^3 + 8x - 12 x^2 \]
\[ = 4x \left( x^2 - 3x + 2 \right)\]
\[ = 4x \left( x - 1 \right)\left( x - 2 \right)\]
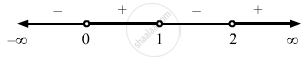
\[\text { Here, 0, 1 and 2 are the critical points}.\]
\[\text { The possible intervals are }\left( - \infty , 0 \right),\left( 0, 1 \right),\left( 1, 2 \right)\text { and }\left( 2, \infty \right).\]
\[\text { For f(x) to be increasing, we must have }\]
\[f'\left( x \right) > 0\]
\[ \Rightarrow 4x \left( x - 1 \right)\left( x - 2 \right) > 0\]
\[ \Rightarrow \left( x - 1 \right)\left( x - 2 \right) > 0\]
\[ \Rightarrow x \in \left( 0, 1 \right) \cup \left( 2, \infty \right) \]
\[\text { So,f(x)is increasing on x } \in \left( 0, 1 \right) \cup \left( 2, \infty \right) . \]
\[\text { For } f(x)\text { to be decreasing, we must have } \]
\[f'(x) < 0\]
\[ \Rightarrow 4x\left( x - 1 \right)\left( x - 2 \right) < 0\]
\[ \Rightarrow x\left( x - 1 \right)\left( x - 2 \right) < 0\]
\[ \Rightarrow x \in \left( - \infty , 0 \right) \cup \left( 1, 2 \right)\]
\[\text { So, f(x) is decreasing on x } \in \left( - \infty , 0 \right) \cup \left( 1, 2 \right) .\]

APPEARS IN
संबंधित प्रश्न
Find the intervals in which f(x) = sin 3x – cos 3x, 0 < x < π, is strictly increasing or strictly decreasing.
The side of an equilateral triangle is increasing at the rate of 2 cm/s. At what rate is its area increasing when the side of the triangle is 20 cm ?
Prove that the function f(x) = loge x is increasing on (0, ∞) ?
Find the interval in which the following function are increasing or decreasing f(x) = 2x3 − 24x + 7 ?
Find the interval in which the following function are increasing or decreasing \[f\left( x \right) = 3 x^4 - 4 x^3 - 12 x^2 + 5\] ?
Find the interval in which the following function are increasing or decreasing \[f\left( x \right) = \frac{3}{2} x^4 - 4 x^3 - 45 x^2 + 51\] ?
Find the intervals in which f(x) = sin x − cos x, where 0 < x < 2π is increasing or decreasing ?
Show that f(x) = e1/x, x ≠ 0 is a decreasing function for all x ≠ 0 ?
Show that f(x) = cos x is a decreasing function on (0, π), increasing in (−π, 0) and neither increasing nor decreasing in (−π, π) ?
Show that the function x2 − x + 1 is neither increasing nor decreasing on (0, 1) ?
Prove that the function f(x) = x3 − 6x2 + 12x − 18 is increasing on R ?
Prove that the function f given by f(x) = x3 − 3x2 + 4x is strictly increasing on R ?
Prove that the function f(x) = cos x is:
(i) strictly decreasing in (0, π)
(ii) strictly increasing in (π, 2π)
(iii) neither increasing nor decreasing in (0, 2π).
Show that f(x) = x + cos x − a is an increasing function on R for all values of a ?
What are the values of 'a' for which f(x) = ax is decreasing on R ?
Write the set of values of a for which f(x) = cos x + a2 x + b is strictly increasing on R ?
If the function f(x) = 2x2 − kx + 5 is increasing on [1, 2], then k lies in the interval
Let f(x) = x3 + ax2 + bx + 5 sin2x be an increasing function on the set R. Then, a and b satisfy.
The function \[f\left( x \right) = \log_e \left( x^3 + \sqrt{x^6 + 1} \right)\] is of the following types:
In the interval (1, 2), function f(x) = 2 | x − 1 | + 3 | x − 2 | is
The function \[f\left( x \right) = \frac{x}{1 + \left| x \right|}\] is
Let ϕ(x) = f(x) + f(2a − x) and f"(x) > 0 for all x ∈ [0, a]. Then, ϕ (x)
Find the values of x for which the following func- tions are strictly increasing : f(x) = x3 – 6x2 – 36x + 7
Prove that y = `(4sinθ)/(2 + cosθ) - θ` is an increasing function if `θ ∈[0, pi/2]`
Solve the following : Find the intervals on which the function y = xx, (x > 0) is increasing and decreasing.
Solve the following:
Find the intervals on which the function f(x) = `x/logx` is increasing and decreasing.
For manufacturing x units, labour cost is 150 – 54x and processing cost is x2. Price of each unit is p = 10800 – 4x2. Find the values of x for which Revenue is increasing.
Test whether the following function f(x) = 2 – 3x + 3x2 – x3, x ∈ R is increasing or decreasing
By completing the following activity, find the values of x such that f(x) = 2x3 – 15x2 – 84x – 7 is decreasing function.
Solution: f(x) = 2x3 – 15x2 – 84x – 7
∴ f'(x) = `square`
∴ f'(x) = 6`(square) (square)`
Since f(x) is decreasing function.
∴ f'(x) < 0
Case 1: `(square)` > 0 and (x + 2) < 0
∴ x ∈ `square`
Case 2: `(square)` < 0 and (x + 2) > 0
∴ x ∈ `square`
∴ f(x) is decreasing function if and only if x ∈ `square`
A circular pIate is contracting at the uniform rate of 5cm/sec. The rate at which the perimeter is decreasing when the radius of the circle is 10 cm Jong is
A man of height 1.9 m walks directly away from a lamp of height 4.75m on a level road at 6m/s. The rate at which the length of his shadow is increasing is
If f(x) = [x], where [x] is the greatest integer not greater than x, then f'(1') = ______.
Let f(x) = x3 + 9x2 + 33x + 13, then f(x) is ______.
The function f (x) = 2 – 3 x is ____________.
The interval in which `y = x^2e^(-x)` is increasing with respect to `x` is
Let 'a' be a real number such that the function f(x) = ax2 + 6x – 15, x ∈ R is increasing in `(-∞, 3/4)` and decreasing in `(3/4, ∞)`. Then the function g(x) = ax2 – 6x + 15, x∈R has a ______.
If f(x) = x5 – 20x3 + 240x, then f(x) satisfies ______.
The function f(x) = x3 + 3x is increasing in interval ______.
