Advertisements
Advertisements
प्रश्न
If sin A = `3/5` then show that 4 tan A + 3 sin A = 6 cos A
उत्तर
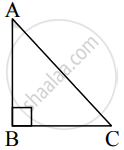
sin A = `3/5` ...(i) [Given]
In ∆ABC,
Let ∠ABC = 90°
∴ sin A = `"BC"/"AC"` .....(ii) [By definition]
∴ `"BC"/"AC" = 3/5` ......[From (i) and (ii)]
Let BC = 3k, AC = 5k
In ∆ABC, ∠B = 90°
∴ AB2 + BC2 = AC2 ......[Pythagoras theorem]
∴ AB2 + (3k)2 = (5k)2
∴ AB2 + 9k2 = 25k2
∴ AB2 = 25k2 – 9k2
∴ AB2 = 16k2
∴ AB = 4k ......[Taking square root of both sides]
Now, tan A = `"BC"/"AB"` ......[By definition]
∴ tan A = `(3"k")/(4"k") = 3/4`
cos A = `"AB"/"AC"` ......[By definition]
∴ cos A = `(4"k")/(5"k") = 4/5`
∴ 4 tan A + 3 sin A = `4(3/4) + 3(3/5)`
= `3 + 9/5`
=`(15 + 9)/5`
= `24/5` ......(iii)
6cos A = `6(4/5) = 24/5` ......(iv)
∴ 4 tan A + 3 sin A = 6 cos A .....[From (iii) and (iv)]
APPEARS IN
संबंधित प्रश्न
If tan 2θ = cot (θ + 6º), where 2θ and θ + 6º are acute angles, find the value of θ
Write all the other trigonometric ratios of ∠A in terms of sec A.
Prove the following trigonometric identities.
`((1 + cot^2 theta) tan theta)/sec^2 theta = cot theta`
solve.
cos240° + cos250°
solve.
sec2 18° - cot2 72°
Evaluate.
`(2tan53^@)/(cot37^@)-cot80^@/tan10^@`
A triangle ABC is right angles at B; find the value of`(secA.cosecC - tanA.cotC)/sinB`
Find the value of x, if sin 2x = 2 sin 45° cos 45°
Find the value of angle A, where 0° ≤ A ≤ 90°.
sin (90° – 3A) . cosec 42° = 1
Find the value of angle A, where 0° ≤ A ≤ 90°.
cos (90° – A) . sec 77° = 1
Evaluate:
`(sin35^circ cos55^circ + cos35^circ sin55^circ)/(cosec^2 10^circ - tan^2 80^circ)`
Use tables to find the acute angle θ, if the value of tan θ is 0.4741
Evaluate:
`(cos75^@)/(sin15^@) + (sin12^@)/(cos78^@) - (cos18^@)/(sin72^@)`
Prove that:
sin (28° + A) = cos (62° – A)
If 0° < A < 90°; find A, if `sinA/(secA - 1) + sinA/(secA + 1) = 2`
Write the maximum and minimum values of cos θ.
If 3 cot θ = 4, find the value of \[\frac{4 \cos \theta - \sin \theta}{2 \cos \theta + \sin \theta}\]
If θ is an acute angle such that \[\tan^2 \theta = \frac{8}{7}\] then the value of \[\frac{\left( 1 + \sin \theta \right) \left( 1 - \sin \theta \right)}{\left( 1 + \cos \theta \right) \left( 1 - \cos \theta \right)}\]
Prove that `"tan A"/"cot A" = (sec^2"A")/("cosec"^2"A")`
In ∆ABC, cos C = `12/13` and BC = 24, then AC = ?
