Advertisements
Advertisements
प्रश्न
There are two poles, one each on either bank of a river just opposite to each other. One pole is 60 m high. From the top of this pole, the angle of depression of the top and foot of the other pole are 30° and 60° respectively. Find the width of the river and height of the other pole.
उत्तर
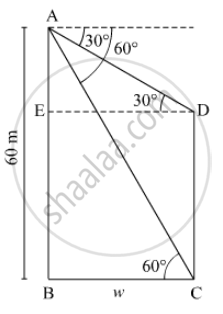
Let the width of the river be w.
In ΔABC,
tan 60° = `"AB"/"BC"`
⇒ `sqrt3` = `60/w`
⇒ w = `60/(sqrt3) = (60sqrt3)/3= 20sqrt3`
In △AED,
tan30° = `"AE"/"ED"`
⇒ `1/(sqrt3) = "AE"/w`
⇒ `1/(sqrt3) = "AE"/(20sqrt3)`
⇒ AE = 20
Height of pole CD = AB − AE
= 60 − 20 = 40 m.
Thus, width of river is `20sqrt3` = 20 x 1.732 = 34.64 m
Height of pole = 40 m.
संबंधित प्रश्न
(1 + tan θ + sec θ) (1 + cot θ − cosec θ) = ______.
Prove the following trigonometric identities
cosec6θ = cot6θ + 3 cot2θ cosec2θ + 1
Prove the following trigonometric identities. `(1 - cos A)/(1 + cos A) = (cot A - cosec A)^2`
Prove the following trigonometric identities.
`(1 - tan^2 A)/(cot^2 A -1) = tan^2 A`
Prove the following identities:
`(1 + sinA)/cosA + cosA/(1 + sinA) = 2secA`
Prove the following identities:
`sqrt((1 + sinA)/(1 - sinA)) = sec A + tan A`
Write the value of `(1 - cos^2 theta ) cosec^2 theta`.
Write the value of `4 tan^2 theta - 4/ cos^2 theta`
\[\frac{\sin \theta}{1 + \cos \theta}\]is equal to
Prove the following identity :
`1/(tanA + cotA) = sinAcosA`
Prove the following identity :
`sec^4A - sec^2A = sin^2A/cos^4A`
Prove the following identity :
`(cot^2θ(secθ - 1))/((1 + sinθ)) = sec^2θ((1-sinθ)/(1 + secθ))`
If x = acosθ , y = bcotθ , prove that `a^2/x^2 - b^2/y^2 = 1.`
Prove that `sqrt((1 - sin θ)/(1 + sin θ)) = sec θ - tan θ`.
Prove the following identities.
`sqrt((1 + sin theta)/(1 - sin theta)` = sec θ + tan θ
Prove that `cot^2 "A" [(sec "A" - 1)/(1 + sin "A")] + sec^2 "A" [(sin"A" - 1)/(1 + sec"A")]` = 0
Prove that `(sin^2theta)/(cos theta) + cos theta` = sec θ
Prove the following:
(sin α + cos α)(tan α + cot α) = sec α + cosec α
If cot θ = `40/9`, find the values of cosec θ and sinθ,
We have, 1 + cot2θ = cosec2θ
1 + `square` = cosec2θ
1 + `square` = cosec2θ
`(square + square)/square` = cosec2θ
`square/square` = cosec2θ ......[Taking root on the both side]
cosec θ = `41/9`
and sin θ = `1/("cosec" θ)`
sin θ = `1/square`
∴ sin θ = `9/41`
The value is cosec θ = `41/9`, and sin θ = `9/41`
Proved that `(1 + secA)/secA = (sin^2A)/(1 - cos A)`.
