Advertisements
Advertisements
Question
The area of the region (in square units) bounded by the curve x2 = 4y, line x = 2 and x-axis is
Options
1
2/3
4/3
8/3
Solution
2/3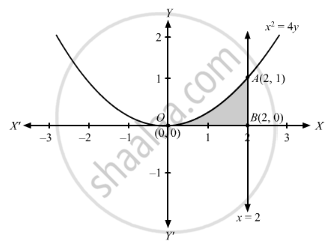
Point of intersection of the parabola x2 = 4y and straight line x = 2 is given by
\[x^2 = 4y\text{ and }x = 2\]
\[ \Rightarrow 4 = 4y\]
\[ \Rightarrow y = 1\]
\[A\left( 2, 1 \right)\text{ is the point of intersection of the curve and straight line }\]
\[\text{ Area of shaded region OAB }= \int_0^2 y dx\]
\[ = \int_0^2 \frac{x^2}{4} dx \]
\[ = \left[ \frac{x^3}{12} \right]_0^2 \]
\[ = \frac{2^3}{12} - 0\]
\[ = \frac{2}{3}\text{ square units }\]
APPEARS IN
RELATED QUESTIONS
Find the area of the region bounded by the parabola y2 = 16x and the line x = 3.
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5.
Draw a rough sketch of the graph of the curve \[\frac{x^2}{4} + \frac{y^2}{9} = 1\] and evaluate the area of the region under the curve and above the x-axis.
Find the area of the region bounded by the curve xy − 3x − 2y − 10 = 0, x-axis and the lines x = 3, x = 4.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Find the area bounded by the curve y = cos x, x-axis and the ordinates x = 0 and x = 2π.
Find the area of the region bounded by x2 = 16y, y = 1, y = 4 and the y-axis in the first quadrant.
Find the area of the region bounded by x2 + 16y = 0 and its latusrectum.
Find the area of the region included between the parabola y2 = x and the line x + y = 2.
Draw a rough sketch of the region {(x, y) : y2 ≤ 5x, 5x2 + 5y2 ≤ 36} and find the area enclosed by the region using method of integration.
Draw a rough sketch and find the area of the region bounded by the two parabolas y2 = 4x and x2 = 4y by using methods of integration.
Find the area of the region bounded by \[y = \sqrt{x}, x = 2y + 3\] in the first quadrant and x-axis.
Find the area common to the circle x2 + y2 = 16 a2 and the parabola y2 = 6 ax.
OR
Find the area of the region {(x, y) : y2 ≤ 6ax} and {(x, y) : x2 + y2 ≤ 16a2}.
Prove that the area common to the two parabolas y = 2x2 and y = x2 + 4 is \[\frac{32}{3}\] sq. units.
Find the area enclosed by the curve \[y = - x^2\] and the straight line x + y + 2 = 0.
Using the method of integration, find the area of the region bounded by the following lines:
3x − y − 3 = 0, 2x + y − 12 = 0, x − 2y − 1 = 0.
Find the area of the region bounded by y = | x − 1 | and y = 1.
Find the area bounded by the lines y = 4x + 5, y = 5 − x and 4y = x + 5.
Using integration, find the area of the following region: \[\left\{ \left( x, y \right) : \frac{x^2}{9} + \frac{y^2}{4} \leq 1 \leq \frac{x}{3} + \frac{y}{2} \right\}\]
Using integration find the area of the region bounded by the curves \[y = \sqrt{4 - x^2}, x^2 + y^2 - 4x = 0\] and the x-axis.
Find the area enclosed by the curves y = | x − 1 | and y = −| x − 1 | + 1.
The area bounded by y = 2 − x2 and x + y = 0 is _________ .
The area bounded by the y-axis, y = cos x and y = sin x when 0 ≤ x ≤ \[\frac{\pi}{2}\] is _________ .
Area lying in first quadrant and bounded by the circle x2 + y2 = 4 and the lines x = 0 and x = 2, is
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
Find the area of the region above the x-axis, included between the parabola y2 = ax and the circle x2 + y2 = 2ax.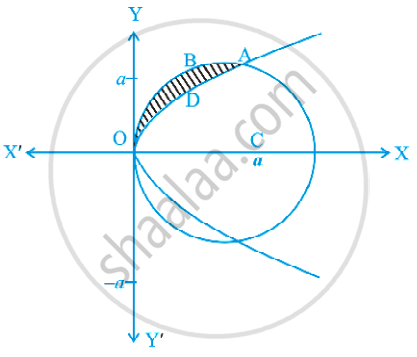
The area of the region bounded by the y-axis, y = cosx and y = sinx, 0 ≤ x ≤ `pi/2` is ______.
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
Using integration, find the area of the region bounded between the line x = 4 and the parabola y2 = 16x.
Area of the region bounded by the curve `y^2 = 4x`, `y`-axis and the line `y` = 3 is:
What is the area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3.
Find the area of the region bounded by the curve `y = x^2 + 2, y = x, x = 0` and `x = 3`
Find the area of the region enclosed by the curves y2 = x, x = `1/4`, y = 0 and x = 1, using integration.
Area of figure bounded by straight lines x = 0, x = 2 and the curves y = 2x, y = 2x – x2 is ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
Let P(x) be a real polynomial of degree 3 which vanishes at x = –3. Let P(x) have local minima at x = 1, local maxima at x = –1 and `int_-1^1 P(x)dx` = 18, then the sum of all the coefficients of the polynomial P(x) is equal to ______.
Sketch the region bounded by the lines 2x + y = 8, y = 2, y = 4 and the Y-axis. Hence, obtain its area using integration.
