Advertisements
Advertisements
प्रश्न
\[\frac{dy}{dx} - y \tan x = e^x\]
उत्तर
We have,
\[\frac{dy}{dx} - y \tan x = e^x \]
\[\text{Comparing with }\frac{dy}{dx} + Py = Q,\text{ we get}\]
\[P = - \tan x \]
\[Q = e^x \]
Now,
\[I . F . = e^{\int - \tan x\ dx} \]
\[ = e^{- \log\left| \left( \sec x \right) \right|} \]
\[ = e^{\log\left| \left( \cos x \right) \right|} \]
\[ = \cos x\]
So, the solution is given by
\[y \cos x = \int e^x \cos x dx + C\]
\[ \Rightarrow y \cos\ x = I + C . . . . . . . . . . . \left( 1 \right)\]
Where,
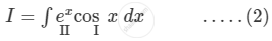
\[ \Rightarrow I = \cos x\int e^x dx - \int\left[ \frac{d}{dx}\left( \cos x \right)\int e^x dx \right]dx\]
\[ \Rightarrow I = \cos x e^x + \int\sin x e^x dx\]
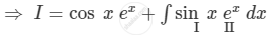
\[ \Rightarrow I = \cos x e^x + \sin x\int e^x dx - \int\left[ \frac{d}{dx}\left( \sin x \right)\int e^x dx \right]dx\]
\[ \Rightarrow I = \cos x e^x + \sin x e^x - \int\cos x e^x dx\]
\[ \Rightarrow I = \cos x e^x + \sin x e^x - I ............\left[\text{From (2)}\right]\]
\[ \Rightarrow 2I = \cos x e^x + \sin x e^x \]
\[ \Rightarrow I = \frac{e^x}{2}\left( \cos x + \sin x \right)\]
\[ \therefore y \cos x = \frac{e^x}{2}\left( \cos x + \sin x \right) + C .............\left[\text{From (1)} \right]\]
APPEARS IN
संबंधित प्रश्न
If x = Φ(t) differentiable function of ‘ t ' then prove that `int f(x) dx=intf[phi(t)]phi'(t)dt`
Find the particular solution of the differential equation `e^xsqrt(1-y^2)dx+y/xdy=0` , given that y=1 when x=0
Form the differential equation of the family of circles in the second quadrant and touching the coordinate axes.
If y = P eax + Q ebx, show that
`(d^y)/(dx^2)=(a+b)dy/dx+aby=0`
Find the particular solution of the differential equation x (1 + y2) dx – y (1 + x2) dy = 0, given that y = 1 when x = 0.
Verify that the given function (explicit or implicit) is a solution of the corresponding differential equation:
xy = log y + C : `y' = (y^2)/(1 - xy) (xy != 1)`
Verify that the given function (explicit or implicit) is a solution of the corresponding differential equation:
y – cos y = x : (y sin y + cos y + x) y′ = y
The number of arbitrary constants in the particular solution of a differential equation of third order are ______.
Find the general solution of the differential equation `dy/dx + sqrt((1-y^2)/(1-x^2)) = 0.`
Find the particular solution of the differential equation
`tan x * (dy)/(dx) = 2x tan x + x^2 - y`; `(tan x != 0)` given that y = 0 when `x = pi/2`
Write the order of the differential equation associated with the primitive y = C1 + C2 ex + C3 e−2x + C4, where C1, C2, C3, C4 are arbitrary constants.
The general solution of the differential equation \[\frac{dy}{dx} = \frac{y}{x}\] is
The number of arbitrary constants in the general solution of differential equation of fourth order is
The general solution of the differential equation \[\frac{y dx - x dy}{y} = 0\], is
The general solution of a differential equation of the type \[\frac{dx}{dy} + P_1 x = Q_1\] is
Find the general solution of the differential equation \[x \cos \left( \frac{y}{x} \right)\frac{dy}{dx} = y \cos\left( \frac{y}{x} \right) + x .\]
cos (x + y) dy = dx
(1 + y + x2 y) dx + (x + x3) dy = 0
(x2 + 1) dy + (2y − 1) dx = 0
(x3 − 2y3) dx + 3x2 y dy = 0
\[\frac{dy}{dx} + y = 4x\]
\[x\frac{dy}{dx} + x \cos^2 \left( \frac{y}{x} \right) = y\]
`(dy)/(dx)+ y tan x = x^n cos x, n ne− 1`
For the following differential equation, find the general solution:- \[\frac{dy}{dx} = \sqrt{4 - y^2}, - 2 < y < 2\]
Solve the following differential equation:-
\[\frac{dy}{dx} - y = \cos x\]
Solve the differential equation dy = cosx(2 – y cosecx) dx given that y = 2 when x = `pi/2`
Solve: `y + "d"/("d"x) (xy) = x(sinx + logx)`
Find the general solution of `("d"y)/("d"x) -3y = sin2x`
Integrating factor of the differential equation `cosx ("d"y)/("d"x) + ysinx` = 1 is ______.
Integrating factor of `(x"d"y)/("d"x) - y = x^4 - 3x` is ______.
Solution of `("d"y)/("d"x) - y` = 1, y(0) = 1 is given by ______.
The solution of `("d"y)/("d"x) + y = "e"^-x`, y(0) = 0 is ______.
Integrating factor of the differential equation `("d"y)/("d"x) + y tanx - secx` = 0 is ______.
The general solution of `("d"y)/("d"x) = 2x"e"^(x^2 - y)` is ______.
Find the particular solution of the differential equation `x (dy)/(dx) - y = x^2.e^x`, given y(1) = 0.
