Advertisements
Advertisements
Question
ABC is an isosceles right-angled triangle. Assuming of AB = BC = x, find the value of each of the following trigonometric ratio: cos 45°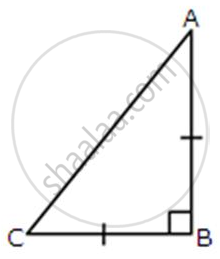
Solution
Given that AB = BC = x
∴ AC = `sqrt(AB^2+BC^2) = sqrt(x^2 + x^2) = xsqrt2`
cos 45° =`"BC"/"AC" = x/(xsqrt2) = 1/sqrt2`
APPEARS IN
RELATED QUESTIONS
Find the value of θ in each of the following :
(i) 2 sin 2θ = √3 (ii) 2 cos 3θ = 1
If tan (A + B) = `sqrt3` and tan (A – B) = `1/sqrt3`; 0° < A + B ≤ 90°; A > B, find A and B.
Evaluate the following in the simplest form: sin 60º cos 45º + cos 60º sin 45º
Express each one of the following in terms of trigonometric ratios of angles lying between 0° and 45°
tan 65° + cot 49°
Express each one of the following in terms of trigonometric ratios of angles lying between 0° and 45°
cot 85° + cos 75°
Prove that sin 48° sec 42° + cos 48° cosec 42° = 2
Prove the following :
`(cos(90°−A) sin(90°−A))/tan(90°−A) - sin^2 A = 0`
Evaluate: Cosec (65 + θ) – sec (25 – θ) – tan (55 – θ) + cot (35 + θ)
Evaluate: tan 7° tan 23° tan 60° tan 67° tan 83°
Evaluate: `(2sin 68)/cos 22 - (2 cot 15^@)/(5 tan 75^@) - (8 tan 45^@ tan 20^@ tan 40^@ tan 50^@ tan 70^@)/5`
Without using trigonometric tables, prove that:
cos54° cos36° − sin54° sin36° = 0
Express each of the following in terms of trigonometric ratios of angles lying between 0° and 45°.
sin67° + cos75°
If A =30o, then prove that :
sin 2A = 2sin A cos A = `(2 tan"A")/(1 + tan^2"A")`
If A = B = 45° ,
show that:
sin (A - B) = sin A cos B - cos A sin B
If tan (A + B) = 1 and tan(A-B)`=1/sqrt3` , 0° < A + B < 90°, A > B, then find the values of A and B.
prove that:
cos (2 x 30°) = `(1 – tan^2 30°)/(1+tan^2 30°)`
secθ . Cot θ= cosecθ ; write true or false
If A =30o, then prove that :
sin 3A = 3 sin A - 4 sin3A.
If A = 30°;
show that:
`(1 – cos 2"A")/(sin 2"A") = tan"A"`
If A = 30°;
show that:
`(1 + sin 2"A" + cos 2"A")/(sin "A" + cos"A") = 2 cos "A"`
Without using tables, evaluate the following: sec30° cosec60° + cos60° sin30°.
Without using tables, evaluate the following: tan230° + tan260° + tan245°
Without using tables, find the value of the following: `(4)/(cot^2 30°) + (1)/(sin^2 60°) - cos^2 45°`
Find the value of x in the following: cos2x = cos60° cos30° + sin60° sin30°
If sinθ = cosθ and 0° < θ<90°, find the value of 'θ'.
Verify the following equalities:
sin 30° cos 60° + cos 30° sin 60° = sin 90°
Find the value of the following:
sin2 30° – 2 cos3 60° + 3 tan4 45°
Verify cos3A = 4cos3A – 3cosA, when A = 30°
If 2 sin 2θ = `sqrt(3)` then the value of θ is
