Advertisements
Advertisements
प्रश्न
उत्तर
We have,
\[\frac{dy}{dx} = x \log x\]
\[ \Rightarrow dy = \left( x \log x \right)dx\]
Integrating both sides, we get
\[\int dy = \int\left( x \log x \right)dx\]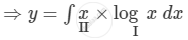
\[ \Rightarrow y = \log x\int x\ dx - \int\left[ \frac{d}{dx}\left( \log x \right)\int x\ dx \right]dx\]
\[ \Rightarrow y = \log x \times \frac{x^2}{2} - \int\left( \frac{1}{x} \times \frac{x^2}{2} \right)dx\]
\[ \Rightarrow y = \frac{1}{2} x^2 \log x - \int\frac{x}{2}dx\]
\[ \Rightarrow y = \frac{1}{2} x^2 \log x - \frac{x^2}{4} + C\]
\[ \text{ Hence, }y = \frac{1}{2} x^2 \log x - \frac{x^2}{4} + \text{C is the solution to the given differential equation.}\]
APPEARS IN
संबंधित प्रश्न
Show that Ax2 + By2 = 1 is a solution of the differential equation x \[\left\{ y\frac{d^2 y}{d x^2} + \left( \frac{dy}{dx} \right)^2 \right\} = y\frac{dy}{dx}\]
For the following differential equation verify that the accompanying function is a solution:
| Differential equation | Function |
|
\[x\frac{dy}{dx} + y = y^2\]
|
\[y = \frac{a}{x + a}\]
|
Differential equation \[\frac{d^2 y}{d x^2} + y = 0, y \left( 0 \right) = 0, y' \left( 0 \right) = 1\] Function y = sin x
Differential equation \[\frac{d^2 y}{d x^2} - 3\frac{dy}{dx} + 2y = 0, y \left( 0 \right) = 1, y' \left( 0 \right) = 3\] Function y = ex + e2x
(1 + x2) dy = xy dx
Solve the following differential equation:
\[y e^\frac{x}{y} dx = \left( x e^\frac{x}{y} + y^2 \right)dy, y \neq 0\]
(x + y) (dx − dy) = dx + dy
Solve the following initial value problem:-
\[x\frac{dy}{dx} - y = \left( x + 1 \right) e^{- x} , y\left( 1 \right) = 0\]
The surface area of a balloon being inflated, changes at a rate proportional to time t. If initially its radius is 1 unit and after 3 seconds it is 2 units, find the radius after time t.
The population of a city increases at a rate proportional to the number of inhabitants present at any time t. If the population of the city was 200000 in 1990 and 250000 in 2000, what will be the population in 2010?
Find the equation of the curve passing through the point \[\left( 1, \frac{\pi}{4} \right)\] and tangent at any point of which makes an angle tan−1 \[\left( \frac{y}{x} - \cos^2 \frac{y}{x} \right)\] with x-axis.
Find the equation of the curve which passes through the point (3, −4) and has the slope \[\frac{2y}{x}\] at any point (x, y) on it.
The normal to a given curve at each point (x, y) on the curve passes through the point (3, 0). If the curve contains the point (3, 4), find its equation.
Radium decomposes at a rate proportional to the quantity of radium present. It is found that in 25 years, approximately 1.1% of a certain quantity of radium has decomposed. Determine approximately how long it will take for one-half of the original amount of radium to decompose?
The slope of a curve at each of its points is equal to the square of the abscissa of the point. Find the particular curve through the point (−1, 1).
The integrating factor of the differential equation (x log x)
\[\frac{dy}{dx} + y = 2 \log x\], is given by
Which of the following is the integrating factor of (x log x) \[\frac{dy}{dx} + y\] = 2 log x?
The integrating factor of the differential equation \[x\frac{dy}{dx} - y = 2 x^2\]
In the following example, verify that the given function is a solution of the corresponding differential equation.
| Solution | D.E. |
| y = xn | `x^2(d^2y)/dx^2 - n xx (xdy)/dx + ny =0` |
Solve the following differential equation.
`y^3 - dy/dx = x dy/dx`
For each of the following differential equations find the particular solution.
(x − y2 x) dx − (y + x2 y) dy = 0, when x = 2, y = 0
For each of the following differential equations find the particular solution.
`y (1 + logx)dx/dy - x log x = 0`,
when x=e, y = e2.
Solve the following differential equation.
`dy/dx + y = e ^-x`
Solve the following differential equation.
`dy/dx + y` = 3
Solve the following differential equation.
`(x + a) dy/dx = – y + a`
Solve the following differential equation.
dr + (2r)dθ= 8dθ
Choose the correct alternative.
The differential equation of y = `k_1 + k_2/x` is
The differential equation of `y = k_1e^x+ k_2 e^-x` is ______.
Choose the correct alternative.
Bacteria increases at the rate proportional to the number present. If the original number M doubles in 3 hours, then the number of bacteria will be 4M in
For the differential equation, find the particular solution (x – y2x) dx – (y + x2y) dy = 0 when x = 2, y = 0
Solve the following differential equation
`x^2 ("d"y)/("d"x)` = x2 + xy − y2
Verify y = `a + b/x` is solution of `x(d^2y)/(dx^2) + 2 (dy)/(dx)` = 0
y = `a + b/x`
`(dy)/(dx) = square`
`(d^2y)/(dx^2) = square`
Consider `x(d^2y)/(dx^2) + 2(dy)/(dx)`
= `x square + 2 square`
= `square`
Hence y = `a + b/x` is solution of `square`
