Advertisements
Advertisements
Question
Using integration, find the area of the region bounded by the triangle ABC whose vertices A, B, C are (−1, 1), (0, 5) and (3, 2) respectively.
Solution
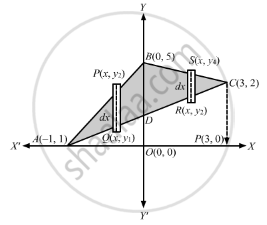
Equation of line AB is
\[y - 1 = \left( \frac{5 - 1}{0 + 1} \right)\left( x - \left( - 1 \right) \right)\]
\[ \Rightarrow y = 4x + 5 \]
Area under the line AB = area ABDO
\[ = \int_{- 1}^0 \left( 4x + 5 \right) dx\]
\[ = \left[ 4\frac{x^2}{2} + 5x \right]_{- 1}^0 \]
\[ = 0 - \left( 2 - 5 \right)\]
\[ \Rightarrow\text{ Area ABDO }= 3\text{ sq . units .} . . \left( 1 \right)\]
Equation of line BC is
\[y - 5 = \left( \frac{2 - 5}{3 - 0} \right)\left( x - 0 \right)\]
\[ \Rightarrow y = - x + 5\]
Area under line BC = Area OBCP
\[ = \int_0^3 \left( - x + 5 \right) dx\]
\[ = \left[ - \frac{x^2}{2} + 5x \right]_0^3 \]
\[ = - \frac{9}{2} + 15 - 0\]
\[ \Rightarrow\text{ Area OBCP }= \frac{21}{2}\text{ sq . units . }. . \left( 2 \right)\]
Equation of line CA is
\[y - 2 = \left( \frac{2 - 1}{3 - \left( - 1 \right)} \right)\left( x - 3 \right) \]
\[ \Rightarrow 4y = x + 5\]
\[ \therefore\text{ Area under line AC = Area ACPA }\]
\[ \Rightarrow A = \int_{- 1}^3 \left( \frac{x + 5}{4} \right)dx\]
\[ \Rightarrow A = \frac{1}{4} \left[ \frac{x}{2}^2 + 5x \right]_{- 1}^3 \]
\[ \Rightarrow A = \frac{1}{4}\left[ \frac{3}{2}^2 + 5 \times 3 - \frac{\left( - 1 \right)}{2}^2 + 5\left( - 1 \right) \right]\]
\[ \Rightarrow A = \frac{1}{4}\left[ \frac{9}{2} + 15 - \frac{1}{2} + 5 \right]\]
\[ \Rightarrow\text{ Area ACPA }= \frac{24}{4} = 6 \text{ sq . units }. . . \left( 3 \right)\]
\[\text{ From }\left( 1 \right), \left( 2 \right)\text{ and }\left( 3 \right)\]
\[\text{ Area }\Delta\text{ ABC }=\text{ Area ABDO + Area OBCP - Area ACPA }\]
\[ \Rightarrow A = 3 + \frac{21}{2} - 6\]
\[ \Rightarrow A = \frac{21}{2} - 3 = \frac{21 - 6}{2} = \frac{15}{2} \text{ sq . units }\]
\[ \therefore\text{ Area }\Delta\text{ ABC }= \frac{15}{2}\text{ sq . units }\]
APPEARS IN
RELATED QUESTIONS
Prove that the curves y2 = 4x and x2 = 4y divide the area of square bounded by x = 0, x = 4, y = 4 and y = 0 into three equal parts.
Find the area of the region lying in the first quandrant bounded by the curve y2= 4x, X axis and the lines x = 1, x = 4
Using integration, find the area of the region bounded by the line y − 1 = x, the x − axis and the ordinates x= −2 and x = 3.
Draw a rough sketch of the curve y = \[\frac{\pi}{2} + 2 \sin^2 x\] and find the area between x-axis, the curve and the ordinates x = 0, x = π.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Compare the areas under the curves y = cos2 x and y = sin2 x between x = 0 and x = π.
Find the area of the region bounded by x2 + 16y = 0 and its latusrectum.
Calculate the area of the region bounded by the parabolas y2 = x and x2 = y.
Find the area of the region \[\left\{ \left( x, y \right): \frac{x^2}{a^2} + \frac{y^2}{b^2} \leq 1 \leq \frac{x}{a} + \frac{y}{b} \right\}\]
Find the area of the region bounded by the curves y = x − 1 and (y − 1)2 = 4 (x + 1).
Find the area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2= 32.
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2.
Make a sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 3; 0 ≤ y ≤ 2x + 3; 0 ≤ x ≤ 3} and find its area using integration.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
The area included between the parabolas y2 = 4x and x2 = 4y is (in square units)
The area bounded by the parabola x = 4 − y2 and y-axis, in square units, is ____________ .
The area of the region \[\left\{ \left( x, y \right) : x^2 + y^2 \leq 1 \leq x + y \right\}\] is __________ .
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
Using the method of integration, find the area of the region bounded by the lines 3x − 2y + 1 = 0, 2x + 3y − 21 = 0 and x − 5y + 9 = 0
Using integration, find the area of the smaller region bounded by the ellipse `"x"^2/9+"y"^2/4=1`and the line `"x"/3+"y"/2=1.`
The area enclosed by the ellipse `x^2/"a"^2 + y^2/"b"^2` = 1 is equal to ______.
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
Find the area bounded by the curve y = sinx between x = 0 and x = 2π.
Draw a rough sketch of the region {(x, y) : y2 ≤ 6ax and x 2 + y2 ≤ 16a2}. Also find the area of the region sketched using method of integration.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
The area of the region bounded by the curve x2 = 4y and the straight line x = 4y – 2 is ______.
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
The area of the region bounded by the ellipse `x^2/25 + y^2/16` = 1 is ______.
Find the area of the region bounded by `y^2 = 9x, x = 2, x = 4` and the `x`-axis in the first quadrant.
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.
Using integration, find the area of the region bounded by the curves x2 + y2 = 4, x = `sqrt(3)`y and x-axis lying in the first quadrant.
Area (in sq.units) of the region outside `|x|/2 + |y|/3` = 1 and inside the ellipse `x^2/4 + y^2/9` = 1 is ______.
Find the area of the smaller region bounded by the curves `x^2/25 + y^2/16` = 1 and `x/5 + y/4` = 1, using integration.
Make a rough sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 1, 0 ≤ y ≤ x + 1, 0 ≤ x ≤ 2} and find the area of the region, using the method of integration.
Find the area of the region bounded by the curve x2 = 4y and the line x = 4y – 2.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
