Advertisements
Advertisements
Question
If the area enclosed by the parabolas y2 = 16ax and x2 = 16ay, a > 0 is \[\frac{1024}{3}\] square units, find the value of a.
Solution
The parabola y2 = 16ax opens towards the positive x-axis and its focus is (4a, 0).
The parabola x2 = 16ay opens towards the positive y-axis and its focus is (0, 4a).
Solving y2 = 16ax and x2 = 16ay, we get
\[\left( \frac{x^2}{16a} \right)^2 = 16ax\]
\[ \Rightarrow x^4 = \left( 16a \right)^3 x\]
\[ \Rightarrow x^4 - \left( 16a \right)^3 x = 0\]
\[ \Rightarrow x\left[ x^3 - \left( 16a \right)^3 \right] = 0\]
\[ \Rightarrow x = 0\text{ or }x = 16a\]
So, the points of intersection of the given parabolas are O(0, 0) and A(16a, 16a).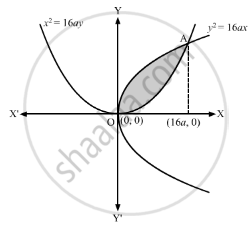
Area enclosed by the given parabolas
= Area of the shaded region
\[= \int_0^{16a} \sqrt{16ax}dx - \int_0^{16a} \frac{x^2}{16a}dx\]
\[ = \left.4\sqrt{a} \times \frac{x^\frac{3}{2}}{\frac{3}{2}}\right|_0^{16a} - \left.\frac{1}{16a} \times \frac{x^3}{3}\right|_0^{16a} \]
\[ = \frac{8\sqrt{a}}{3}\left[ \left( 16a \right)^\frac{3}{2} - 0 \right] - \frac{1}{48a}\left[ \left( 16a \right)^3 - 0 \right]\]
\[ = \frac{8\sqrt{a}}{3} \times 64a\sqrt{a} - \frac{256 a^2}{3}\]
\[ = \frac{512 a^2}{3} - \frac{256 a^2}{3}\]
\[ = \frac{256 a^2}{3}\text{ square units }\]
But,
Area enclosed by the given parabolas = \[\frac{1024}{3}\] square units ..........(Given)
\[\therefore \frac{256 a^2}{3} = \frac{1024}{3}\]
\[ \Rightarrow a^2 = \frac{1024}{256} = 4\]
\[ \Rightarrow a = 2 ............\left( a > 0 \right)\]
Thus, the value of a is 2.
APPEARS IN
RELATED QUESTIONS
Find the area bounded by the curve y2 = 4ax, x-axis and the lines x = 0 and x = a.
Using the method of integration, find the area of the triangular region whose vertices are (2, -2), (4, 3) and (1, 2).
Find the area of ellipse `x^2/1 + y^2/4 = 1`
Draw a rough sketch of the graph of the curve \[\frac{x^2}{4} + \frac{y^2}{9} = 1\] and evaluate the area of the region under the curve and above the x-axis.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Compare the areas under the curves y = cos2 x and y = sin2 x between x = 0 and x = π.
Find the area of the region {(x, y) : y2 ≤ 8x, x2 + y2 ≤ 9}.
Find the area of the region between the circles x2 + y2 = 4 and (x − 2)2 + y2 = 4.
Find the area of the region bounded by \[y = \sqrt{x}, x = 2y + 3\] in the first quadrant and x-axis.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Using integration, find the area of the region bounded by the triangle whose vertices are (−1, 2), (1, 5) and (3, 4).
Find the area of the region in the first quadrant enclosed by x-axis, the line y = \[\sqrt{3}x\] and the circle x2 + y2 = 16.
Find the area bounded by the curves x = y2 and x = 3 − 2y2.
Make a sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 3; 0 ≤ y ≤ 2x + 3; 0 ≤ x ≤ 3} and find its area using integration.
Find the area enclosed by the curves y = | x − 1 | and y = −| x − 1 | + 1.
If the area bounded by the parabola \[y^2 = 4ax\] and the line y = mx is \[\frac{a^2}{12}\] sq. units, then using integration, find the value of m.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
The area of the region formed by x2 + y2 − 6x − 4y + 12 ≤ 0, y ≤ x and x ≤ 5/2 is ______ .
The area of the region \[\left\{ \left( x, y \right) : x^2 + y^2 \leq 1 \leq x + y \right\}\] is __________ .
The area bounded by the parabola y2 = 8x, the x-axis and the latusrectum is ___________ .
Area of the region bounded by the curve y2 = 4x, y-axis and the line y = 3, is
Using integration, find the area of the region bounded by the line x – y + 2 = 0, the curve x = \[\sqrt{y}\] and y-axis.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using vertical strips.
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
Using integration, find the area of the smaller region bounded by the ellipse `"x"^2/9+"y"^2/4=1`and the line `"x"/3+"y"/2=1.`
Find the area of the region bounded by the parabola y2 = 2px, x2 = 2py
Find the area of region bounded by the line x = 2 and the parabola y2 = 8x
Sketch the region `{(x, 0) : y = sqrt(4 - x^2)}` and x-axis. Find the area of the region using integration.
Find the area of the region bounded by y = `sqrt(x)` and y = x.
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
The area of the region bounded by the curve y = x + 1 and the lines x = 2 and x = 3 is ______.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
The curve x = t2 + t + 1,y = t2 – t + 1 represents
Find the area of the region bounded by the curve `y^2 - x` and the line `x` = 1, `x` = 4 and the `x`-axis.
Find the area of the region bounded by `x^2 = 4y, y = 2, y = 4`, and the `y`-axis in the first quadrant.
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.
Smaller area bounded by the circle `x^2 + y^2 = 4` and the line `x + y = 2` is.
The area (in sq.units) of the region A = {(x, y) ∈ R × R/0 ≤ x ≤ 3, 0 ≤ y ≤ 4, y ≤x2 + 3x} is ______.
Hence find the area bounded by the curve, y = x |x| and the coordinates x = −1 and x = 1.
